We will discuss a lot about principal stress, principal stress example, Mohr’s circle and other related topics in this article. We will also discuss about finding principal stresses using Mohr’s circle.
When a single stress acts on a system we can easily make out that principal stress is the magnitude of the stress acting on the plane. When multiple stresses act on the system, then it gets difficult to assume the failure point of the material.
Hence, concept of principal stresses come into play, in this article we will discuss about principal stresses.
What are principal stresses?
Principal stresses are the value of the stresses acting normal to the plane where the shear stress is considered zero. This plane is oriented at an angle called as principal angle. Principal plane is the plane on which the principal stresses act.
1st principal stress, 2nd principal stress and 3rd principal stress are the three types of principal stresses generally used. We will discuss about these stresses in detail in further sections.
What is major principal stress?
The major principal is also called as 1st principal stress and it is the maximum tensile stress normal to the plane where the value of shear stress is zero. The plane on which this stress is acting is called as principal plane. It is an important fact that shear stress value is always zero in principal planes.
Mathematically, the major principal stress is given by following-
where the subscripts x and y represent stresses in x and y direction respectively.
What is minor principal stress?
The minor principal stress generally called as 3rd principal stress is the value of maximum compressive stress. This stress is also normal to the plane where the value of shear stress is zero.
There is another stress value which is intermediate in magnitude, it is called as 2nd principal stress. It is the minimum compressive stress acting in the system.
Mathematically, minor principal stress can be given by-
Maximum principal stress example
The formula for maximum principal stress or major principal stress is discussed in above sections.
Let us assume following data for stresses acting on a system. Using the following data we need to find maximum principal stress.
Given data:
Stress in x axis- 10 Mpa
Stress in y axis- 10 Mpa
Shear stress- 0 Mpa
Substituting the values in the formula of maximum principal stress we get maximum principal stress= 10 MPa
What is minimum principal stress?
Minimum principal stress or minor principal stress is the value of maximum compressive stress acting normal to the plane where shear stress is zero. This stress is the least of all the three principal stresses.
Mathematically, the minimum principal stress can be given as-
where x and y represent stresses in x and y directions respectively.
Minimum principal stress example
We have discussed formula for minimum principal stress in above sections. Let us assume the following data to find the minimum principal stress.
Given data:
Stress in x direction- 10 MPa
Stress in y direction- 10 MPa
Shear stress- 0 MPa
Substituting the values in the formula for minimum principal stress we get, minimum principal stress= 10 MPa
Mohr’s circle
Mohr’s circle is graphical representation of stresses and is used to identify the failure points of the material. It makes it convenient for engineers to get an idea of nature of stresses acting on the system and calculating failure points.
The image below shows Mohr’s circle for a 3D system of forces.
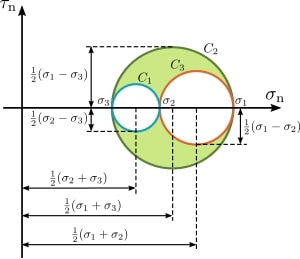
Image credits: Wikipedia
Mohr’s circle for two dimensional state of stress
The Mohr’s circle matrix for two dimensional state of stress can be given as-
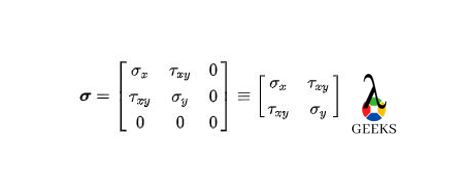
The name itself suggests that the stress acting in z direction is zero.
Equation of Mohr’s circle
Let us consider a two dimensional state of stress that is stress in z direction is zero. The Mohr’s circle equation for the assumed system of stresses can be written as-
As discussed in above sections, here also x and y represent stresses in x and y direction respectively. Theta represents principal angle.
Is principal stress same as Von Mises stress?
The principal stress is same as Von Mises stress for a single stress acting on the system. However, for more than one stress acting on the system the Von Mises stress and principal stress are different.
The principal stresses are real stresses acting on the plane whereas the Von Mises stress is a derived version of stress that tells us whether the material will yield or fracture under the given set of stresses.
Finding principal stresses from Mohr’s circle
The principal stresses can be found using the formula given below-
Maximum principal stress can be given by –
Minimum principal stress can be given using the formula given below-
R is the radius of Mohr’s circle.
The radius of Mohr’s circle represents maximum in-plane shear stress.
Stress matrix
The stress matrix or Cauchy stress tensor represents all the stresses acting on the system in a matrix form. This matrix represents stresses acting in all the three directions. The matrix is discussed in above sections.
The stress matrix is used to identify the stresses acting in a particular direction and is used to calculate the three main principal stresses.
Significance of principal stresses
The principal stresses are used to find yield stresses (such as Von Mises stress) which tells us whether the material will fail or yield under the given set of stresses. Principal stresses are used are in theories of failure.
Different theories of failure (such as Rankine, Tresca’s, Von Mises etc) use values of principal stresses to find whether the material will yield or fail with the given set of stresses.
Hi ….I am Abhishek Khambhata, have pursued B. Tech in Mechanical Engineering. Throughout four years of my engineering, I have designed and flown unmanned aerial vehicles. My forte is fluid mechanics and thermal engineering. My fourth-year project was based on the performance enhancement of unmanned aerial vehicles using solar technology. I would like to connect with like-minded people.