The Comprehensive Guide to Polar Moment of Inertia: A Detailed Exploration

The polar moment of inertia (J) is a crucial parameter in the analysis and design of mechanical systems, particularly in the context of torsional deformation and stress. This comprehensive guide delves into the intricacies of polar moment of inertia, providing a detailed exploration of its definition, formulas, applications, and practical considerations for physics students and engineers.

Understanding Polar Moment of Inertia

The polar moment of inertia, also known as the torsional moment of inertia, is a measure of an object’s resistance to torsional or twisting deformation around a specified axis. It is calculated as the sum of the moments of inertia about two perpendicular axes lying in the plane of the cross-section that passes through the point of interest.

The formula for the polar moment of inertia is given by:

|J = ∫r^2 dA|

Where:
– J is the polar moment of inertia
– r is the distance from the axis of rotation to the infinitesimal area element dA

The units of the polar moment of inertia are length to the fourth power (L^4), with the SI unit being meters to the fourth power (m^4) and the imperial unit being inches to the fourth power (in^4).

Importance of Polar Moment of Inertia

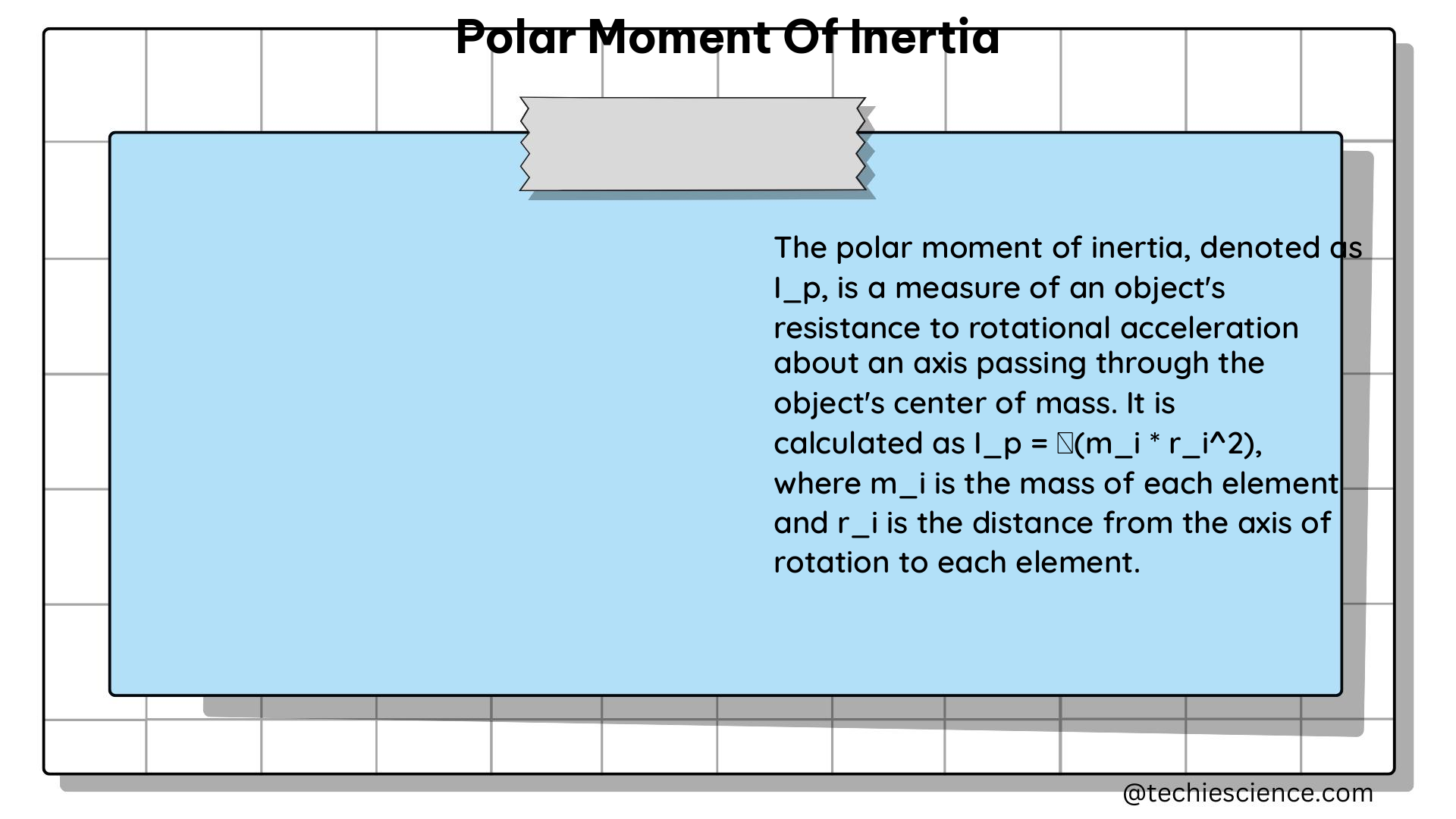
The polar moment of inertia is a crucial parameter in the design and analysis of mechanical systems, particularly in the context of drive shafts, torsional vibrations, and the calculation of torsional stresses and deformations.

1. Drive Shaft Design: The polar moment of inertia is a key factor in determining the resistance of a drive shaft to torsional deformation. Shafts with a larger polar moment of inertia will be more resistant to twisting, making them more suitable for applications that involve high torsional loads.

2. Torsional Vibration Analysis: The polar moment of inertia is used in the analysis of torsional vibrations in mechanical systems, such as those found in rotating machinery. It helps determine the natural frequencies and mode shapes of the system, which are crucial for understanding and mitigating torsional vibration issues.

3. Torsional Stress and Deformation Calculations: The polar moment of inertia is used in the calculation of torsional stresses and angular deformations in circular cross-section members subjected to torsional loads. This information is essential for the structural integrity and reliability of mechanical components.

Types of Cross-Section Polar Moment of Inertia

There are three main types of cross-section polar moment of inertia:

1. Hollow Cylinder Shaft:
2. Formula: |J = π(R1^4 – R0^4)/2|

3. Where:

   • R1 is the outer radius
   • R0 is the inner radius

4. Thin-Walled Shaft:

5. Formula: |J = 2πRt^3|

6. Where:

   • R is the radius
   • t is the thickness

7. Solid Cylinder Shaft:

8. Formula: |J = πR^4/2|
9. Where:
   • R is the radius

These formulas provide a convenient way to calculate the polar moment of inertia for different cross-sectional geometries, which is essential for the design and analysis of various mechanical components.

Polar Moment of Inertia vs. Moment of Inertia

It is important to distinguish between the polar moment of inertia and the moment of inertia, as they are related but distinct concepts.

The moment of inertia is a measure of an object’s resistance to angular acceleration around a specified axis. It is calculated as the sum of the products of the mass of each element of the object and the square of its distance from the axis.

The formula for the moment of inertia is:

|I = ∫r^2 dm|

Where:
– I is the moment of inertia
– r is the distance from the axis of rotation to the infinitesimal mass element dm

The units of the moment of inertia are mass times length squared (ML^2), with the SI unit being kilogram meters squared (kg m^2).

The key difference between the polar moment of inertia and the moment of inertia is that the polar moment of inertia is specifically related to torsional deformation, while the moment of inertia is a more general measure of an object’s resistance to angular acceleration.

Practical Applications of Polar Moment of Inertia

The polar moment of inertia has numerous practical applications in various engineering fields, including:

1. Shaft Design: The polar moment of inertia is a critical parameter in the design of drive shafts, as it determines the shaft’s resistance to torsional deformation and the resulting stresses.

2. Torsional Vibration Analysis: The polar moment of inertia is used in the analysis of torsional vibrations in rotating machinery, such as engines, turbines, and gearboxes, to ensure the system’s stability and prevent potential failures.

3. Structural Analysis: The polar moment of inertia is used in the analysis of torsional stresses and deformations in circular cross-section members, such as columns, beams, and shafts, subjected to torsional loads.

4. Fluid Mechanics: The polar moment of inertia is used in the analysis of the torsional behavior of fluid flow in circular pipes and ducts, which is important for the design of piping systems and fluid transport equipment.

5. Biomechanics: The polar moment of inertia is used in the analysis of the torsional behavior of biological structures, such as bones and joints, which is important for understanding and treating musculoskeletal injuries and disorders.

Numerical Examples and Problem-Solving

To further illustrate the application of polar moment of inertia, let’s consider a few numerical examples:

Example 1: Hollow Cylinder Shaft
Suppose we have a hollow cylinder shaft with an outer radius of 50 mm and an inner radius of 40 mm. Calculate the polar moment of inertia of the shaft.

Given:
– Outer radius (R1) = 50 mm = 0.05 m
– Inner radius (R0) = 40 mm = 0.04 m

Using the formula for a hollow cylinder shaft:
|J = π(R1^4 – R0^4)/2|
|J = π(0.05^4 – 0.04^4)/2|
|J = π(0.000625 – 0.000256)/2|
|J = π(0.000369)/2|
|J = 2.89 × 10^-6 m^4|

Example 2: Thin-Walled Shaft
Consider a thin-walled shaft with a radius of 75 mm and a thickness of 5 mm. Calculate the polar moment of inertia of the shaft.

Given:
– Radius (R) = 75 mm = 0.075 m
– Thickness (t) = 5 mm = 0.005 m

Using the formula for a thin-walled shaft:
|J = 2πRt^3|
|J = 2π(0.075)(0.005)^3|
|J = 2π(0.075)(0.000125)|
|J = 5.89 × 10^-7 m^4|

Example 3: Solid Cylinder Shaft
Suppose we have a solid cylinder shaft with a radius of 60 mm. Calculate the polar moment of inertia of the shaft.

Given:
– Radius (R) = 60 mm = 0.06 m

Using the formula for a solid cylinder shaft:
|J = πR^4/2|
|J = π(0.06)^4/2|
|J = π(0.001296)/2|
|J = 2.04 × 10^-6 m^4|

These examples demonstrate the application of the different formulas for calculating the polar moment of inertia for various cross-sectional geometries, which is essential for the design and analysis of mechanical systems.

Conclusion

The polar moment of inertia is a fundamental concept in the field of mechanics and engineering, with numerous practical applications in the design and analysis of mechanical systems. This comprehensive guide has provided a detailed exploration of the definition, formulas, and importance of the polar moment of inertia, as well as its distinction from the moment of inertia.

By understanding the principles and applications of the polar moment of inertia, physics students and engineers can enhance their ability to analyze and design mechanical components, ensuring the structural integrity and reliability of their systems.

References

1. Byju’s: Polar Moment of Inertia – https://byjus.com/jee/polar-moment-of-inertia/
2. Engineering Statics: Polar Moment of Inertia – https://engineeringstatics.org/polar-moment.html
3. ScienceDirect: Polar Moment of Inertia – https://www.sciencedirect.com/topics/engineering/polar-moment