The Parallelogram Law of Force: A Comprehensive Guide for Science Students

The parallelogram law of force is a fundamental concept in physics that allows for the addition of vectors, specifically forces, in a geometric manner. This law is based on the principle that if two forces acting on a body are represented by the two adjacent sides of a parallelogram drawn from a point, then the resultant force is represented completely by the diagonal of the parallelogram drawn from the same point.

Understanding the Parallelogram Law of Force

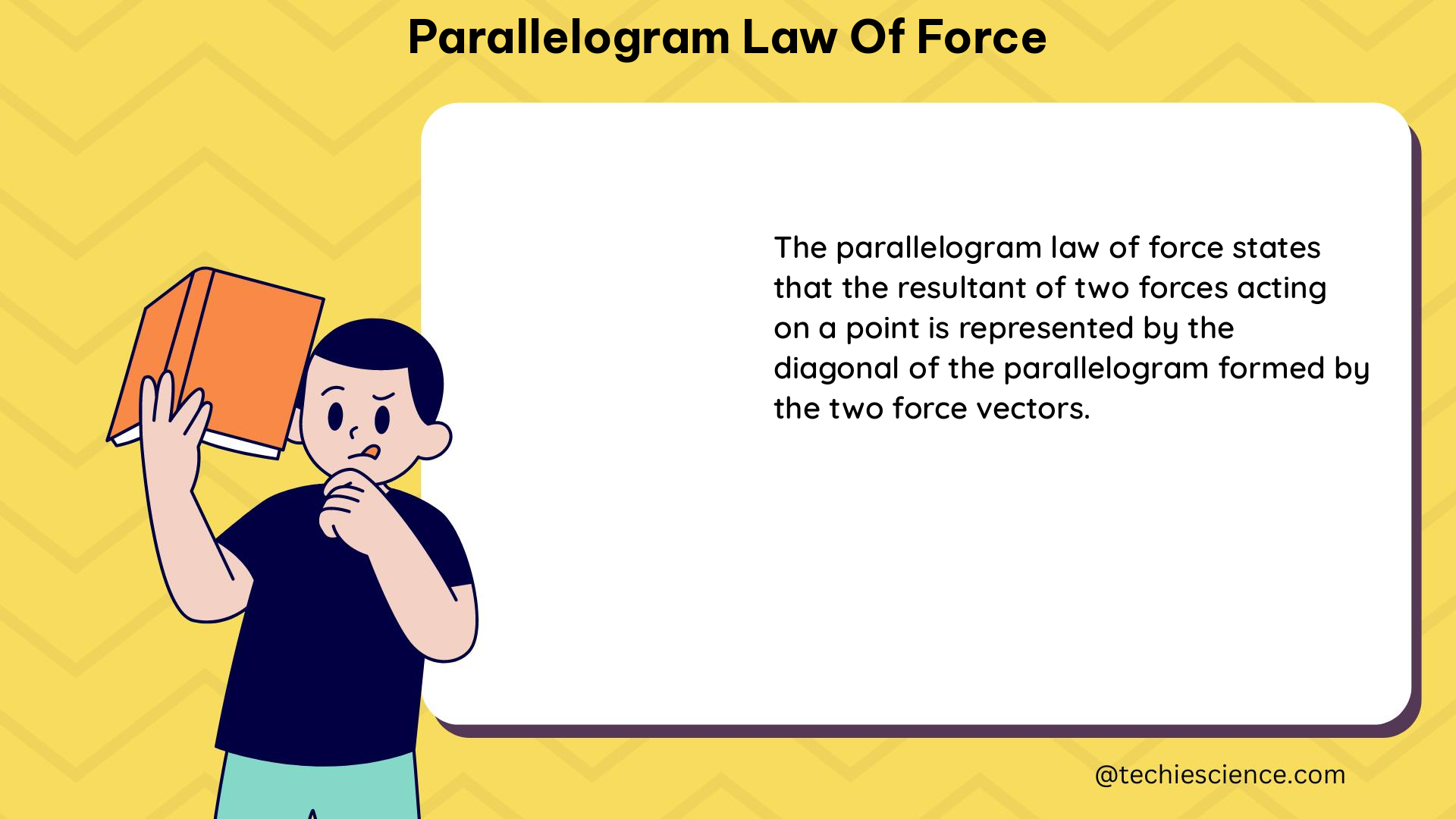
The parallelogram law of force is a theorem that states the following:

Theorem: If two forces, P and Q, are represented in magnitude and direction by the adjacent sides of a parallelogram, then the resultant force, R, is represented in magnitude and direction by the diagonal of the parallelogram.

Mathematically, the parallelogram law of force can be expressed as:

R = √(P^2 + Q^2 + 2PQcosθ)

Where:
– R is the magnitude of the resultant force
– P and Q are the magnitudes of the two forces
– θ is the angle between the two forces

The direction of the resultant force, β, can be calculated using the formula:

β = tan^-1[(Qsinθ)/(P + Qcosθ)]

Applying the Parallelogram Law of Force

Example 1: Calculating the Resultant Force

Consider two forces, P and Q, acting on a body with magnitudes of 4 N and 7 N, respectively, and an angle of 45° between them.

To calculate the magnitude of the resultant force, R, we can use the formula:

R = √(4^2 + 7^2 + 2×4×7cos45°)
R ≈ 12.008 N

To calculate the direction of the resultant force, β, we can use the formula:

β = tan^-1[(7sin45°)/(4 + 7cos45°)]
β ≈ 28.95°

Example 2: Applying the Parallelogram Law to Three Forces

The parallelogram law of force can also be used to calculate the resultant force of three or more forces. This involves applying the parallelogram law multiple times to add two forces at a time until all forces have been added together.

Consider three forces, P, Q, and R, acting on a body with magnitudes of 4 N, 7 N, and 5 N, respectively, and angles of 45° between P and Q, and 60° between Q and R.

Step 1: Calculate the resultant of forces P and Q using the parallelogram law.
R1 = √(4^2 + 7^2 + 2×4×7cos45°)
R1 ≈ 12.008 N
β1 = tan^-1[(7sin45°)/(4 + 7cos45°)]
β1 ≈ 28.95°

Step 2: Calculate the resultant of R1 and R using the parallelogram law.
R2 = √(12.008^2 + 5^2 + 2×12.008×5cos60°)
R2 ≈ 14.142 N
β2 = tan^-1[(5sin60°)/(12.008 + 5cos60°)]
β2 ≈ 45°

The final resultant force, R2, has a magnitude of approximately 14.142 N and a direction of 45°.

Graphical Representation of the Parallelogram Law of Force

The parallelogram law of force can be represented graphically using a parallelogram diagram. In this diagram, the two forces, P and Q, are represented by the adjacent sides of the parallelogram, and the resultant force, R, is represented by the diagonal of the parallelogram.

Applications of the Parallelogram Law of Force

The parallelogram law of force has a wide range of applications in various fields, including:

1. Engineering: Engineers can use the parallelogram law of force to calculate the resultant force acting on structures, such as buildings, bridges, and machines, due to various forces, such as wind, weight, and earthquakes. This information is crucial for designing safe and stable structures.

2. Sports: Coaches and athletes can use the parallelogram law of force to analyze the movements of athletes and improve their performance. For example, in soccer, the parallelogram law can be used to calculate the resultant force acting on a player during a kick, which can help optimize the player’s technique and increase their power and accuracy.

3. Physics Education: The parallelogram law of force is a fundamental concept in physics and is often used in the teaching and learning of vector addition, force analysis, and other related topics.

Conclusion

The parallelogram law of force is a powerful tool for understanding and analyzing the addition of vectors, specifically forces, in a geometric manner. By mastering the concepts and applications of this law, science students can develop a deeper understanding of physics and its real-world applications. This comprehensive guide has provided a detailed overview of the parallelogram law of force, including its theorem, mathematical formulas, examples, and graphical representation, as well as its diverse applications in various fields.

References

1. Parallelogram Law of Vectors
2. Parallelogram Law of Forces
3. Parallelogram Law of Forces Diagram
4. Understanding Parallelogram of Forces