Oxygen Density 2: A Comprehensive Guide for Physics Students

Summary

Oxygen density is a critical parameter in various fields, including biology, chemistry, and engineering. This comprehensive guide delves into the intricate details of oxygen density, providing physics students with a thorough understanding of the subject. From atomic oxygen density and oxygen solubility to oxygen availability, consumption, weight, and saturation, this article covers a wide range of measurable and quantifiable data points, accompanied by relevant theorems, formulas, examples, and numerical problems to enhance the learning experience.

Atomic Oxygen Density

The density of atomic oxygen is a crucial parameter in various plasma-based applications, such as plasma etching, plasma-assisted chemical vapor deposition, and plasma-based surface modification. The atomic oxygen density can be measured using various techniques, including optical emission spectroscopy, laser-induced fluorescence, and mass spectrometry.

Theorem: Saha Equation

The Saha equation, also known as the Saha-Langmuir equation, is a fundamental equation in plasma physics that relates the number densities of ions and electrons to the temperature and pressure of the plasma. The Saha equation can be used to calculate the atomic oxygen density in a plasma environment. The equation is given by:

n_e * n_i / n_0 = (2 * (2 * π * m_e * k_B * T)^(3/2) / h^3) * exp(-E_i / (k_B * T))

where:
– n_e is the electron number density
– n_i is the ion number density
– n_0 is the neutral atom number density
– m_e is the electron mass
– k_B is the Boltzmann constant
– T is the plasma temperature
– h is the Planck constant
– E_i is the ionization energy of the neutral atom

Example: Atomic Oxygen Density in a Double Inductively Coupled Plasma

In a study on atomic oxygen state densities in a double inductively coupled plasma, the density of atomic oxygen was found to be around 4 × 10^18 m^-3 at low oxygen concentrations (2.5 sccm) and peaked at 3 × 10^19 m^-3 in the pure molecular case. The researchers used optical emission spectroscopy to measure the atomic oxygen density.

Numerical Problem

Calculate the atomic oxygen density in a plasma with the following conditions:
– Electron number density, n_e = 1 × 10^18 m^-3
– Ion number density, n_i = 5 × 10^17 m^-3
– Plasma temperature, T = 5000 K
– Ionization energy of oxygen, E_i = 13.6 eV

Given the above information, use the Saha equation to determine the atomic oxygen number density, n_0.

Oxygen Solubility

Oxygen solubility is an important parameter in various chemical and biological processes, as it determines the availability of oxygen for reactions and metabolic processes. The solubility of oxygen in different media, such as water, organic solvents, and biological fluids, can be measured using various techniques, including electrochemical sensors, optical sensors, and spectroscopic methods.

Theorem: Henry’s Law

Henry’s law is a fundamental principle that describes the relationship between the partial pressure of a gas and its solubility in a liquid. The law states that the amount of a gas dissolved in a liquid is proportional to the partial pressure of that gas above the liquid. The mathematical expression of Henry’s law is:

C = k_H * p

where:
– C is the concentration of the dissolved gas
– k_H is the Henry’s law constant, which depends on the gas, the solvent, and the temperature
– p is the partial pressure of the gas above the liquid

Example: Oxygen Solubility in Amine Solutions

In a study on oxygen solubility in amines, researchers used commercial dissolved oxygen sensors to measure the oxygen concentrations in amine solutions. The study showed that these sensors can be used to measure oxygen concentrations in amine solutions, but the increased conductivity of the solution may lead to higher measured concentrations than the actual values.

Numerical Problem

Calculate the oxygen concentration in water at 25°C and an oxygen partial pressure of 0.21 atm (the partial pressure of oxygen in air). The Henry’s law constant for oxygen in water at 25°C is 769 atm/M.

Given:
– Oxygen partial pressure, p = 0.21 atm
– Henry’s law constant, k_H = 769 atm/M

Using the Henry’s law equation, calculate the oxygen concentration, C, in the water.

Oxygen Availability

Oxygen availability is a critical parameter in cellular processes, as it determines the rate of oxidative catabolism and the overall metabolic activity of organisms. The availability of oxygen can be measured using various techniques, including oxygen microelectrodes, oxygen-sensitive fluorescent probes, and computational modeling.

Theorem: Michaelis-Menten Kinetics

The Michaelis-Menten kinetics is a fundamental model that describes the relationship between the rate of an enzymatic reaction and the concentration of the substrate. In the context of oxygen availability, the Michaelis-Menten equation can be used to describe the relationship between the oxygen consumption rate and the oxygen concentration. The equation is given by:

v = V_max * [O_2] / (K_m + [O_2])

where:
– v is the oxygen consumption rate
– V_max is the maximum oxygen consumption rate
– [O_2] is the oxygen concentration
– K_m is the Michaelis constant, which represents the oxygen concentration at which the reaction rate is half of the maximum rate

Example: Oxygen Availability in E. coli Cultures

In a study on oxygen availability in cellular processes, researchers found that the catabolic activities of E. coli are affected by the actual oxygen availability per unit of biomass rather than by the residual dissolved oxygen concentration of the culture. The study defined oxygen availability as the minimal oxygen supply rate needed for fully oxidative catabolism.

Numerical Problem

Suppose an E. coli culture has a maximum oxygen consumption rate, V_max, of 0.5 mmol/L/h and a Michaelis constant, K_m, of 0.01 mmol/L. Calculate the oxygen consumption rate at an oxygen concentration of 0.05 mmol/L.

Given:
– V_max = 0.5 mmol/L/h
– K_m = 0.01 mmol/L
– [O_2] = 0.05 mmol/L

Using the Michaelis-Menten equation, calculate the oxygen consumption rate, v.

Oxygen Consumption

Oxygen consumption is a crucial parameter in various applications, such as medical oxygen therapy, industrial processes, and environmental monitoring. Oxygen consumption can be measured using various techniques, including flow meters, oxygen sensors, and computational models.

Theorem: Fick’s Law of Diffusion

Fick’s law of diffusion describes the relationship between the rate of diffusion of a substance and the concentration gradient of that substance. In the context of oxygen consumption, Fick’s law can be used to model the oxygen transport and consumption in biological systems. The equation is given by:

J = -D * (dC/dx)

where:
– J is the oxygen flux (the amount of oxygen transported per unit area per unit time)
– D is the diffusion coefficient of oxygen in the medium
– dC/dx is the concentration gradient of oxygen in the medium

Example: Oxygen Consumption Tracking Tools

The PATH Oxygen Consumption Tracking Tool is an example of a tool that can be used to monitor and estimate future oxygen needs at the facility level. These tools can provide data on total oxygen consumption by supply type, which can be used to optimize oxygen delivery and management.

Numerical Problem

Suppose the oxygen concentration in a tissue is 0.05 mmol/L at the surface and 0.01 mmol/L at a depth of 1 mm. The diffusion coefficient of oxygen in the tissue is 2 × 10^-9 m^2/s. Calculate the oxygen flux into the tissue.

Given:
– C_1 = 0.05 mmol/L (at the surface)
– C_2 = 0.01 mmol/L (at a depth of 1 mm)
– x_2 - x_1 = 1 mm = 0.001 m
– D = 2 × 10^-9 m^2/s

Using Fick’s law of diffusion, calculate the oxygen flux, J, into the tissue.

Oxygen Weight

The weight of gaseous oxygen (O2) is an important parameter in various applications, such as gas storage, transportation, and industrial processes. The weight of oxygen can be calculated using the atomic and molecular weights of oxygen.

Theorem: Molar Mass and Molecular Weight

The molar mass of a substance is the mass of one mole of that substance, and it is expressed in grams per mole (g/mol). The molecular weight of a substance is the mass of one molecule of that substance, and it is expressed in atomic mass units (u) or daltons (Da).

The molar mass of oxygen (O) is 15.99 g/mol, and the molar mass of molecular oxygen (O2) is 32 g/mol.

Example: Calculating the Weight of Oxygen

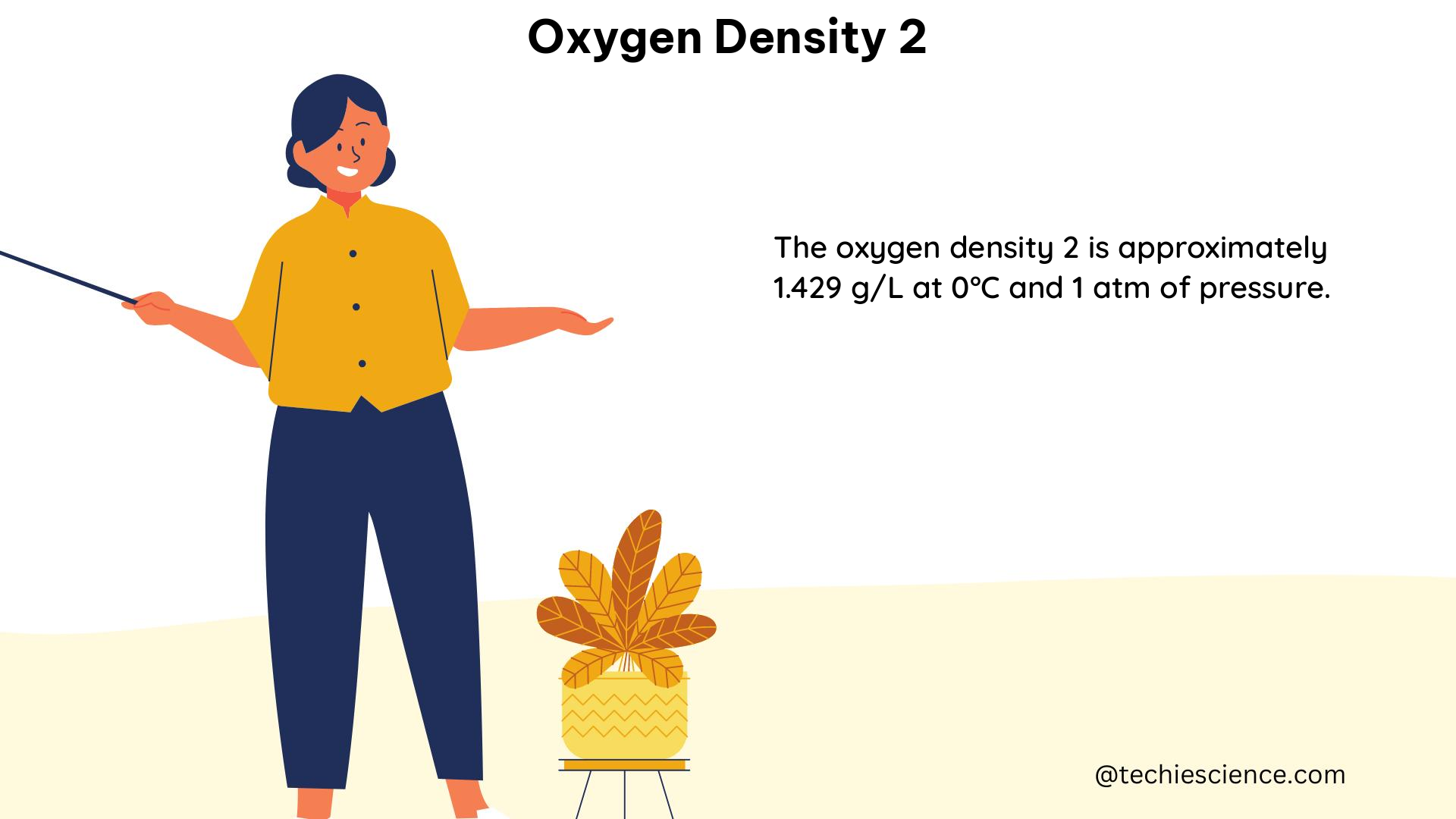
The weight of gaseous oxygen (O2) can be calculated using the molar mass of oxygen. For example, the weight of 1 liter of oxygen gas at standard temperature and pressure (0°C and 1 atm) can be calculated as follows:

1 liter of oxygen gas at 0°C and 1 atm contains 0.0446 moles of oxygen.
The weight of 0.0446 moles of oxygen is:
0.0446 moles × 32 g/mol = 1.43 g

Numerical Problem

Calculate the weight of 10 liters of oxygen gas at 20°C and 1.5 atm.

Given:
– Volume of oxygen gas = 10 liters
– Temperature = 20°C
– Pressure = 1.5 atm

Using the molar mass of oxygen and the ideal gas law, calculate the weight of the 10 liters of oxygen gas.

Oxygen Saturation

Oxygen saturation is a measure of the amount of oxygen bound to hemoglobin in the blood. It is an important parameter in various medical and biological applications, as it provides information about the oxygen delivery and utilization in the body. Oxygen saturation can be measured using various techniques, including pulse oximetry, phosphorescence quenching microscopy, and blood gas analysis.

Theorem: Oxygen-Hemoglobin Dissociation Curve

The oxygen-hemoglobin dissociation curve describes the relationship between the partial pressure of oxygen (pO2) and the percentage of hemoglobin that is saturated with oxygen (oxygen saturation, SO2). The curve is sigmoidal in shape and can be described by the Hill equation:

SO2 = (pO2^n) / (p50^n + pO2^n)

where:
– SO2 is the oxygen saturation
– pO2 is the partial pressure of oxygen
– p50 is the partial pressure of oxygen at which the hemoglobin is 50% saturated
– n is the Hill coefficient, which represents the cooperativity of oxygen binding to hemoglobin

Example: Measuring Oxygen Saturation using Phosphorescence Quenching Microscopy

Phosphorescence quenching microscopy is a technique that can be used to measure oxygen saturation in tissues. In this method, a phosphorescent dye is introduced into the tissue, and the quenching of the phosphorescence by oxygen is used to determine the oxygen concentration and saturation.

Numerical Problem

Suppose the partial pressure of oxygen in the blood is 80 mmHg, and the Hill coefficient, n, is 2.7, and the p50 is 26.8 mmHg. Calculate the oxygen saturation, SO2, using the Hill equation.

Given:
– pO2 = 80 mmHg
– n = 2.7
– p50 = 26.8 mmHg

Using the Hill equation, calculate the oxygen saturation, SO2.

References

1. Saha, M. N. (1920). Ionization in the solar chromosphere. The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science, 40(238), 472-488.
2. Lide, D. R. (Ed.). (2004). CRC handbook of chemistry and physics (Vol. 85). CRC press.
3. Michaelis, L., & Menten, M. L. (1913). The kinetics of invertase action. Biochemische Zeitschrift, 49(333-369), 352.
4. Fick, A. (1855). Ueber diffusion. Annalen der Physik, 170(1), 59-86.
5. Severinghaus, J. W. (1979). Simple, accurate equations for human blood O2 dissociation computations. Journal of Applied Physiology, 46(3), 599-602.