Numerical aperture (NA) is a crucial parameter in microscopy that determines the resolution, light-gathering ability, and depth of field of a microscope objective. This comprehensive guide will delve into the technical details of numerical aperture, providing physics students with a thorough understanding of this fundamental concept.
Understanding Numerical Aperture
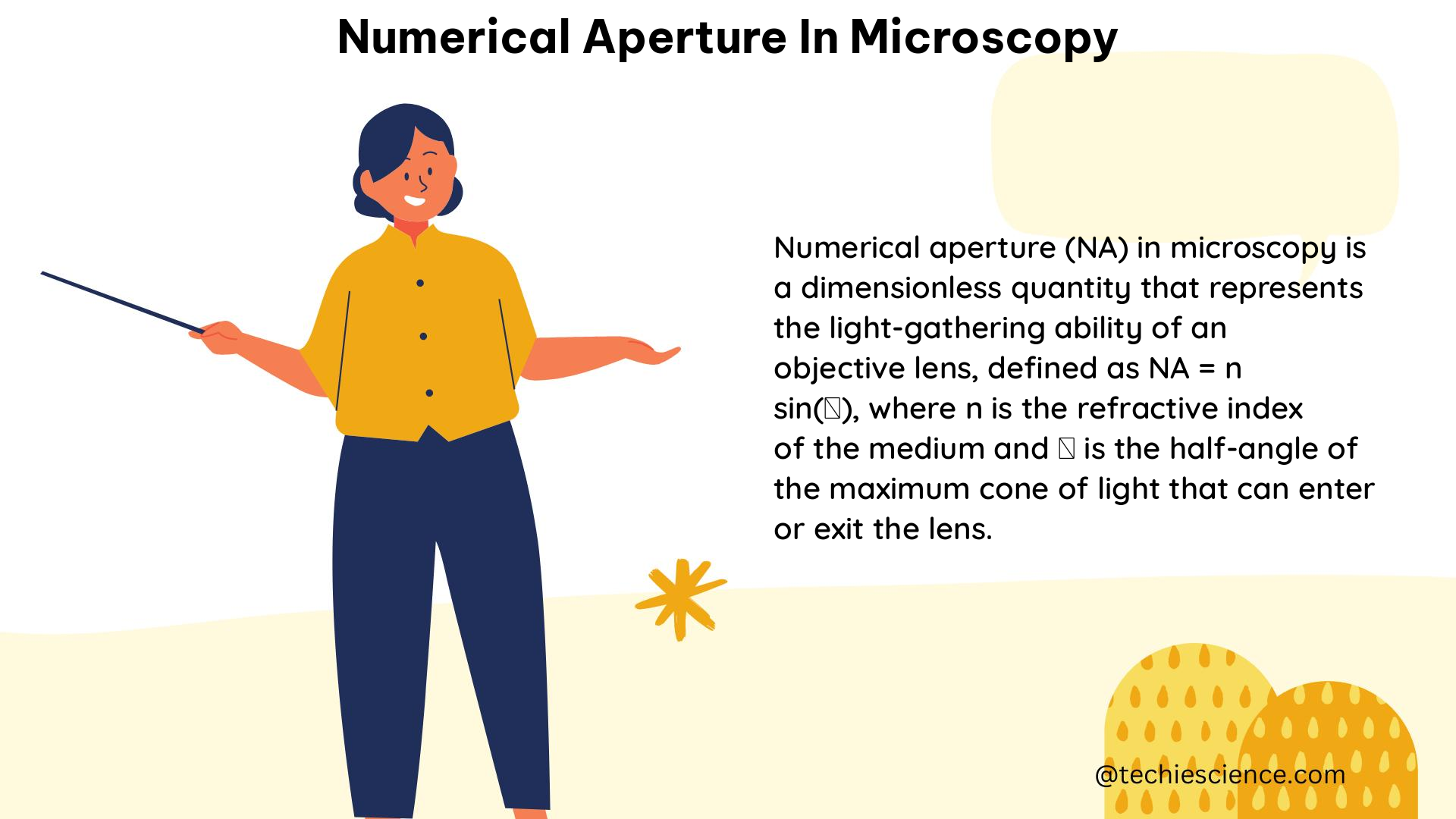
Numerical aperture is a dimensionless quantity that measures the light-gathering ability of a microscope objective. It is defined as the sine of half the angular aperture of the objective multiplied by the refractive index of the medium between the front lens of the objective and the cover glass. Mathematically, it is expressed as:
NA = n(sinα)
Where:
– n is the refractive index of the medium
– α is the angular aperture of the objective
The refractive index of the medium plays a crucial role in determining the numerical aperture. For example, in air (n = 1.0), the maximum theoretical numerical aperture for a dry objective is around 0.95, while in water (n = 1.33) or oil (n = 1.52), the maximum numerical aperture can be higher, reaching up to 1.4 or 1.6, respectively.
Relationship between Numerical Aperture and Resolution
The numerical aperture of a microscope objective is directly related to its ability to resolve fine specimen details. The higher the numerical aperture, the greater the objective’s ability to resolve fine details. This is because a higher numerical aperture allows increasingly oblique rays to enter the objective’s front lens, producing a more highly resolved image.
The relationship between numerical aperture and resolution is governed by the Abbe diffraction limit, which states that the minimum distance between two resolvable points (d) is given by:
d = λ / (2 × NA)
Where:
– d is the minimum distance between two resolvable points
– λ is the wavelength of the illuminating light
– NA is the numerical aperture of the objective
This formula demonstrates that the resolution of a microscope objective is directly proportional to its numerical aperture and inversely proportional to the wavelength of the illuminating light. For example, using a higher numerical aperture objective and shorter wavelength light (e.g., blue or ultraviolet) can significantly improve the resolution of a microscope.
Numerical Aperture and Depth of Field
In addition to resolution, the numerical aperture of a microscope objective also determines the depth of field, which is the distance over which the image remains in focus. A high numerical aperture objective has a shallow depth of field, while a low numerical aperture objective has a deeper depth of field.
The relationship between numerical aperture and depth of field can be expressed as:
Depth of field = λ / (2 × NA^2)
This formula shows that as the numerical aperture increases, the depth of field decreases. This is because a high numerical aperture objective collects light from a narrower angle, resulting in a narrower depth of field, while a low numerical aperture objective collects light from a wider angle, resulting in a deeper depth of field.
Practical Considerations for Numerical Aperture
In practice, the highest numerical aperture for a dry objective is around 0.90, as going beyond this value can lead to technical challenges, such as increased aberrations and reduced working distance. For immersion objectives, where the space between the objective and the cover glass is filled with a medium with a higher refractive index (e.g., water or oil), the numerical aperture can be significantly higher, reaching up to 1.4 or 1.6.
When selecting a microscope objective, it is essential to consider the trade-offs between numerical aperture, resolution, and depth of field. A higher numerical aperture objective will provide better resolution but a shallower depth of field, while a lower numerical aperture objective will have a deeper depth of field but lower resolution.
Numerical Aperture Calculations and Examples
To illustrate the concepts of numerical aperture, let’s consider a few examples:
- Dry Objective with NA = 0.65:
- Refractive index of air (n) = 1.0
- Angular aperture (α) = sin^-1(0.65) = 40.5°
- Numerical aperture (NA) = n × sin(α) = 1.0 × sin(40.5°) = 0.65
-
Minimum resolvable distance (d) = λ / (2 × NA) = 550 nm / (2 × 0.65) = 423 nm
-
Oil Immersion Objective with NA = 1.4:
- Refractive index of oil (n) = 1.52
- Angular aperture (α) = sin^-1(1.4/1.52) = 67.1°
- Numerical aperture (NA) = n × sin(α) = 1.52 × sin(67.1°) = 1.4
-
Minimum resolvable distance (d) = λ / (2 × NA) = 550 nm / (2 × 1.4) = 196 nm
-
Water Immersion Objective with NA = 1.2:
- Refractive index of water (n) = 1.33
- Angular aperture (α) = sin^-1(1.2/1.33) = 60.0°
- Numerical aperture (NA) = n × sin(α) = 1.33 × sin(60.0°) = 1.2
- Minimum resolvable distance (d) = λ / (2 × NA) = 550 nm / (2 × 1.2) = 229 nm
These examples demonstrate how the numerical aperture, angular aperture, and refractive index of the medium are related, and how they impact the resolution and depth of field of a microscope objective.
Conclusion
Numerical aperture is a fundamental concept in microscopy that determines the resolution, light-gathering ability, and depth of field of a microscope objective. By understanding the technical details and relationships between numerical aperture, resolution, and depth of field, physics students can make informed decisions when selecting and using microscope objectives for their research and experiments.
References:
- Microscope Resolution: Concepts, Factors and Calculation. Leica Microsystems. https://www.leica-microsystems.com/science-lab/life-science/microscope-resolution-concepts-factors-and-calculation/
- Numerical Aperture Light Cones. Nikon’s MicroscopyU. https://www.microscopyu.com/tutorials/nuaperture
- The effect of numerical aperture on quantitative use-wear studies. National Center for Biotechnology Information. https://www.ncbi.nlm.nih.gov/pmc/articles/PMC6474883/
- Numerical Aperture and Resolution. Navitar. https://navitar.com/navitar-blog/numerical-aperture-and-resolution/
- Microscopy Basics | Numerical Aperture and Resolution. Carl Zeiss Microscopy. https://zeiss-campus.magnet.fsu.edu/articles/basics/resolution.html

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.