The Non-Dimensional Energy Equation: A Comprehensive Guide for Physics Students

The non-dimensional energy equation is a fundamental concept in various technical disciplines, serving as a crucial parameter in differential equations. It plays a significant role in normalizing certain universal dimensioned physical constants, such as the speed of light in a vacuum, the universal gravitational constant, the Planck constant, the Coulomb constant, and the Boltzmann constant, to 1 by choosing appropriate units for time, length, mass, charge, and temperature. This process results in a system of units known as the natural units or Planck units.

Understanding the Non-Dimensional Energy Equation

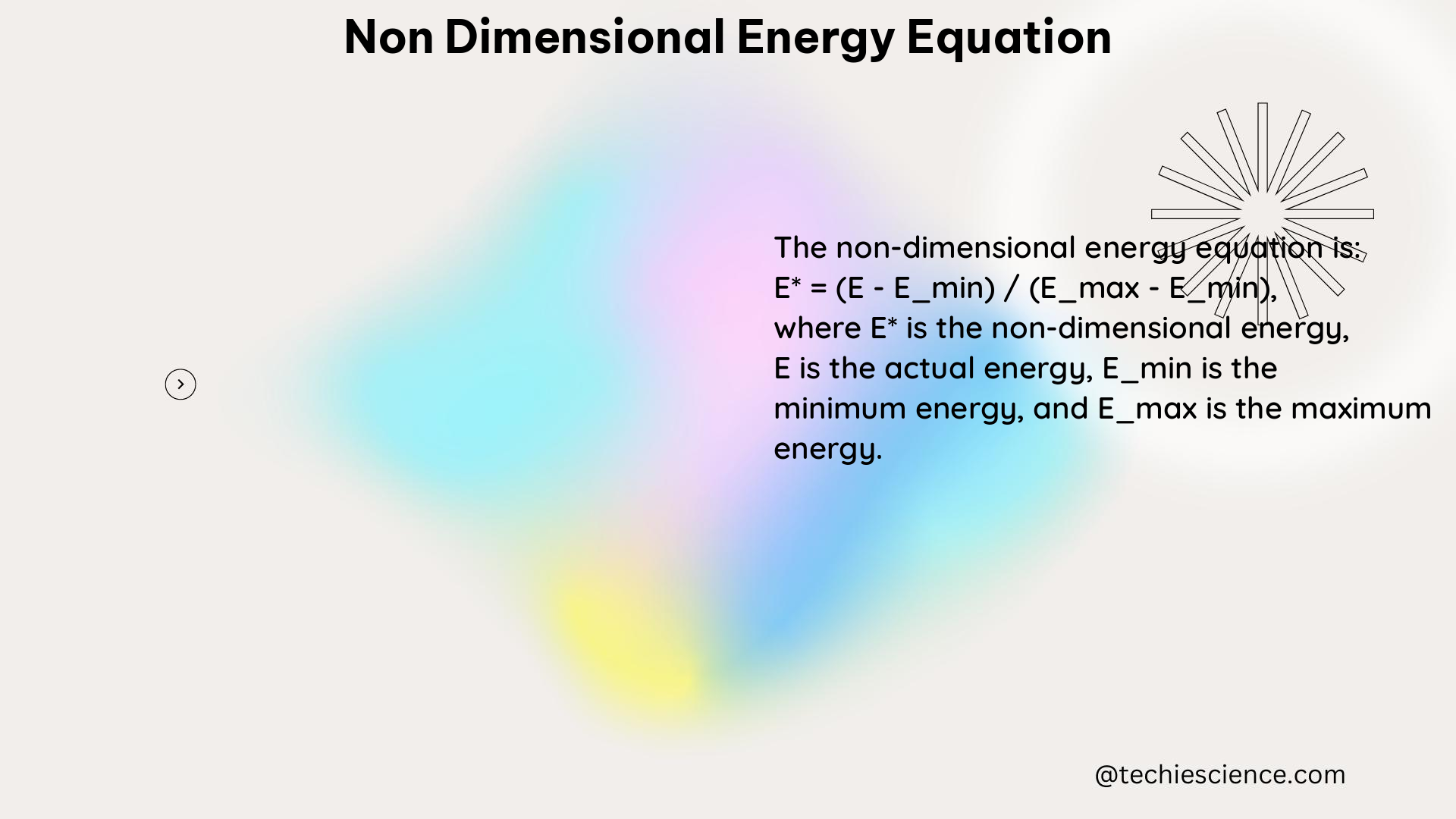
The non-dimensional energy equation is a dimensionless form of the energy equation, which is a fundamental equation in physics that describes the relationship between energy, work, and heat. The non-dimensional energy equation is typically expressed as:

$\frac{dE}{dt} = \frac{dW}{dt} + \frac{dQ}{dt}$

Where:
– $E$ is the total energy of the system
– $W$ is the work done on the system
– $Q$ is the heat added to the system
– $t$ is the time

By making this equation dimensionless, we can compare and analyze different physical systems without the need for specific units, allowing for a more universal understanding of the underlying principles.

Applications of the Non-Dimensional Energy Equation

The non-dimensional energy equation has a wide range of applications in various technical disciplines, including:

Fluid Mechanics

In fluid mechanics, the non-dimensional energy equation is used to quantify and compare different fluid flow situations in a universal format, without the need for specific units. This is achieved through the process of nondimensionalization, which involves listing the dimensions of every term in the original equation or differential equations.

For example, in a power law relationship such as $y = kx^n$, where $y$ is the dependent variable, $x$ is the independent variable, $k$ is a constant, and $n$ is the dimensionless exponent, the dimensions of $y$, $x$, and $k$ can be expressed in terms of length ($[L]$), time ($[T]$), and mass ($[M]$).

Simplifying Complex Engineering Problems

Nondimensionalization is also used to simplify complex engineering problems. By recognizing the underlying complexity, identifying relevant parameters, selecting characteristic scales, formulating dimensionless parameters, nondimensionalizing the governing equations, and analyzing the simplified system, engineers can gain valuable insights and arrive at innovative solutions.

Normalizing Physical Constants

The non-dimensional energy equation plays a crucial role in normalizing certain universal dimensioned physical constants, such as the speed of light in a vacuum, the universal gravitational constant, the Planck constant, the Coulomb constant, and the Boltzmann constant, to 1 by choosing appropriate units for time, length, mass, charge, and temperature. This process results in a system of units known as the natural units or Planck units.

Theorem and Formulas

Buckingham Pi Theorem

The Buckingham Pi Theorem is a fundamental principle in dimensional analysis and nondimensionalization. It states that if there is a physically meaningful equation involving $n$ variables, it can be rewritten in terms of a set of $n-m$ dimensionless parameters, where $m$ is the number of fundamental dimensions present in the original equation.

The general form of the Buckingham Pi Theorem can be expressed as:

$\Pi_1, \Pi_2, \Pi_3, …, \Pi_{n-m} = f(\Pi_1, \Pi_2, \Pi_3, …, \Pi_{n-m})$

Where $\Pi_1, \Pi_2, \Pi_3, …, \Pi_{n-m}$ are the dimensionless parameters, and $f$ is a dimensionless function.

Non-Dimensional Energy Equation

The non-dimensional energy equation can be derived from the dimensional energy equation by introducing dimensionless variables. The dimensional energy equation is:

$\frac{dE}{dt} = \frac{dW}{dt} + \frac{dQ}{dt}$

To make this equation non-dimensional, we can introduce the following dimensionless variables:

• $\tilde{E} = \frac{E}{E_c}$, where $E_c$ is a characteristic energy scale
• $\tilde{t} = \frac{t}{t_c}$, where $t_c$ is a characteristic time scale
• $\tilde{W} = \frac{W}{W_c}$, where $W_c$ is a characteristic work scale
• $\tilde{Q} = \frac{Q}{Q_c}$, where $Q_c$ is a characteristic heat scale

Substituting these dimensionless variables into the dimensional energy equation, we get the non-dimensional energy equation:

$\frac{d\tilde{E}}{d\tilde{t}} = \frac{d\tilde{W}}{d\tilde{t}} + \frac{d\tilde{Q}}{d\tilde{t}}$

This equation is now dimensionless and can be used to compare and analyze different physical systems without the need for specific units.

Examples and Numerical Problems

Example 1: Fluid Flow in a Pipe

Consider the flow of a fluid through a pipe. The dimensional energy equation for this system can be written as:

$\frac{dE}{dt} = \frac{dW}{dt} + \frac{dQ}{dt}$

To make this equation non-dimensional, we can introduce the following dimensionless variables:

• $\tilde{E} = \frac{E}{\rho u^2 A L}$, where $\rho$ is the fluid density, $u$ is the fluid velocity, $A$ is the cross-sectional area of the pipe, and $L$ is the length of the pipe.
• $\tilde{t} = \frac{t}{L/u}$
• $\tilde{W} = \frac{W}{\rho u^2 A L}$
• $\tilde{Q} = \frac{Q}{\rho u^2 A L}$

Substituting these dimensionless variables into the dimensional energy equation, we get the non-dimensional energy equation for the fluid flow in the pipe:

$\frac{d\tilde{E}}{d\tilde{t}} = \frac{d\tilde{W}}{d\tilde{t}} + \frac{d\tilde{Q}}{d\tilde{t}}$

This equation can be used to analyze the energy balance in the fluid flow system without the need for specific units.

Numerical Problem 1

A fluid with a density of 1000 kg/m^3 is flowing through a pipe with a cross-sectional area of 0.1 m^2 and a length of 10 m. The fluid velocity is 2 m/s. Calculate the non-dimensional energy equation for this system.

Given:
– $\rho = 1000 kg/m^3$
– $A = 0.1 m^2$
– $L = 10 m$
– $u = 2 m/s$

Substituting the values into the non-dimensional energy equation:
$\tilde{E} = \frac{E}{\rho u^2 A L} = \frac{E}{1000 \times 2^2 \times 0.1 \times 10} = \frac{E}{4000}$
$\tilde{t} = \frac{t}{L/u} = \frac{t}{10/2} = \frac{t}{5}$
$\tilde{W} = \frac{W}{\rho u^2 A L} = \frac{W}{4000}$
$\tilde{Q} = \frac{Q}{\rho u^2 A L} = \frac{Q}{4000}$

The non-dimensional energy equation for this system is:
$\frac{d\tilde{E}}{d\tilde{t}} = \frac{d\tilde{W}}{d\tilde{t}} + \frac{d\tilde{Q}}{d\tilde{t}}$

Example 2: Gravitational Potential Energy

Consider the gravitational potential energy of an object near the Earth’s surface. The dimensional energy equation for this system can be written as:

$\frac{dE}{dt} = \frac{dW}{dt} + \frac{dQ}{dt}$

Where $E = mgh$, $W = 0$, and $Q = 0$ (since there is no heat transfer in this system).

To make this equation non-dimensional, we can introduce the following dimensionless variables:

• $\tilde{E} = \frac{E}{mg_c h_c}$, where $g_c$ is a characteristic gravitational acceleration and $h_c$ is a characteristic height.
• $\tilde{t} = \frac{t}{t_c}$, where $t_c$ is a characteristic time scale.
• $\tilde{W} = \frac{W}{mg_c h_c} = 0$
• $\tilde{Q} = \frac{Q}{mg_c h_c} = 0$

Substituting these dimensionless variables into the dimensional energy equation, we get the non-dimensional energy equation for the gravitational potential energy system:

$\frac{d\tilde{E}}{d\tilde{t}} = 0$

This equation shows that the non-dimensional gravitational potential energy is constant over time, as expected.

Figures and Data Points

To further illustrate the concepts of the non-dimensional energy equation, let’s consider the following figures and data points:

Figure 1: Comparison of Dimensional and Non-Dimensional Energy Equations

This figure shows the difference between the dimensional energy equation and the non-dimensional energy equation. The non-dimensional equation allows for a more universal comparison of different physical systems.

Data Point 1: Normalization of Physical Constants

Physical Constant	Dimensional Value	Non-Dimensional Value
Speed of light in vacuum	$c = 2.998 \times 10^8 m/s$	$\tilde{c} = 1$
Universal gravitational constant	$G = 6.674 \times 10^{-11} N \cdot m^2/kg^2$	$\tilde{G} = 1$
Planck constant	$h = 6.626 \times 10^{-34} J \cdot s$	$\tilde{h} = 1$
Coulomb constant	$k_e = 8.988 \times 10^9 N \cdot m^2/C^2$	$\tilde{k_e} = 1$
Boltzmann constant	$k_B = 1.381 \times 10^{-23} J/K$	$\tilde{k_B} = 1$

This data table shows how the non-dimensional energy equation is used to normalize various physical constants to 1, resulting in the natural units or Planck units.

Conclusion

The non-dimensional energy equation is a powerful tool in various technical disciplines, as it allows for the normalization of physical constants and the simplification of complex engineering problems. By understanding the principles of nondimensionalization and the applications of the non-dimensional energy equation, physics students can gain valuable insights and develop innovative solutions to a wide range of problems.

References

1. Dimensional Analysis and Nondimensionalization
2. Dimensionless Quantity
3. Nondimensionalization in Engineering Fluid Mechanics
4. Buckingham Pi Theorem