Microscope Diffraction Limit Formula: A Comprehensive Guide

The diffraction limit is a fundamental principle in microscopy that describes the smallest feature size that can be resolved using an optical microscope. It is determined by the wavelength of light used to image the specimen and the numerical aperture (NA) of the objective lens. This comprehensive guide will delve into the intricacies of the microscope diffraction limit formula, providing you with a deep understanding of this crucial concept in the field of microscopy.

Understanding the Abbe Diffraction Limit Formula

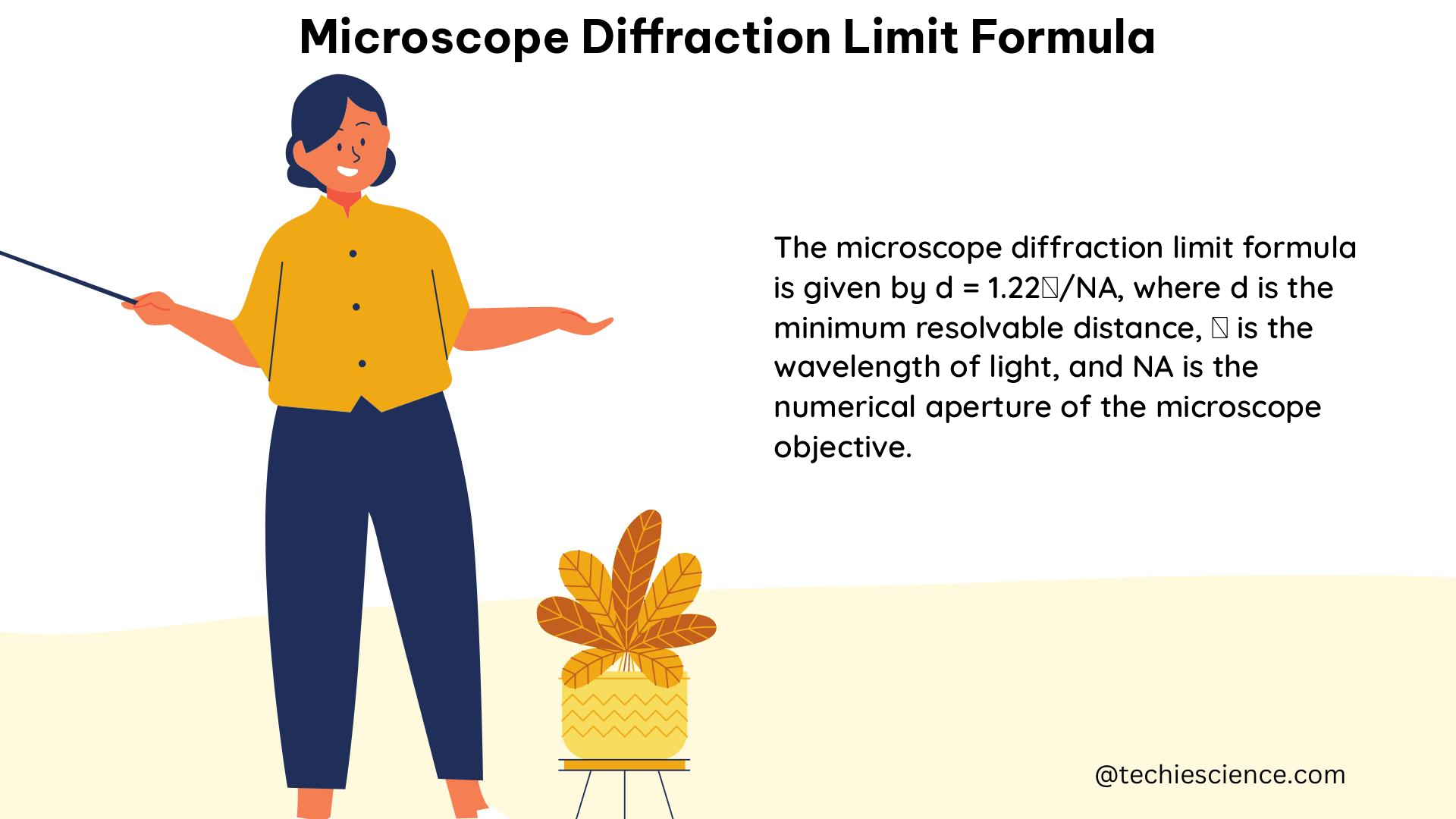
According to Abbe’s theory, the lateral resolution (d) of a microscope is given by the formula:

d = λ/(2NA)

where λ is the wavelength of light used to image the specimen, and NA is the numerical aperture of the objective lens.

Let’s explore this formula in more detail:

• Wavelength (λ): The wavelength of light used in the imaging process plays a crucial role in determining the resolution. Shorter wavelengths, such as those in the ultraviolet or blue-violet range, can achieve higher resolutions compared to longer wavelengths, such as those in the red or infrared range.

• Numerical Aperture (NA): The numerical aperture of the objective lens is a measure of the light-gathering ability of the lens. A higher NA value indicates a larger cone of light that can be collected by the lens, resulting in a higher resolution.

For example, if we use green light with a wavelength of 514 nm and an oil-immersion objective with an NA of 1.45, the theoretical limit of lateral resolution will be:

d = 514 nm / (2 × 1.45) = 177 nm

This means that the smallest feature that can be resolved using this microscope setup is approximately 177 nanometers.

Axial Resolution and the Abbe Diffraction Limit Formula

In addition to lateral resolution, the Abbe diffraction limit formula can also be used to calculate the axial resolution (d) of a microscope:

d = 2λ/(NA)^2

Here, the axial resolution is inversely proportional to the square of the numerical aperture (NA) and directly proportional to the wavelength (λ) of the light used.

Continuing the previous example, if we assume a wavelength of 514 nm and an objective with an NA of 1.45, the theoretical axial resolution will be:

d = 2 × 514 nm / (1.45)^2 = 488 nm

This means that the smallest feature that can be resolved in the axial direction is approximately 488 nanometers.

The Rayleigh Criterion and Microscope Resolution

The Rayleigh Criterion is a slightly refined formula based on Abbe’s diffraction limits. It takes into account the numerical aperture of both the objective lens and the condenser lens:

R = 1.22λ/(NA obj + NAcond)

where λ is the wavelength of light used to image the specimen, NAobj is the numerical aperture of the objective lens, and NAcond is the numerical aperture of the condenser lens.

Using the same example as before, with a green light of 514 nm, an oil-immersion objective with an NA of 1.45, and a condenser with an NA of 0.95, the theoretical limit of resolution according to the Rayleigh Criterion will be:

R = 1.22 × 514 nm / (1.45 + 0.95) = 261 nm

This demonstrates that the Rayleigh Criterion provides a slightly more conservative estimate of the resolution compared to the Abbe diffraction limit formula.

Full Width at Half Maximum (FWHM) and Microscope Resolution

Another way to measure the resolution of a microscope is the full width at half maximum (FWHM) of the Airy pattern. The FWHM is the width of the central peak of the Airy pattern at half its maximum intensity.

The FWHM can be calculated using the following formula:

FWHM = 0.51λ/NA

Applying this to the same example, with a light wavelength of 514 nm and an objective with an NA of 1.45, the theoretical resolution based on the FWHM will be:

FWHM = 0.51 × 514 nm / 1.45 = 181 nm

This value represents the minimum distance between two points that can be distinguished as separate features in the microscope image.

Factors Affecting Microscope Resolution

To achieve the maximum theoretical resolution of a microscope system, several factors must be considered:

1. Wavelength of Light: Using a shorter wavelength of light, such as ultraviolet or blue-violet, can improve the resolution of the microscope.

2. Numerical Aperture (NA): Choosing an objective lens with the highest available NA can significantly enhance the resolution.

3. Condenser Numerical Aperture: Ensuring that the numerical aperture of the condenser lens is matched to or slightly less than the numerical aperture of the objective lens can optimize the resolution.

4. Microscope Alignment: Proper alignment of the entire microscope system, including the illumination, condenser, and objective, is crucial for achieving the best possible resolution.

5. Specimen Preparation: Proper sample preparation, such as staining or labeling, can enhance the contrast and visibility of the features of interest, improving the overall resolution.

6. Environmental Factors: Controlling factors like temperature, humidity, and vibrations can minimize disturbances that can degrade the microscope’s resolution.

Practical Considerations and Limitations

While the microscope diffraction limit formulas provide a theoretical understanding of the resolution limits, there are practical considerations and limitations to keep in mind:

1. Optical Aberrations: Imperfections in the optical components of the microscope, such as lens distortions or chromatic aberrations, can degrade the actual resolution achieved.

2. Specimen Characteristics: The nature of the specimen, its refractive index, and the presence of scattering or absorbing materials can influence the effective resolution.

3. Imaging Techniques: Advanced imaging techniques, such as confocal microscopy or super-resolution microscopy, can overcome the diffraction limit and achieve higher resolutions.

4. Practical Limitations: In real-world applications, factors like sample preparation, environmental conditions, and instrument limitations may prevent the achievement of the theoretical maximum resolution.

Conclusion

The microscope diffraction limit formula is a fundamental principle in the field of microscopy, governing the smallest feature size that can be resolved using an optical microscope. By understanding the Abbe diffraction limit, the Rayleigh Criterion, and the FWHM, you can gain a comprehensive understanding of the factors that influence microscope resolution and the strategies to optimize it.

Remember, the diffraction limit is not a rigid constraint, but rather a guiding principle that can be pushed through the use of advanced imaging techniques and careful consideration of the various factors affecting resolution. Mastering the microscope diffraction limit formula is a crucial step in becoming a proficient microscopist and advancing your research in the field of microscopy.

References

1. Microscope Resolution: Concepts, Factors and Calculation, Leica Microsystems, https://www.leica-microsystems.com/science-lab/life-science/microscope-resolution-concepts-factors-and-calculation/
2. Single Slit Diffraction, Physics – Lumen Learning, https://courses.lumenlearning.com/suny-physics/chapter/27-5-single-slit-diffraction/
3. The Diffraction Barrier in Optical Microscopy, Nikon’s MicroscopyU, https://www.microscopyu.com/techniques/super-resolution/the-diffraction-barrier-in-optical-microscopy
4. How Diffraction Limits the Optical Resolution of a Microscope, Scientific Imaging, https://scientificimaging.com/knowledge-base/how-diffraction-limits-the-optical-resolution-of-a-microscope/
5. The Diffraction Limit, Emma Benjaminson, https://sassafras13.github.io/DiffractionLimit/