Comprehensive Guide: How to Measure Energy Distribution in Blackbody Radiation

Measuring the energy distribution in blackbody radiation is a crucial task in various fields of physics, including astrophysics, thermal engineering, and quantum mechanics. This comprehensive guide will provide you with a detailed understanding of the fundamental principles and practical techniques involved in quantifying the energy distribution of blackbody radiation.

Planck’s Law of Blackbody Radiation

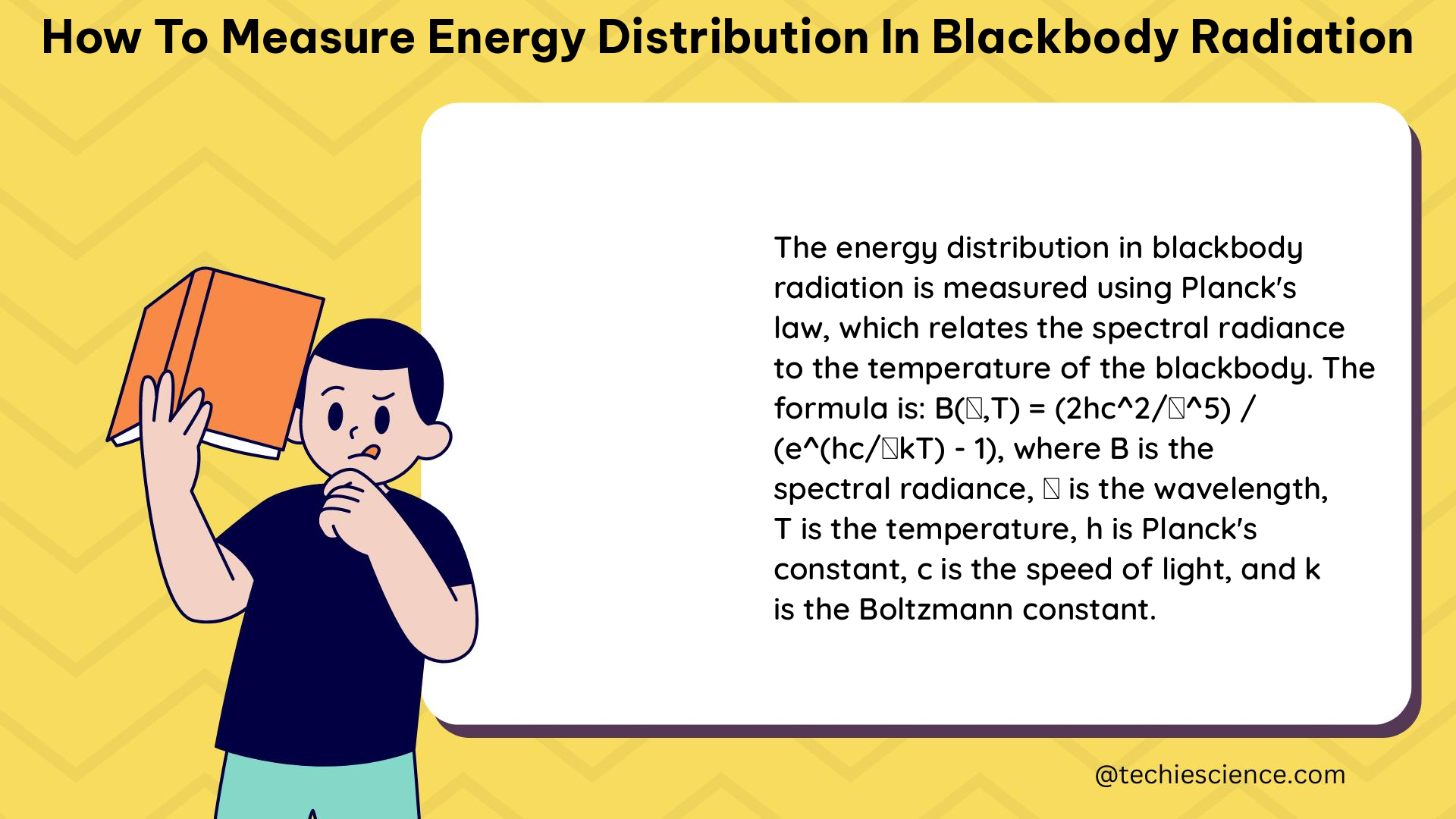
The foundation for measuring the energy distribution in blackbody radiation is Planck’s law, which describes the spectral radiance (power per unit solid angle and per unit of area normal to the propagation) density of frequency ν radiation per unit frequency at thermal equilibrium at temperature T. Planck’s law is expressed as:

Bν(T) = (2hν³) / (c² (e^(hν/kT) – 1))

Where:
– Bν(T) is the spectral radiance density of frequency ν radiation per unit frequency at thermal equilibrium at temperature T. The units are power per [area × solid angle × frequency].
– h is the Planck constant (6.626 × 10^-34 J·s).
– c is the speed of light in a vacuum (3.00 × 10^8 m/s).
– k is the Boltzmann constant (1.38 × 10^-23 J/K).
– ν is the frequency of the electromagnetic radiation.
– T is the absolute temperature of the blackbody in Kelvin (K).

By measuring the spectral radiance at different frequencies, you can determine the energy distribution of the blackbody radiation.

Wien’s Displacement Law

In addition to Planck’s law, Wien’s displacement law is another important tool for measuring the energy distribution in blackbody radiation. This law states that the wavelength at which the spectral radiance of a blackbody is maximum, λmax, is inversely proportional to the temperature, T, of the blackbody. The relationship is expressed as:

λmax = (b / T)

Where:
– λmax is the wavelength at which the spectral radiance of the blackbody is maximum.
– b is the Wien displacement constant, which has a value of 2.898 × 10^-3 m·K.
– T is the absolute temperature of the blackbody in Kelvin (K).

By measuring the wavelength at which the spectral radiance of a blackbody is maximum, you can determine the temperature of the blackbody using Wien’s displacement law.

Stefan-Boltzmann Law

The Stefan-Boltzmann law is another important relationship that can be used to measure the energy distribution in blackbody radiation. This law states that the total power radiated per unit area of a blackbody is directly proportional to the fourth power of the blackbody’s absolute temperature. The relationship is expressed as:

j* = σ T⁴

Where:
– j* is the total power radiated per unit area of a blackbody.
– σ is the Stefan-Boltzmann constant, which has a value of 5.670 × 10^-8 W/(m²·K⁴).
– T is the absolute temperature of the blackbody in Kelvin (K).

By measuring the total power radiated by a blackbody and its temperature, you can use the Stefan-Boltzmann law to determine the energy distribution of the blackbody radiation.

Experimental Techniques for Measuring Energy Distribution

To measure the energy distribution in blackbody radiation, you can use various experimental techniques, including:

1. Spectroscopy: Use a spectrometer to measure the spectral radiance of the blackbody radiation at different frequencies. This data can then be used to plot the energy distribution and compare it to Planck’s law.

2. Pyrometry: Use a pyrometer, which is a device that measures the temperature of an object by detecting the infrared radiation it emits. By measuring the temperature of the blackbody, you can use Wien’s displacement law and the Stefan-Boltzmann law to determine the energy distribution.

3. Bolometry: Use a bolometer, which is a device that measures the total power of incident electromagnetic radiation. By measuring the total power radiated by the blackbody and its temperature, you can use the Stefan-Boltzmann law to determine the energy distribution.

4. Interferometry: Use an interferometer to measure the coherence properties of the blackbody radiation, which can be related to the energy distribution through the Wiener-Khinchin theorem.

5. Calorimetry: Use a calorimeter to measure the heat absorbed or emitted by the blackbody, which can be used to determine the energy distribution.

Each of these techniques has its own advantages and limitations, and the choice of method will depend on the specific requirements of your experiment, the available equipment, and the desired level of accuracy.

Practical Considerations and Limitations

When measuring the energy distribution in blackbody radiation, it is important to consider the following practical considerations and limitations:

1. Thermal Equilibrium: Planck’s law and the other laws mentioned assume that the blackbody is in thermal equilibrium. In practice, this condition may not be perfectly met, and corrections may need to be made to account for deviations from thermal equilibrium.

2. Isotropy: The laws also assume that the blackbody radiation is isotropic, meaning that the spectral radiance is the same in all directions. In some cases, the radiation may not be perfectly isotropic, and corrections may be necessary.

3. Emissivity: Real-world materials may not have a perfect blackbody emissivity of 1, which can affect the measured energy distribution. The emissivity of the material should be taken into account when interpreting the results.

4. Experimental Uncertainties: Measurements of spectral radiance, temperature, and power can be subject to various experimental uncertainties, such as instrument calibration, measurement errors, and environmental factors. These uncertainties should be properly accounted for in the analysis.

5. Frequency Range: The frequency range over which the measurements are made can also affect the accuracy of the energy distribution determination. Ideally, the measurements should cover a wide range of frequencies to capture the full energy distribution.

By understanding these practical considerations and limitations, you can design and execute experiments that provide accurate and reliable measurements of the energy distribution in blackbody radiation.

Conclusion

Measuring the energy distribution in blackbody radiation is a fundamental task in various fields of physics. This comprehensive guide has provided you with a detailed understanding of the underlying principles, experimental techniques, and practical considerations involved in this process. By mastering the concepts and methods presented here, you can become an expert in quantifying the energy distribution of blackbody radiation and apply this knowledge to your research or practical applications.

References

1. Planck, M. (1901). “Über das Gesetz der Energieverteilung im Normalspectrum”. Annalen der Physik. 309 (3): 553–563.
2. Wien, W. (1896). “Über die Energieverteilung im Emissionsspektrum eines schwarzen Körpers”. Annalen der Physik. 294 (8): 662–669.
3. Stefan, J. (1879). “Über die Beziehung zwischen der Wärmestrahlung und der Temperatur”. Sitzungsberichte der mathematisch-naturwissenschaftlichen Classe der kaiserlichen Akademie der Wissenschaften. 79: 391–428.
4. Boltzmann, L. (1884). “Ableitung des Stefan’schen Gesetzes, betreffend die Abhängigkeit der Wärmestrahlung von der Temperatur aus der electromagnetischen Lichttheorie”. Annalen der Physik. 258 (6): 291–294.
5. Siegel, D. M. (1976). “Balfour Stewart and Gustav Kirchhoff: Two Independent Approaches to Kirchhoff’s Law”. Isis. 67 (4): 565–600.
6. Planck, M. (1914). The Theory of Heat Radiation. P. Blakiston’s Son & Co.
7. Rybicki, G. B., & Lightman, A. P. (1979). Radiative Processes in Astrophysics. Wiley-Interscience.
8. Modest, M. F. (2013). Radiative Heat Transfer. Academic Press.