Mastering the Art of Finding Velocity 2: A Comprehensive Guide

In the realm of physics, understanding the concept of velocity is crucial. Velocity, which represents the rate of change in an object’s position over time, is a fundamental quantity that governs the motion of objects. When it comes to analyzing the motion of an object, the ability to determine the second velocity, or velocity 2, is a valuable skill. This comprehensive guide will delve into the intricacies of finding velocity 2, equipping you with the necessary knowledge and tools to tackle this concept with confidence.

Understanding Velocity and Acceleration

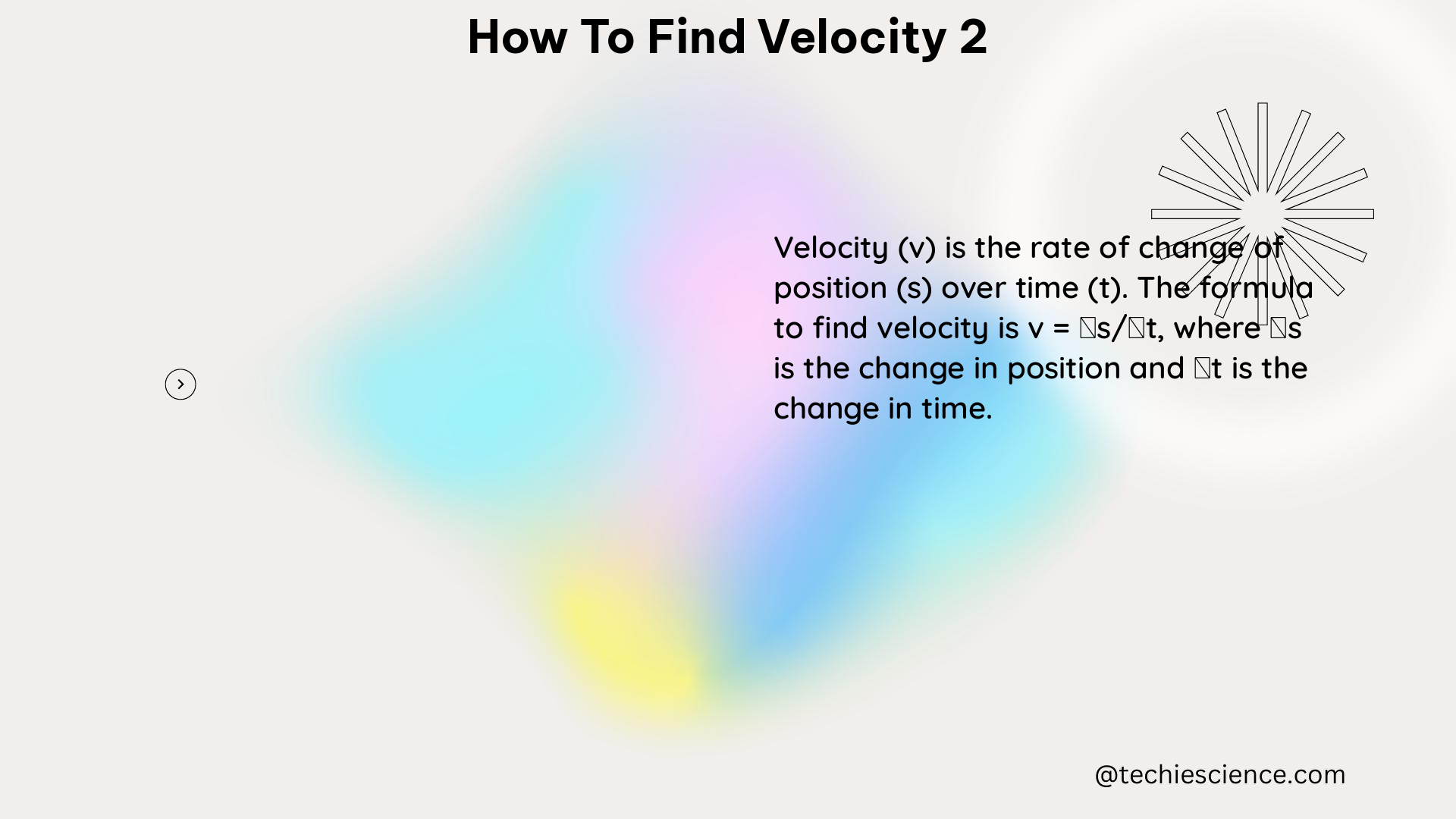
To begin, let’s revisit the fundamental principles of velocity and acceleration. Velocity is defined as the change in an object’s position divided by the change in time, expressed mathematically as:

v = Δx/Δt

where v represents the velocity, Δx represents the change in position, and Δt represents the change in time.

Acceleration, on the other hand, is the rate of change in velocity over time, expressed as:

a = Δv/Δt

where a represents the acceleration, Δv represents the change in velocity, and Δt represents the change in time.

Understanding these basic relationships is crucial in determining velocity 2, as it allows us to apply the appropriate formulas and principles to solve various problems.

Calculating Velocity 2 from Position-Time Data

One of the most common methods for finding velocity 2 is to use the position-time data of an object. If you are given the initial position (x1) at time t1 and the final position (x2) at time t2, you can calculate the average velocity (velocity 1) using the formula:

v1 = (x2 - x1) / (t2 - t1)

To find the second velocity (velocity 2) at a specific time t, you can use the following steps:

1. Determine the total displacement (Δx) and the total time (Δt) between the initial and final positions.
2. Calculate the average velocity (velocity 1) using the formula above.
3. Assuming constant acceleration, you can find the second velocity (velocity 2) at time t by using the formula:

v2 = v1 + a * (t - t1)

where a represents the acceleration of the object.

This approach allows you to determine the second velocity (velocity 2) at any point in time, provided that you have the necessary position-time data and the assumption of constant acceleration holds true.

Velocity 2 from Velocity-Time Graphs

Another method for finding velocity 2 is to use a velocity-time graph. If you are given a velocity-time graph, you can directly read the value of the velocity at the desired time to determine the second velocity (velocity 2).

To illustrate this, let’s consider an example:

Suppose you have a velocity-time graph that shows the velocity of an object over time. If you want to find the second velocity (velocity 2) at a specific time t, you can simply locate the point on the graph corresponding to that time and read the value of the velocity at that point.

It’s important to note that the velocity-time graph can also provide information about the acceleration of the object. The slope of the velocity-time graph represents the acceleration, which can be useful in calculating the second velocity (velocity 2) using the formula:

v2 = v1 + a * (t - t1)

where a is the acceleration obtained from the slope of the velocity-time graph.

Numerical Examples and Problem-Solving Strategies

To further solidify your understanding of finding velocity 2, let’s explore some numerical examples and problem-solving strategies.

Example 1: Constant Acceleration
A car travels from position x1 = 10 m at time t1 = 0 s to position x2 = 40 m at time t2 = 4 s. Calculate the average velocity (velocity 1) and the second velocity (velocity 2) at time t = 2 s.

Solution:
1. Calculate the average velocity (velocity 1) using the formula:
v1 = (x2 - x1) / (t2 - t1) = (40 - 10) / (4 - 0) = 30/4 = 7.5 m/s
2. To find the second velocity (velocity 2) at time t = 2 s, we can use the formula:
v2 = v1 + a * (t - t1)
Assuming constant acceleration, we can calculate the second velocity (velocity 2) as:
v2 = 7.5 m/s + a * (2 - 0) = 7.5 m/s + a * 2
Since the car travels 30 m in 4 s, its average speed during this time is 7.5 m/s. If it travels at a constant speed, it would cover 15 m in 2 s (half of the total distance in half of the total time). Therefore, the second velocity (velocity 2) is 15 m/2 s = 7.5 m/s.

Example 2: Vertical Motion with Gravity
A ball is thrown vertically upward with an initial velocity of 20 m/s. What is its velocity after 3 s?

Solution:
In this case, we can use the formula for velocity as a function of time, which is:

v(t) = v0 - gt

where v0 is the initial velocity, g is the acceleration due to gravity (9.8 m/s^2), and t is the time.

After 3 s, the ball’s velocity is:

v(3) = 20 - 9.8 * 3 = -1.4 m/s

The negative sign indicates that the ball is moving downward.

These examples demonstrate the application of the formulas and principles discussed earlier. By understanding the underlying concepts and practicing problem-solving strategies, you can confidently tackle a wide range of velocity 2 problems.

Advanced Techniques and Considerations

While the methods discussed so far cover the basic principles of finding velocity 2, there are additional techniques and considerations that can be useful in more complex scenarios.

1. Non-constant Acceleration: If the acceleration of the object is not constant, you may need to use more advanced formulas, such as the kinematic equations, to determine the second velocity (velocity 2).

2. Calculus-based Approach: For situations with continuously varying velocity, you can use calculus-based methods, such as differentiation, to find the second velocity (velocity 2) at a specific time.

3. Numerical Simulations: In some cases, where analytical solutions are not feasible, you may need to resort to numerical simulations to model the object’s motion and determine the second velocity (velocity 2).

4. Experimental Measurements: In real-world applications, you may need to rely on experimental data and measurements to determine the second velocity (velocity 2). This may involve the use of specialized equipment, such as motion sensors or high-speed cameras.

5. Coordinate Systems and Frames of Reference: Depending on the problem, you may need to consider the appropriate coordinate system and frame of reference to accurately calculate the second velocity (velocity 2).

By exploring these advanced techniques and considerations, you can expand your problem-solving capabilities and tackle more complex scenarios involving the determination of velocity 2.

Conclusion

In this comprehensive guide, we have delved into the intricacies of finding velocity 2 in physics. From understanding the fundamental principles of velocity and acceleration to applying various techniques, such as using position-time data and velocity-time graphs, you now have a solid foundation to tackle a wide range of velocity 2 problems.

Remember, the key to mastering the art of finding velocity 2 lies in your ability to apply the appropriate formulas, understand the underlying physics concepts, and develop a systematic problem-solving approach. By practicing the examples and exploring the advanced techniques presented in this guide, you will be well on your way to becoming a proficient problem-solver in the realm of velocity and motion analysis.

Reference:

1. Calculating Speed and Velocity From a Data Table – YouTube
2. How to Calculate Velocity – wikiHow
3. Motion of a Mass on a Spring – The Physics Classroom