How to Find Top Speed with Acceleration and Time: A Comprehensive Guide for Physics Students

Summary

To find the top speed with acceleration and time, we can use the formula v = u + at, where v is the top speed, u is the initial velocity (usually 0), a is the acceleration, and t is the time. First, we need to find the time it takes to reach the top speed using the formula t = (v - u) / a. Once we have the time, we can substitute it back into the first formula to find the top speed. This guide will provide a detailed explanation of these concepts, along with examples, physics formulas, and numerical problems to help physics students master the topic.

Understanding the Relationship between Acceleration, Velocity, and Time

In physics, the relationship between acceleration, velocity, and time is described by the SUVAT equations, also known as the kinematic equations. These equations are fundamental to understanding and solving problems related to motion with constant acceleration.

The SUVAT equations are:

1. v = u + at
2. s = ut + 0.5at^2
3. v^2 = u^2 + 2as
4. t = (v - u) / a

Where:
– v is the final velocity (m/s)
– u is the initial velocity (m/s)
– a is the acceleration (m/s^2)
– t is the time (s)
– s is the displacement (m)

These equations can be rearranged and used to solve for different variables, depending on the information given in the problem.

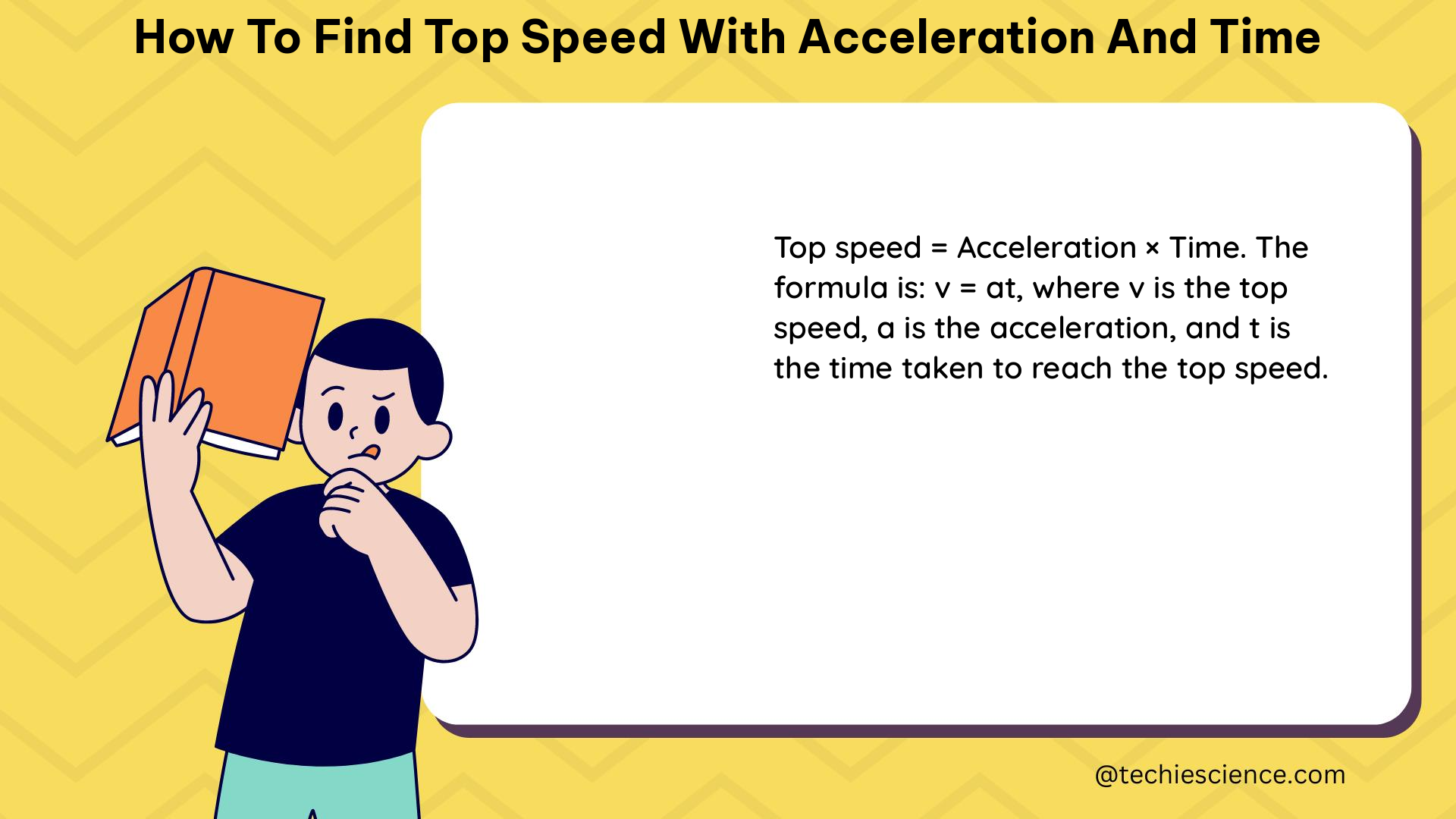
Calculating Top Speed with Acceleration and Time

To find the top speed with acceleration and time, we can use the following formula:

v = u + at

Where:
– v is the top speed (m/s)
– u is the initial velocity (usually 0 m/s)
– a is the acceleration (m/s^2)
– t is the time (s)

To find the time it takes to reach the top speed, we can use the formula:

t = (v - u) / a

Once we have the time, we can substitute it back into the first formula to find the top speed.

Example 1: Finding Top Speed of a Car

Let’s say we have a car with an acceleration of 5 m/s^2, and it takes 10 seconds to reach its top speed. We can use the formula for time to find the top speed:

t = (v - u) / a
10 = (v - 0) / 5
v = 50 m/s

So, the top speed of the car is 50 m/s.

Example 2: Finding Top Speed of a Sprinter

In the case of a sprinter running a 100m race, we can use the same principles to find the time it takes to reach the top speed and the time it takes to run the rest of the race at the top speed.

Let’s say the sprinter’s top speed is 15 m/s, and it takes 3 seconds to reach that speed. We can use the formula for time to find the acceleration:

t = (v - u) / a
3 = (15 - 0) / a
a = 5 m/s^2

Now that we know the acceleration, we can use the formula for distance to find out how far the sprinter has traveled in those 3 seconds:

d = 0.5 × a × t^2
d = 0.5 × 5 × 3^2
d = 22.5 m

So, the sprinter has traveled 22.5 m in the first 3 seconds. Now, we need to find out how long it takes to run the remaining 77.5 m at the top speed of 15 m/s:

t = d / v
t = 77.5 / 15
t = 5.167 s

The total time taken for the race is 3 s (acceleration phase) + 5.167 s (maximum speed phase) = 8.167 s.

Numerical Problems

1. A car accelerates from rest to a top speed of 80 m/s in 20 seconds. Calculate the acceleration and the top speed.

Given:
– Initial velocity, u = 0 m/s
– Time, t = 20 s
– Top speed, v = 80 m/s

To find the acceleration:
v = u + at
80 = 0 + a × 20
a = 4 m/s^2

Therefore, the acceleration of the car is 4 m/s^2.

1. A sprinter accelerates from rest to a top speed of 12 m/s in 3 seconds. Calculate the distance traveled during the acceleration phase.

Given:
– Initial velocity, u = 0 m/s
– Time, t = 3 s
– Top speed, v = 12 m/s

To find the distance traveled during the acceleration phase:
s = ut + 0.5at^2
s = 0 × 3 + 0.5 × a × 3^2
s = 0 + 0.5 × a × 9
s = 0.5 × a × 9

To find the acceleration, a:
v = u + at
12 = 0 + a × 3
a = 4 m/s^2

Substituting the acceleration, we get:
s = 0.5 × 4 × 9
s = 18 m

Therefore, the distance traveled during the acceleration phase is 18 m.

Conclusion

In this comprehensive guide, we have explored the concepts of acceleration, velocity, and time, and how they are related through the SUVAT equations. We have also learned how to use these equations to find the top speed with acceleration and time, with detailed examples and numerical problems to help physics students master the topic.

By understanding the underlying principles and applying the appropriate formulas, physics students can confidently solve a wide range of problems related to motion with constant acceleration, including finding the top speed, time, and distance traveled.

References

1. Calculate time to accelerate to Max Velocity – Chief Delphi
   https://www.chiefdelphi.com/t/calculate-time-to-accelerate-to-max-velocity/348542
2. Calculate max velocity given time, distance and acceleration – Math Stack Exchange
   https://math.stackexchange.com/questions/3304696/calculate-max-velocity-given-time-distance-and-acceleration
3. Calculate time from Max Velocity, Acceleration & Distance – Reddit
   https://www.reddit.com/r/learnmath/comments/hx4gqg/calculate_time_from_max_velocity_acceleration/
4. Calculating Acceleration from Velocity and Time | CK-12 Foundation
   https://flexbooks.ck12.org/cbook/ck-12-middle-school-physical-science-flexbook-2.0/section/9.8/primary/lesson/calculating-acceleration-from-velocity-and-time-ms-ps/
5. How to Calculate Time and Distance from Acceleration and Velocity – Dummies
   https://www.dummies.com/article/academics-the-arts/science/physics/how-to-calculate-time-and-distance-from-acceleration-and-velocity-174278/