In the world of physics and mathematics, understanding the concept of tangential acceleration is crucial. It plays a significant role in analyzing the motion of objects in circular or rotational motion. In this blog post, we will explore the concept of tangential acceleration in detail, including its definition, importance, and how to calculate it in various scenarios. So, let’s dive in!
How to Find Tangential Acceleration
Definition of Tangential Acceleration
tangential acceleration refers to the rate at which the tangential velocity of an object changes over time in a circular or rotational motion. It is a measure of how quickly an object’s speed or direction changes along the circular path it follows. In simple terms, it represents the acceleration experienced by an object moving in a circle.
Importance of Tangential Acceleration in Physics and Mathematics
tangential acceleration is essential in understanding the dynamics of rotational motion. It helps us analyze and predict how objects move in circular paths, such as planets orbiting the sun, cars taking turns on a racetrack, or even the motion of a spinning top. By considering tangential acceleration, we can determine the forces acting on an object, its velocity, and how it responds to external influences.
The Formula to Find Tangential Acceleration
The formula to calculate tangential acceleration depends on various factors, including angular acceleration, time, and linear velocity. It can be expressed as:
![]()
Where:
– ![]() represents the tangential acceleration
represents the tangential acceleration
– ![]() is the radius of the circular path
is the radius of the circular path
– ![]() denotes the angular acceleration
denotes the angular acceleration
Now that we have a clear understanding of tangential acceleration let’s explore how to calculate it in different scenarios.
How to Calculate Tangential Acceleration

Calculating Tangential Acceleration from Angular Acceleration

To calculate tangential acceleration from angular acceleration, we can use the formula mentioned earlier: ![]() . Let’s consider an example to illustrate this:
. Let’s consider an example to illustrate this:
Example 1:
Suppose a particle is moving in a circular path with a radius of 3 meters, and it experiences an angular acceleration of 2 rad/s². To find the tangential acceleration, we can apply the formula:
![]()
![]()
Hence, the tangential acceleration is 6 m/s².
Finding Tangential Acceleration Given Time
Sometimes, we may need to calculate tangential acceleration when the time is given. In such cases, we can use a different formula based on the initial angular velocity, the angular acceleration, and the time. The formula is:
![]()
Where:
– (a_t) represents the tangential acceleration
– (\omega_0) is the initial angular velocity
– (\alpha) denotes the angular acceleration
– (t) is the time
Example 2:
Let’s consider a scenario where an object starts from rest and experiences an angular acceleration of 5 rad/s² for a duration of 2 seconds. The initial angular velocity ![]() is 0. By substituting the given values, we can calculate the tangential acceleration:
is 0. By substituting the given values, we can calculate the tangential acceleration:
![]()
![]()
Hence, the tangential acceleration is 10 m/s².
Determining Tangential Acceleration Without Time
In some cases, we may need to determine the tangential acceleration without knowing the time duration. In such situations, we can use equations that involve the angular velocity ![]() , the radius (r), and the tangential acceleration (at). One such equation is:
, the radius (r), and the tangential acceleration (at). One such equation is:
![]()
Example 3:
Suppose an object is moving in a circular path with a radius of 2 meters and has an angular velocity of 3 rad/s. To find the tangential acceleration, we can use the formula:
![]()
![]()
Therefore, the tangential acceleration is 18 m/s².
Now that we have covered the basics of calculating tangential acceleration, let’s explore how to solve it in different scenarios.
How to Solve for Tangential Acceleration in Different Scenarios
Finding Tangential Acceleration in Circular Motion

When dealing with circular motion, tangential acceleration is an important parameter to consider. It helps us understand how objects accelerate along the circular path. In circular motion, tangential acceleration is always directed towards the center of the circle. The magnitude of tangential acceleration depends on factors like angular acceleration, radius, and linear velocity.
Determining Tangential Acceleration of a Pendulum
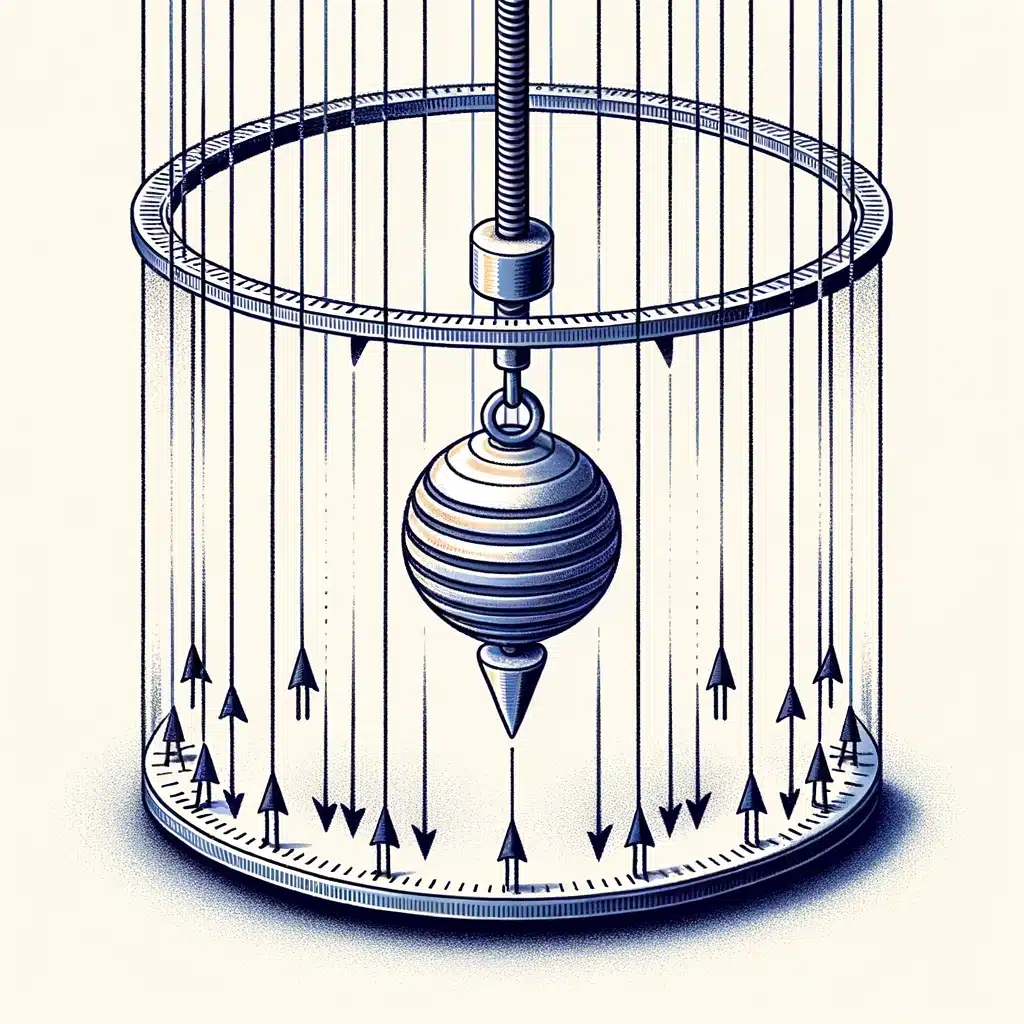
A pendulum is an excellent example where tangential acceleration comes into play. When a pendulum swings back and forth, the bob experiences tangential acceleration. The magnitude of tangential acceleration is determined by the length of the pendulum, the angle it swings, and the gravitational acceleration.
Calculating Tangential Acceleration in Vertical Circular Motion

In vertical circular motion, the tangential acceleration helps us understand how objects accelerate or decelerate as they move up or down along the circular path. The tangential acceleration in vertical circular motion varies depending on the location of the object in the circular path. At the topmost point, the tangential acceleration is directed downward, while at the bottommost point, it is directed upwards.
How to Find Tangential Velocity and Speed with Centripetal Acceleration
Finding Tangential Velocity with Centripetal Acceleration and Radius
tangential velocity represents the linear velocity of an object moving along a circular path. It is related to centripetal acceleration (the acceleration towards the center of the circle) and the radius of the circular path. The formula to calculate tangential velocity is:
![]()
Where:
– ![]() represents the tangential velocity
represents the tangential velocity
– ![]() is the centripetal acceleration
is the centripetal acceleration
– ![]() denotes the radius
denotes the radius
Calculating Tangential Speed with Centripetal Acceleration
tangential speed refers to the magnitude of the tangential velocity. It represents how fast an object is moving along a circular path. To calculate tangential speed, we need to know the tangential acceleration and the time it takes for the object to complete one revolution around the circle. The formula for tangential speed is:
![]()
Where:
– ![]() represents the tangential speed
represents the tangential speed
– ![]() is the tangential acceleration
is the tangential acceleration
– ![]() denotes the time
denotes the time
How to Find Tangential Component of Linear Acceleration
Finding Tangential Acceleration from Radial Acceleration
In certain cases, we may need to determine the tangential acceleration from the radial acceleration. Radial acceleration is the component of acceleration directed towards or away from the center of the circle. It is perpendicular to the tangential acceleration. To find the tangential acceleration from radial acceleration, we can use the following formula:
![]()
Where:
– ![]() represents the tangential acceleration
represents the tangential acceleration
– ![]() is the radial acceleration
is the radial acceleration
Calculating Tangential Acceleration from Tangential Velocity
In some scenarios, we may need to find the tangential acceleration using the tangential velocity and the time taken to change the velocity. The formula to calculate tangential acceleration in such cases is:
![]()
Where:
– ![]() represents the tangential acceleration
represents the tangential acceleration
– ![]() is the final tangential velocity
is the final tangential velocity
– ![]() denotes the initial tangential velocity
denotes the initial tangential velocity
– ![]() is the time
is the time
Determining Tangential Acceleration from Velocity
Sometimes, we may need to find the tangential acceleration when only the velocity of the object is known. In such cases, we can use the following formula:
![]()
Where:
– ![]() represents the tangential acceleration
represents the tangential acceleration
– ![]() is the tangential velocity
is the tangential velocity
– ![]() denotes the radius
denotes the radius
How to Find Acceleration Tangential and Normal
When an object moves in a circular path, it experiences two types of acceleration: tangential acceleration and radial or centripetal acceleration. tangential acceleration is responsible for the change in the object’s speed or direction along the circular path, while radial acceleration keeps the object moving towards the center of the circle. The sum of these two accelerations gives the total acceleration of the object.
How to Find Direction of Tangential Acceleration
The direction of tangential acceleration is determined by the change in the object’s velocity along the circular path. It always points tangent to the circular path, either in the same direction as the motion or in the opposite direction, depending on whether the object is accelerating or decelerating.
Multivariable Questions on Tangential Acceleration
How to Find Tangential Acceleration with Multiple Variables
In more complex scenarios, we may come across questions that involve multiple variables to find the tangential acceleration. To solve these problems, we need to carefully analyze the given information, identify the relevant formulas, and apply them step by step. Let’s consider an example:
Example 4:
Suppose an object is moving along a circular path with a radius of 5 meters. The object’s tangential velocity is 10 m/s, and the time taken to complete one revolution is 4 seconds. To find the tangential acceleration, we can use the formula:
![]()
Substituting the given values:
![]()
![]()
![]()
Hence, the tangential acceleration is 2.5 m/s².
Quick Facts :
Q: What is the concept of tangential acceleration?
A: The concept of tangential acceleration is related to the acceleration of an object moving in a circular path. It can be understood as the rate of change in the speed of the object along its tangential direction. It is known as tangential acceleration because the direction of the acceleration vector is tangential to the direction of the velocity vector at any given point.
Q: What is the formula for tangential acceleration?
A: The formula for tangential acceleration is a = r * α, where ‘a’ represents the tangential acceleration, ‘r’ is the radius, and ‘α’ represents the angular acceleration of the object. It is the product of the radius of the motion and the angular acceleration.
Q: How does tangential acceleration relate to uniform circular motion?
A: In uniform circular motion, the magnitude of the velocity remains constant but the direction of the velocity changes continuously. Hence, there is an additional acceleration acting along the radius towards the center, known as centripetal acceleration. If the object executing circular motion has uniform acceleration, then the tangential acceleration is zero.
| Attribute Of Tangential Acceleration | Characteristic in Uniform Circular Motion |
|---|---|
| Presence | None (tangential acceleration is zero) |
| Role | Not applicable (since speed is constant) |
| Direction | No direction (as there is no tangential acceleration) |
| Magnitude | 0 m/s² (no change in the magnitude of velocity) |
| Effect on Speed | No effect (speed is constant) |
| Effect on Trajectory | No effect (trajectory remains circular at constant radius) |
| Resulting Motion Type | Uniform circular motion (constant speed, constant radius) |
| Necessary Conditions | No net force in the tangential direction |
| Equation |
Q: What’s the difference between radial and tangential acceleration?
| Attribute | Radial (Centripetal) Acceleration | Tangential Acceleration |
|---|---|---|
| Vector Relationship | Always points radially inward regardless of the object’s motion direction. | Aligned with the instantaneous direction of velocity change, either forward or backward along the path. |
| Dependence on Velocity | Depends on the square of the tangential velocity (speed) and inversely on the radius of curvature. | Directly related to the rate of change of the object’s speed, irrespective of its path curvature. |
| Role in Circular Motion | Provides the necessary force component to keep an object in a circular path without influencing the object’s speed. | Responsible for the change in speed of an object in circular motion, without affecting the radius of the path. |
| Independence from Speed | Independent of changes in the object’s speed; an object in uniform circular motion has constant radial acceleration. | Directly dependent on changes in speed; without a change in speed, tangential acceleration is nonexistent. |
| Represented in Equations | Prominently features in Newton’s second law for rotational motion (F=ma_r) when considering the force necessary for circular motion. | Featured in the kinematic equations of motion when an object’s speed is changing. |
| Measurement | Measured in terms of centripetal force required per unit mass to maintain the circular path (N/kg or m/s²). | Measured as the rate of change of speed, indicating how quickly an object accelerates or decelerates (m/s²). |
| In Rotational Dynamics | Analogous to force in linear dynamics, but for rotating systems, it represents the radial force per mass needed to maintain rotation. | Analogous to the force component in linear dynamics that causes a change in kinetic energy due to speed variation. |
| Work Done | Does no work because the radial acceleration is perpendicular to the displacement of the object in circular motion. | Does work as it is in the direction of displacement, contributing to a change in the kinetic energy of the object. |
| Effect on Angular Momentum | Does not change the angular momentum of an object in a closed system since there is no torque involved. | Can change the angular momentum if it is associated with a torque, affecting the rotational speed. |
| Energy Consideration | Since it doesn’t change the speed, it doesn’t directly contribute to a change in kinetic energy; it affects potential energy in a gravitational field. | Directly affects kinetic energy as it changes the speed; in a gravitational field, it can also affect potential energy. |
Q: What does tangential acceleration tell us?
A: Tangential acceleration gives us an idea about how rapidly the speed of an object is changing with time in the tangential direction. If tangential acceleration is positive, the object is speeding up. If it is negative, the object is slowing down.
Q: How does the tangential acceleration formula apply to solving problems?
A: The tangential acceleration formula is particularly useful in cases where an object moves in a circular path and its speed changes at a uniform rate. It helps calculate the change in speed at any given point of time. The formula can be applied directly or by integrating the equation if the angular acceleration is not constant.
Q: Could you provide a solved example using the tangential acceleration formula?
A: Sure. Suppose an object is moving on a circular path of radius 4 meters with an angular acceleration of 2 rad/s². The tangential acceleration (a) would be a = r * α = 4 m * 2 rad/s² = 8 m/s². Here, we’ve used the formula for tangential acceleration to calculate the acceleration of the object.
Q: What is the relationship between total acceleration, centripetal and tangential acceleration?
A: The total acceleration of an object moving in a circular path is the vector sum of the centripetal and tangential acceleration. Mathematically, total acceleration = √((centripetal acceleration)² + (tangential acceleration)²). The centripetal acceleration is directed towards the center of the circle, whereas the tangential acceleration is in the tangent direction to the circle at that point.
Q: How are the tangential acceleration and the velocity vector related?
A: The velocity vector of an object executing circular motion has two components: the radial and the tangential. And tangential acceleration has an effect on the magnitude of the velocity vector along the tangential direction. If there is any tangential acceleration, it means that the magnitude of the velocity vector is changing.
How can tangential acceleration and angular acceleration be related?
To understand the relationship between tangential acceleration and angular acceleration, it is important to consider the concept of Finding Angular Acceleration of a Wheel. Angular acceleration refers to the rate at which the angular velocity of a rotating object changes over time. On the other hand, tangential acceleration refers to the linear acceleration experienced by an object moving in a circular path. These two concepts are interconnected because the tangential acceleration of a point on a rotating object is related to the angular acceleration of the object. By understanding how tangential acceleration and angular acceleration are connected, we can gain insights into the dynamics of rotational motion.
Q: What are the applications of tangential acceleration in real life?
A: Tangential acceleration has many practical applications in real-life situations such as turning of vehicles where the speed changes due to tangential acceleration. It’s used in the dynamics of rotational motions such as gears, pulleys, and wheels. It’s also applicable in the field of astronomy for studying the planetary motion of celestial objects.
Also Read:
- How to find mass and acceleration with force
- How to find tension force with acceleration
- How to find angular acceleration without time
- How to find acceleration projectile motion
- Torque and angular acceleration
- Centripetal acceleration and mass
- How to find acceleration with mass and radius
- How to calculate force without acceleration
- How to find total acceleration
- How to find total acceleration in circular motion
Hi, I’m Akshita Mapari. I have done M.Sc. in Physics. I have worked on projects like Numerical modeling of winds and waves during cyclone, Physics of toys and mechanized thrill machines in amusement park based on Classical Mechanics. I have pursued a course on Arduino and have accomplished some mini projects on Arduino UNO. I always like to explore new zones in the field of science. I personally believe that learning is more enthusiastic when learnt with creativity. Apart from this, I like to read, travel, strumming on guitar, identifying rocks and strata, photography and playing chess.