How to Find Spring Potential Energy: A Comprehensive Guide

The spring potential energy, also known as the elastic potential energy, is the energy stored in a spring when it is compressed or stretched from its equilibrium position. This energy can be released to do work, making it an important concept in physics and engineering. In this comprehensive guide, we will delve into the details of how to calculate spring potential energy, including the necessary formulas, examples, and practical applications.

Understanding Spring Potential Energy

Spring potential energy is the potential energy stored in a spring due to its deformation from its equilibrium position. When a spring is compressed or stretched, it stores energy that can be released to do work. The amount of energy stored in the spring is directly proportional to the square of the displacement from the equilibrium position and the spring constant.

The formula for calculating spring potential energy is:

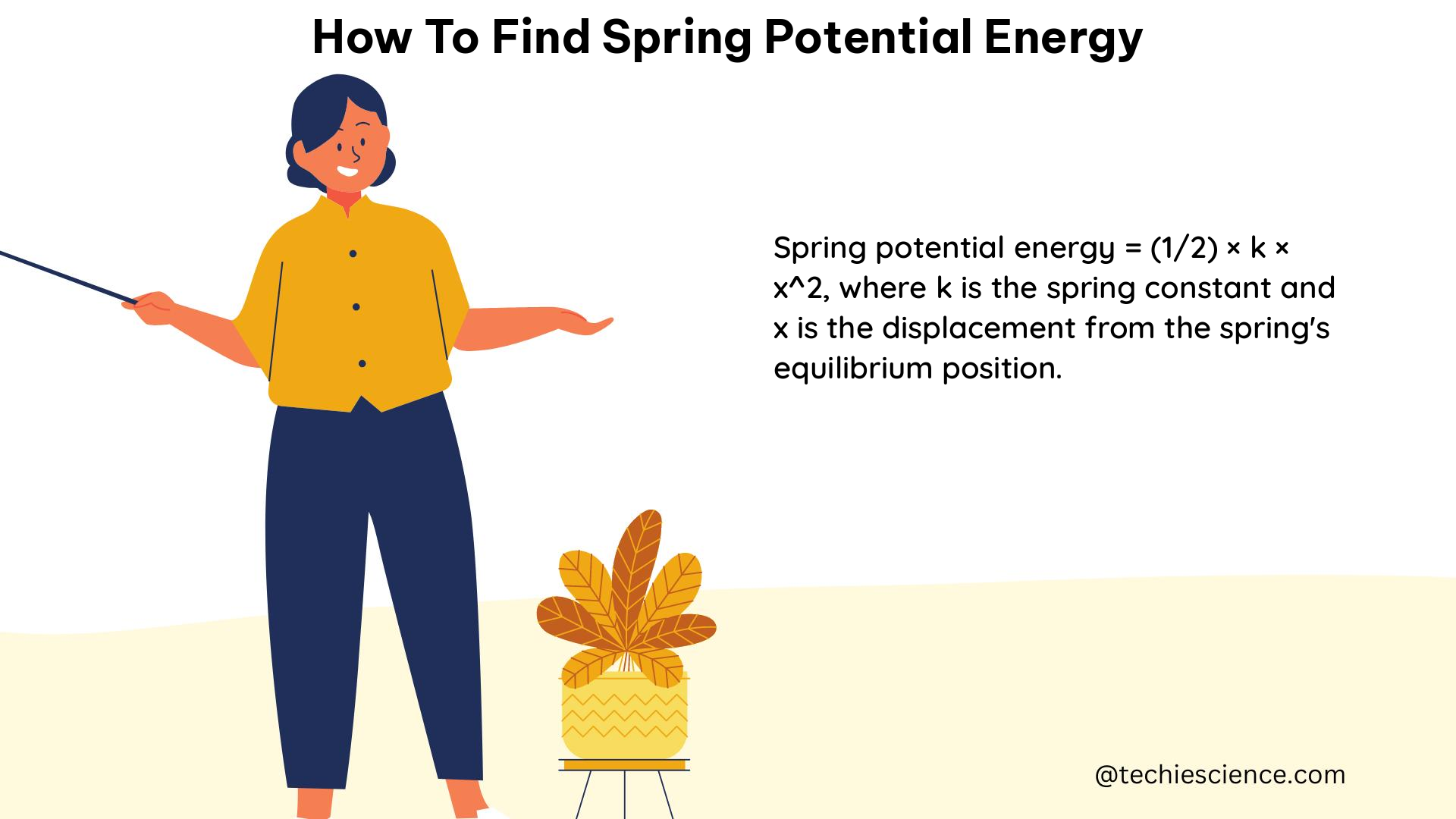
U = 1/2 * k * x^2

Where:
– U is the spring potential energy (in Joules)
– k is the spring constant (in Newtons per meter, N/m)
– x is the displacement from the equilibrium position (in meters, m)

The spring constant, k, is a measure of the stiffness of the spring. A higher spring constant means the spring is stiffer and requires more force to compress or stretch it.

Calculating Spring Potential Energy

To calculate the spring potential energy, you need to know the values of the spring constant and the displacement from the equilibrium position.

Step 1: Determine the spring constant

The spring constant, k, can be found through experimentation or provided in the problem statement. The spring constant is a measure of the stiffness of the spring and is typically expressed in Newtons per meter (N/m).

Step 2: Measure the displacement from equilibrium

The displacement, x, is the distance the spring is compressed or stretched from its equilibrium position. This value is typically provided in the problem statement or can be measured experimentally.

Step 3: Plug the values into the formula

Once you have the values for the spring constant and the displacement, you can plug them into the formula to calculate the spring potential energy:

U = 1/2 * k * x^2

Example Calculation

Let’s consider an example where a spring has a spring constant of 50 N/m and is stretched 0.2 meters from its equilibrium position.

Given:
– Spring constant, k = 50 N/m
– Displacement, x = 0.2 m

Plugging these values into the formula:
U = 1/2 * 50 N/m * (0.2 m)^2
U = 1/2 * 50 N/m * 0.04 m^2
U = 1 Joule

Therefore, the spring potential energy stored in this spring is 1 Joule.

Dealing with Multiple Springs

When dealing with an arrangement of multiple springs, you can model the system as a single equivalent spring with an equivalent spring constant, k_eq. The equivalent spring constant is the sum of the individual spring constants.

The formula for the equivalent spring constant is:

k_eq = k_1 + k_2 + ... + k_n

Where:
– k_eq is the equivalent spring constant
– k_1, k_2, …, k_n are the individual spring constants

Once you have the equivalent spring constant, you can use the same formula to calculate the spring potential energy:

U = 1/2 * k_eq * x^2

Where x is the displacement from the equilibrium position of the equivalent spring.

Considerations in Calculating Work and Elastic Potential Energy

When calculating the work and elastic potential energy for a spring, it is important to consider the following:

1. Initial Spring Length: Measure the length of the spring with no added mass. This will be the reference point for calculating the change in spring length.
2. Force and Displacement: The force applied over a distance is the work done, and this work is done in the same direction as the applied force. The relationship can be expressed as: Work = (Force)(Distance) or W = Fx.
3. Average Applied Force: The average applied force over the distance x is (0 + kx)/2 or Fs = ½ kx.

By considering these factors, you can accurately calculate the work done and the elastic potential energy stored in the spring.

Practical Applications of Spring Potential Energy

Spring potential energy has numerous practical applications in various fields, including:

1. Mechanical Systems: Springs are used in a wide range of mechanical systems, such as shock absorbers, car suspensions, and household appliances, to store and release energy as needed.
2. Energy Storage: Springs can be used as a means of storing energy, which can then be released to do work. This is particularly useful in applications where energy needs to be stored and released in a controlled manner, such as in mechanical clocks and some types of toys.
3. Vibration Damping: The energy stored in a spring can be used to dampen vibrations, which is important in applications such as building construction, machinery, and transportation.
4. Oscillatory Systems: Springs are a key component in oscillatory systems, such as pendulums and mass-spring systems, which are used in various scientific and engineering applications, including timekeeping and vibration analysis.

Conclusion

In this comprehensive guide, we have explored the concept of spring potential energy, including the necessary formulas, examples, and practical applications. By understanding the principles of spring potential energy, you can effectively calculate the energy stored in a spring and apply this knowledge to a wide range of physics and engineering problems. Remember to always consider the spring constant, displacement, and other relevant factors when determining the spring potential energy.

References

1. Spring Potential Energy: Overview & Equation – StudySmarter
   https://www.studysmarter.co.uk/explanations/physics/work-energy-and-power/spring-potential-energy/
2. Elastic Potential Energy in a Spring (Hooke’s Law Revisited)
   https://www.ntschools.org/cms/lib/NY19000908/Centricity/Domain/112/Potential%20Energy%20on%20a%20Spring.2%20for%20webpage.pdf
3. Spring Potential Energy: Definition, Equation, Units (w/ Examples)
   https://sciencing.com/spring-potential-energy-definition-equation-units-w-examples-13720807.html