Sliding friction, also known as kinetic friction, is a crucial concept in physics that describes the force that opposes the relative motion between two surfaces in contact. Understanding how to accurately determine sliding friction is essential for various applications, from engineering design to everyday problem-solving. In this comprehensive guide, we will delve into the intricacies of calculating sliding friction, providing you with the necessary tools and techniques to master this fundamental topic.
Understanding the Basics of Sliding Friction
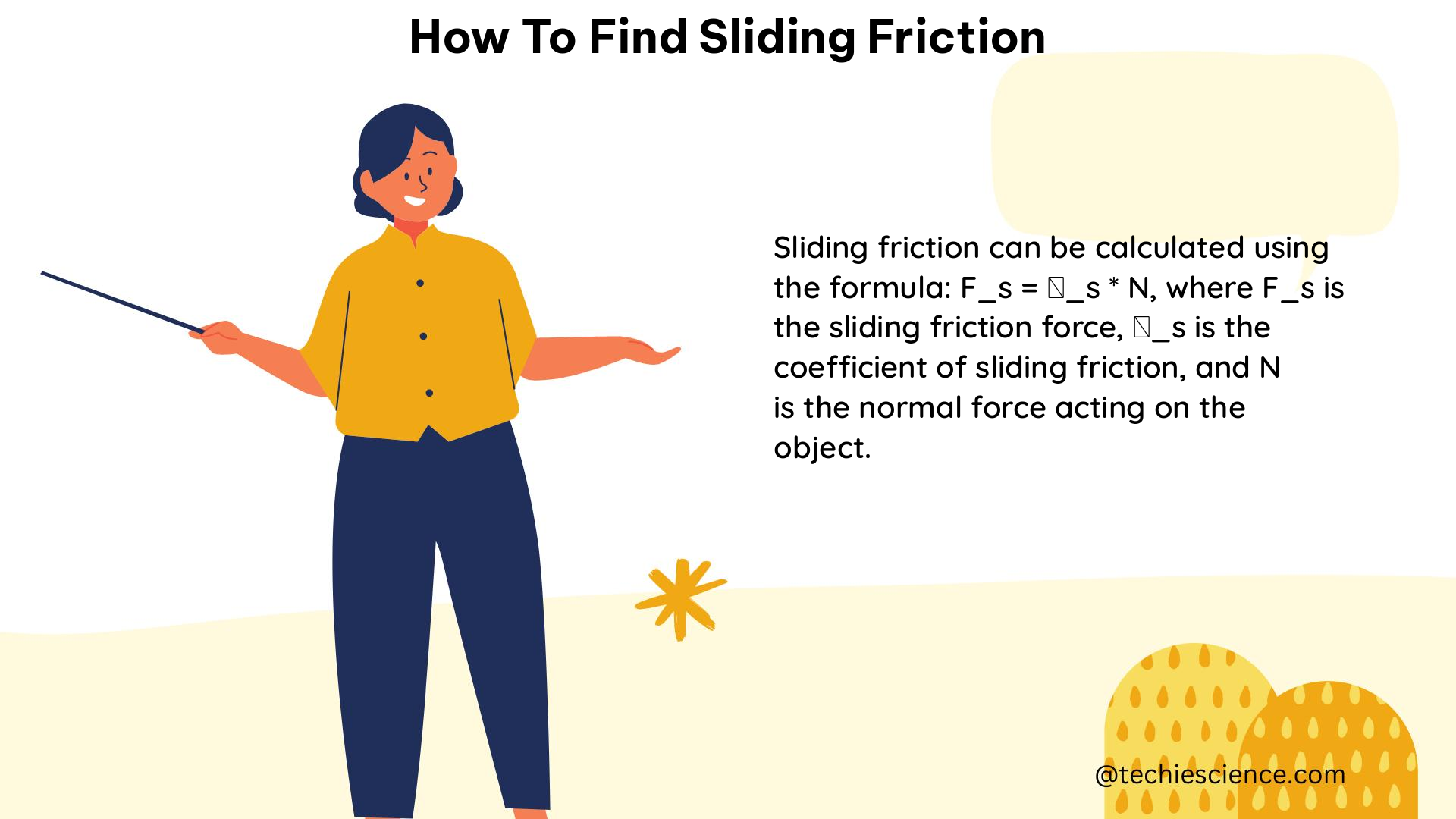
Sliding friction is the force that acts on an object as it slides across a surface. This force is proportional to the normal force, which is the force exerted by the surface on the object perpendicular to the surface. The relationship between sliding friction, normal force, and the coefficient of kinetic friction is expressed by the formula:
fk = μk × N
Where:
– fk is the kinetic (sliding) friction force
– μk is the coefficient of kinetic friction
– N is the normal force
The coefficient of kinetic friction, μk, is a dimensionless quantity that depends on the materials in contact and the surface conditions. It is typically less than the coefficient of static friction, μs, which is the friction force that must be overcome to initiate motion.
Calculating the Normal Force
The normal force, N, is the force exerted by the surface on the object perpendicular to the surface. For an object resting on a flat surface, the normal force is equal to the object’s weight, which can be calculated as:
N = m × g
Where:
– m is the mass of the object
– g is the acceleration due to gravity (9.8 m/s² on Earth)
For example, if an object has a mass of 10 kg and is resting on a flat surface, the normal force would be:
N = 10 kg × 9.8 m/s² = 98 N
Determining the Coefficient of Kinetic Friction
The coefficient of kinetic friction, μk, is a crucial parameter in the calculation of sliding friction. This value depends on the materials in contact and the surface conditions, and it must be determined experimentally or obtained from reference tables.
Here are some typical values of the coefficient of kinetic friction for common material combinations:
| Material Combination | Coefficient of Kinetic Friction (μk) |
|---|---|
| Steel on steel | 0.10 – 0.15 |
| Aluminum on steel | 0.45 – 0.61 |
| Rubber on concrete | 0.50 – 0.80 |
| Wood on wood | 0.20 – 0.50 |
| Teflon on Teflon | 0.04 – 0.10 |
It’s important to note that the coefficient of kinetic friction can vary depending on factors such as surface roughness, temperature, and the presence of lubricants or contaminants.
Calculating Sliding Friction Force
Once you have determined the normal force and the coefficient of kinetic friction, you can calculate the sliding friction force using the formula:
fk = μk × N
For example, if an object with a mass of 10 kg is resting on a surface with a coefficient of kinetic friction of 0.2, the sliding friction force would be:
fk = 0.2 × (10 kg × 9.8 m/s²) = 19.6 N
Calculating Acceleration with Sliding Friction
When an external force, Fa, is applied to an object on a surface with sliding friction, the total force on the object is the difference between the applied force and the sliding friction force:
F = Fa - fk
Rearranging the equation, we can find the acceleration of the object:
a = (Fa - fk) / m
Where:
– a is the acceleration of the object
– Fa is the applied force
– fk is the sliding friction force
– m is the mass of the object
For example, if an object with a mass of 5 kg is subjected to an applied force of 20 N and the sliding friction force is 10 N, the acceleration of the object would be:
a = (20 N - 10 N) / 5 kg = 2 m/s²
Practical Applications and Examples
Sliding friction is a fundamental concept in physics with numerous practical applications. Here are a few examples:
-
Braking Systems: Understanding sliding friction is crucial in the design of braking systems for vehicles. The coefficient of kinetic friction between the brake pads and the brake discs or drums determines the braking force and the vehicle’s stopping distance.
-
Mechanical Devices: Sliding friction plays a crucial role in the design and operation of various mechanical devices, such as bearings, gears, and pulleys. Accurate calculation of sliding friction is necessary to ensure efficient and reliable performance.
-
Inclined Planes: When an object is placed on an inclined plane, the sliding friction force acts parallel to the surface, opposing the motion of the object. Analyzing the sliding friction force is essential in determining the object’s acceleration or the minimum angle required for the object to start sliding.
-
Robotics and Automation: In the field of robotics and automation, understanding and controlling sliding friction is crucial for the precise movement and manipulation of objects by robotic systems.
-
Sports and Recreation: Sliding friction is an important factor in various sports and recreational activities, such as skiing, snowboarding, and ice skating. Analyzing the sliding friction can help athletes and designers optimize equipment and techniques for improved performance.
Conclusion
Mastering the concept of sliding friction is a crucial step in understanding and applying physics principles in various real-world scenarios. By following the comprehensive approach outlined in this guide, you can confidently calculate sliding friction, determine the normal force, and analyze the effects of sliding friction on the motion of objects. This knowledge will empower you to tackle a wide range of physics problems and design more efficient and reliable systems.
References
- Serway, R. A., & Jewett, J. W. (2018). Physics for Scientists and Engineers with Modern Physics (10th ed.). Cengage Learning.
- Halliday, D., Resnick, R., & Walker, J. (2013). Fundamentals of Physics (10th ed.). Wiley.
- Hibbeler, R. C. (2018). Engineering Mechanics: Statics (14th ed.). Pearson.
- Giancoli, D. C. (2014). Physics for Scientists and Engineers with Modern Physics (4th ed.). Pearson.
- Tipler, P. A., & Mosca, G. (2008). Physics for Scientists and Engineers (6th ed.). W. H. Freeman.
Hi ….I am Abhishek Khambhata, have pursued B. Tech in Mechanical Engineering. Throughout four years of my engineering, I have designed and flown unmanned aerial vehicles. My forte is fluid mechanics and thermal engineering. My fourth-year project was based on the performance enhancement of unmanned aerial vehicles using solar technology. I would like to connect with like-minded people.