How to Find Reynolds Number Without Velocity: A Comprehensive Guide

Summary

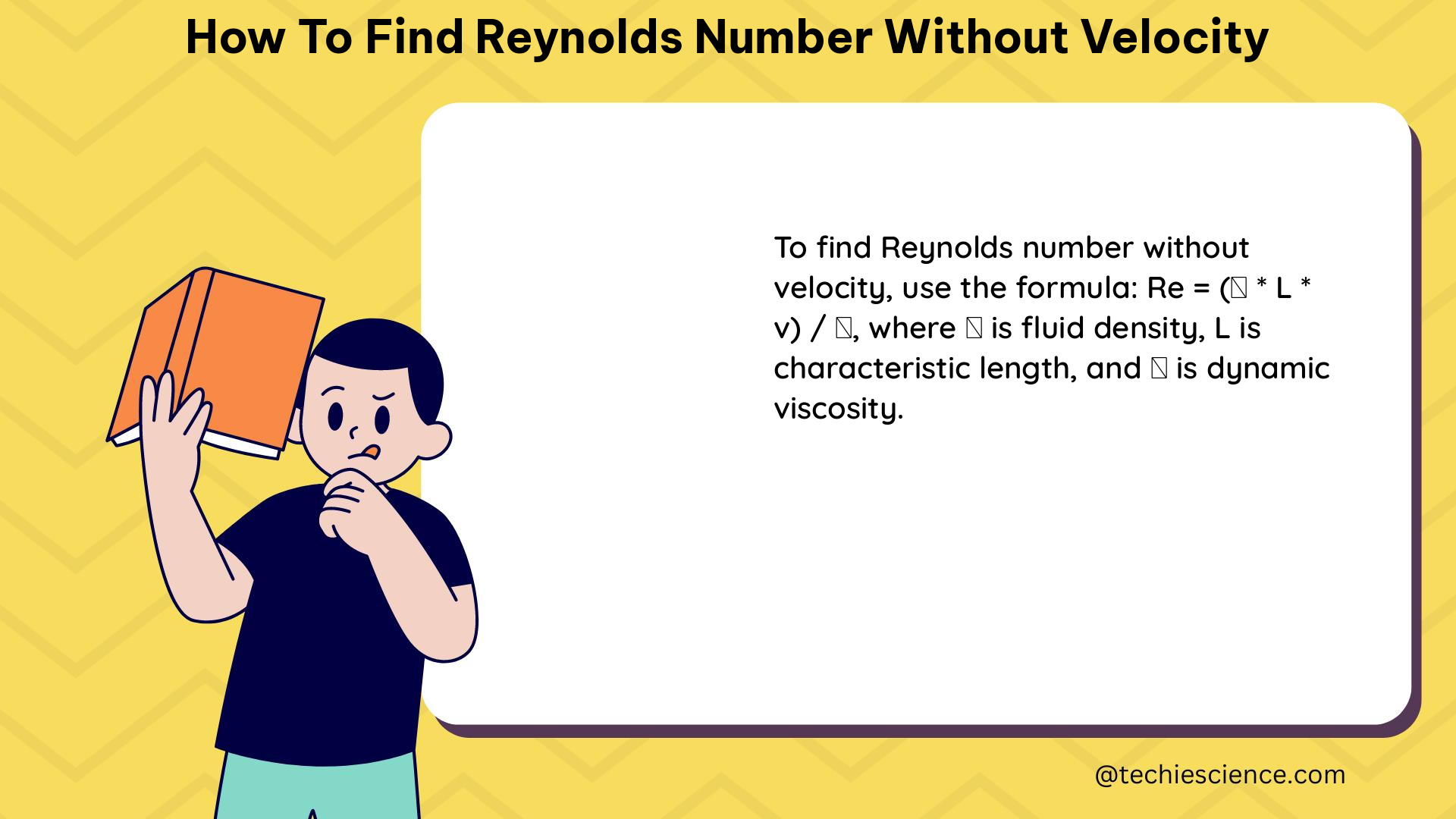
To determine the Reynolds number (Re) without knowing the velocity, you can use the formula Re = ρL/μ, where ρ is the fluid density, L is the characteristic length, and μ is the dynamic viscosity. This formula is derived from the Navier-Stokes equations, which describe the motion of fluid substances. By measuring the fluid’s density, dynamic viscosity, and characteristic length, you can calculate the Reynolds number and understand the flow regime of the fluid, whether it is laminar, transitional, or turbulent.

Understanding the Reynolds Number Formula

The Reynolds number is a dimensionless quantity that represents the ratio of inertial forces to viscous forces within a fluid flow. It is a crucial parameter in fluid mechanics and is used to predict flow patterns, flow regimes, and the likelihood of flow instabilities.

The formula for the Reynolds number is:

Re = ρvL/μ

Where:
– ρ (rho) is the fluid density, measured in kg/m³ or slugs/ft³
– v is the fluid velocity, measured in m/s or ft/s
– L is the characteristic length, measured in meters or feet
– μ (mu) is the dynamic viscosity of the fluid, measured in Pa·s or lb·s/ft²

However, in situations where the fluid velocity is not known, you can rearrange the formula to solve for the Reynolds number without velocity:

Re = ρL/μ

This formula is derived from the Navier-Stokes equations, which describe the motion of fluid substances. By using this formula, you can calculate the Reynolds number based on the fluid’s density, dynamic viscosity, and characteristic length.

Measuring Fluid Density (ρ)

The fluid density (ρ) is the mass of the fluid per unit volume. It can be measured in various units, such as kilograms per cubic meter (kg/m³) or slugs per cubic foot (slugs/ft³).

To measure the fluid density, you can use the following methods:

1. Direct Measurement: Weigh a known volume of the fluid and divide the mass by the volume to obtain the density.
2. Pycnometer Method: Use a pycnometer, which is a calibrated glass vessel with a known volume, to measure the mass of the fluid and calculate the density.
3. Hydrometer Method: Use a hydrometer, which is a device that measures the relative density of a liquid compared to water.
4. Calculation from Composition: If the chemical composition of the fluid is known, you can calculate the density using the molar masses and mole fractions of the components.

The accuracy of the density measurement is crucial for the correct calculation of the Reynolds number.

Measuring Dynamic Viscosity (μ)

The dynamic viscosity (μ) is a measure of the fluid’s resistance to flow. It can be measured in various units, such as pascal-seconds (Pa·s) or pounds-seconds per square foot (lb·s/ft²).

To measure the dynamic viscosity, you can use the following methods:

1. Capillary Viscometer: Measure the time it takes for a known volume of fluid to flow through a calibrated capillary tube under the influence of gravity.
2. Rotational Viscometer: Measure the torque required to rotate a spindle or cylinder immersed in the fluid at a known angular velocity.
3. Falling Ball Viscometer: Measure the time it takes for a small ball to fall through a known distance in the fluid.
4. Oscillating Viscometer: Measure the damping of an oscillating body immersed in the fluid.

The choice of viscosity measurement method depends on the fluid properties, the required accuracy, and the available equipment.

Determining the Characteristic Length (L)

The characteristic length (L) is a measure of the size of the fluid system. It can be the diameter of a pipe, the length of a plate, or any other relevant length scale.

The choice of the characteristic length depends on the specific fluid flow problem you are studying. For example:

• For flow through a circular pipe, the characteristic length is the pipe diameter.
• For flow over a flat plate, the characteristic length is the length of the plate.
• For flow around a sphere, the characteristic length is the diameter of the sphere.
• For flow around an airfoil, the characteristic length is the chord length of the airfoil.

Accurately measuring the characteristic length is crucial for the correct calculation of the Reynolds number.

Calculating the Reynolds Number

Once you have measured the fluid density (ρ), dynamic viscosity (μ), and characteristic length (L), you can calculate the Reynolds number using the formula:

Re = ρL/μ

For example, let’s consider the flow of air over a flat plate with the following parameters:

• Fluid: Air
• Density (ρ): 1.225 kg/m³
• Dynamic Viscosity (μ): 1.81 × 10⁻⁵ Pa·s
• Characteristic Length (L): 1 m

Plugging these values into the formula:

Re = ρL/μ
Re = (1.225 kg/m³) × (1 m) / (1.81 × 10⁻⁵ Pa·s)
Re = 67,700

This Reynolds number indicates that the flow over the flat plate is in the turbulent regime, as it is greater than the critical Reynolds number of approximately 4,000.

Interpreting the Reynolds Number

The Reynolds number is a dimensionless quantity that describes the flow regime of the fluid. The flow regime can be classified as:

1. Laminar Flow: Re < 2,300
2. The fluid moves in smooth, predictable paths.

3. Viscous forces dominate, and the flow is stable.

4. Transitional Flow: 2,300 ≤ Re ≤ 4,000

5. The flow may switch between laminar and turbulent regimes.

6. Both viscous and inertial forces are significant.

7. Turbulent Flow: Re > 4,000

8. The fluid moves in complex, unpredictable paths.
9. Inertial forces dominate, and the flow is unstable.

Understanding the flow regime is crucial for predicting the behavior of the fluid, such as pressure drop, heat transfer, and the likelihood of flow instabilities.

Practical Applications and Examples

The ability to calculate the Reynolds number without velocity has numerous practical applications in various fields, such as:

1. Fluid Mechanics: Analyzing the flow of fluids (liquids and gases) through pipes, over surfaces, and around objects.
2. Heat Transfer: Determining the heat transfer characteristics in heat exchangers, boilers, and other thermal systems.
3. Aerodynamics: Studying the flow of air around aircraft, vehicles, and other structures.
4. Hydraulics: Analyzing the flow of water in rivers, canals, and other hydraulic systems.
5. Biomedical Engineering: Investigating the flow of blood in the cardiovascular system.
6. Chemical Engineering: Designing and optimizing chemical processes involving fluid flow.

Here are a few examples of how the Reynolds number without velocity can be used:

Example 1: Flow of air over a wing
– Fluid: Air
– Density (ρ): 1.225 kg/m³
– Dynamic Viscosity (μ): 1.81 × 10⁻⁵ Pa·s
– Characteristic Length (L): 1 m (chord length of the wing)
– Reynolds number: Re = ρL/μ = 67,700 (turbulent flow)

Example 2: Flow of water through a pipe
– Fluid: Water
– Density (ρ): 1,000 kg/m³
– Dynamic Viscosity (μ): 1.002 × 10⁻³ Pa·s
– Characteristic Length (L): 0.1 m (pipe diameter)
– Reynolds number: Re = ρL/μ = 10,000 (transitional flow)

Example 3: Flow of blood in a blood vessel
– Fluid: Blood
– Density (ρ): 1,060 kg/m³
– Dynamic Viscosity (μ): 3.5 × 10⁻³ Pa·s
– Characteristic Length (L): 0.005 m (blood vessel diameter)
– Reynolds number: Re = ρL/μ = 1.5 (laminar flow)

These examples demonstrate how the Reynolds number without velocity can be used to analyze and understand the flow regimes in various fluid systems.

Conclusion

In summary, to find the Reynolds number without velocity, you can use the formula Re = ρL/μ, where ρ is the fluid density, L is the characteristic length, and μ is the dynamic viscosity. By accurately measuring these parameters, you can calculate the Reynolds number and determine the flow regime of the fluid, whether it is laminar, transitional, or turbulent. This knowledge is crucial for understanding and predicting the behavior of fluid systems in a wide range of applications, from fluid mechanics and heat transfer to aerodynamics and biomedical engineering.

References

1. Simscale: What is the Reynolds Number? A Complete Guide
2. Reddit: How to find Reynolds Number without velocity?
3. The Engineering ToolBox: Reynolds Number
4. ScienceDirect: Particle Reynolds Number
5. Wikipedia: Reynolds Number