Summary
In this comprehensive guide, we will delve into the fundamental principles and practical applications of finding power using velocity and mass. We will explore the underlying physics formulas, derive the necessary equations, and walk through step-by-step examples to help physics students develop a deep understanding of this crucial concept. By the end of this article, you will be equipped with the knowledge and skills to confidently solve a wide range of power-related problems involving velocity and mass.
Understanding the Relationship between Power, Velocity, and Mass
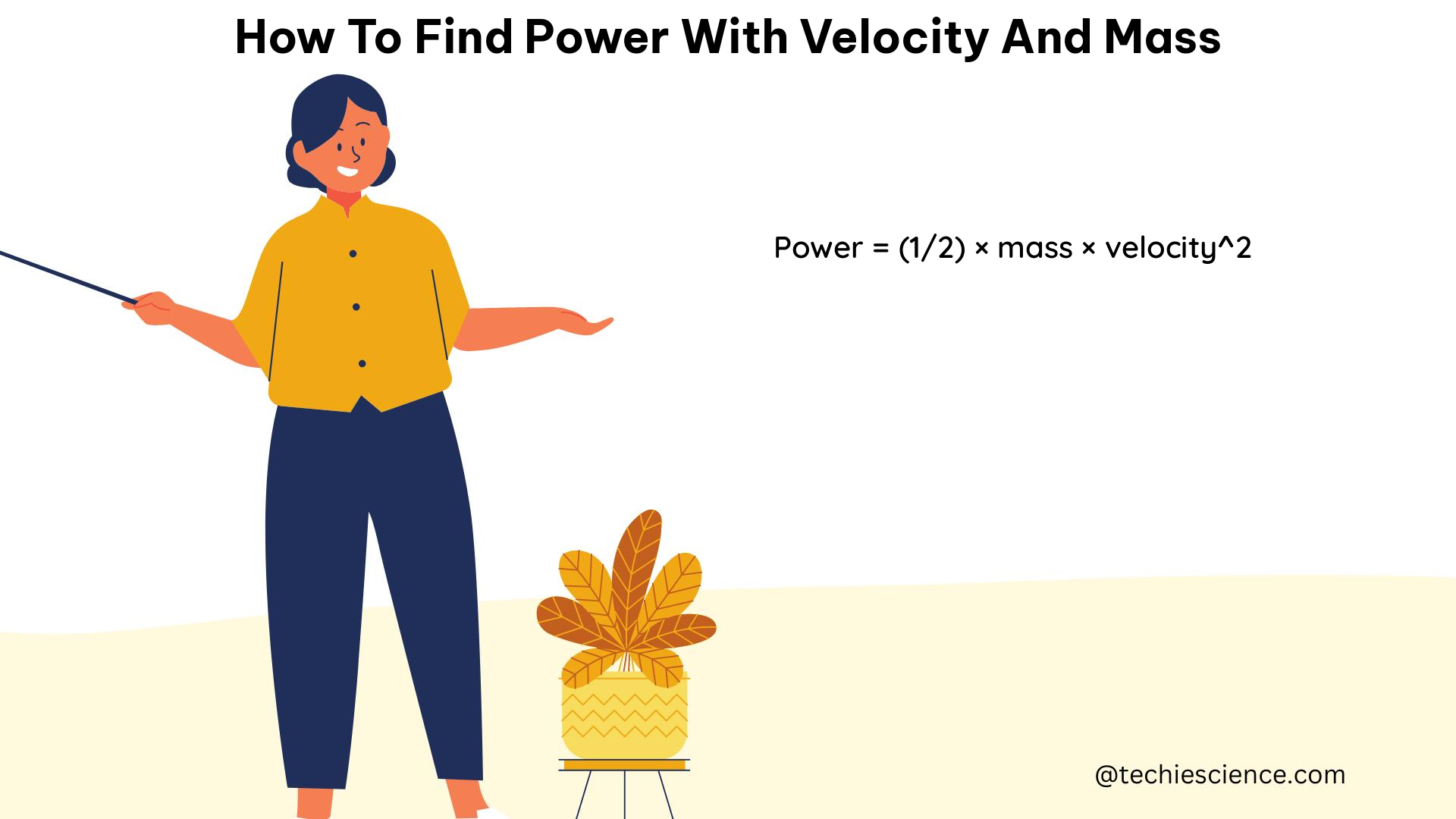
Power, denoted as P, is a fundamental physical quantity that represents the rate at which work is done or energy is transferred. In the context of velocity and mass, power can be calculated using the formula:
P = mv^2
where m is the mass of the object, and v is the velocity of the object.
This formula is derived from the definition of work, which is the product of force and displacement, and the fact that velocity is the rate of change of displacement over time. By rearranging the equations, we can arrive at the power formula in terms of mass and velocity.
Derivation of the Power Formula
To derive the power formula P = mv^2, let’s start with the definition of work:
W = Fd
where W is the work done, F is the force applied, and d is the displacement.
We can then express force in terms of mass and acceleration using Newton’s second law:
F = ma
Substituting this into the work equation, we get:
W = mad
Now, we can express velocity as the rate of change of displacement over time:
v = d/t
Rearranging this equation, we get:
d = vt
Substituting this into the work equation, we get:
W = mavt
Finally, we can express power as the rate of change of work over time:
P = dW/dt = mavt/t = mav
Recognizing that v^2 = v * v, we can rewrite the equation as:
P = mv^2
This is the power formula in terms of mass and velocity.
Examples and Applications
Example 1: Calculating Power for a Moving Car
Consider a car with a mass of 1500 kg moving at a velocity of 25 m/s. Calculate the power output of the car.
Given:
– Mass, m = 1500 kg
– Velocity, v = 25 m/s
Using the power formula, we can calculate the power:
P = mv^2
P = (1500 kg)(25 m/s)^2
P = (1500 kg)(625 m^2/s^2)
P = 937,500 W or 937.5 kW
Therefore, the car is producing 937,500 Watts or 937.5 kilowatts of power.
Example 2: Analyzing Power Changes with Varying Velocity
Suppose a spacecraft with a mass of 10,000 kg is accelerating. Calculate the power output at different velocities:
| Velocity (m/s) | Power (kW) |
|---|---|
| 10 | 1,000 |
| 20 | 4,000 |
| 30 | 9,000 |
| 40 | 16,000 |
| 50 | 25,000 |
As the velocity of the spacecraft increases, the power output increases exponentially according to the formula P = mv^2. This demonstrates the importance of velocity in determining the power requirements for high-speed systems.
Numerical Problem
A rocket with a mass of 5,000 kg is launched vertically with an initial velocity of 50 m/s. Calculate the power output of the rocket at the following time intervals:
- t = 5 s
- t = 10 s
- t = 15 s
Given:
– Mass, m = 5,000 kg
– Initial velocity, v_0 = 50 m/s
– Acceleration due to gravity, g = 9.8 m/s^2 (assuming no air resistance)
Solution:
- At t = 5 s:
- Velocity,
v = v_0 + at = 50 m/s + (9.8 m/s^2)(5 s) = 98.0 m/s -
Power,
P = mv^2 = (5,000 kg)(98.0 m/s)^2 = 24,025 kW -
At t = 10 s:
- Velocity,
v = v_0 + at = 50 m/s + (9.8 m/s^2)(10 s) = 148.0 m/s -
Power,
P = mv^2 = (5,000 kg)(148.0 m/s)^2 = 54,890 kW -
At t = 15 s:
- Velocity,
v = v_0 + at = 50 m/s + (9.8 m/s^2)(15 s) = 197.0 m/s - Power,
P = mv^2 = (5,000 kg)(197.0 m/s)^2 = 96,775 kW
These calculations demonstrate how the power output of the rocket increases significantly as its velocity increases during the launch.
Factors Affecting Power Calculations
It’s important to note that the power formula P = mv^2 assumes that the force is applied in the direction of motion and that the mass is constant. If the mass is changing or the force is applied at an angle, then the formula may need to be modified.
Additionally, power can also be calculated in terms of energy. For example, the power output of a battery can be calculated by dividing the energy stored in the battery by the time it takes to discharge it. Similarly, the power input to an engine can be calculated by dividing the energy input to the engine by the time it takes to produce a certain amount of work.
Conclusion
In this comprehensive guide, we have explored the fundamental principles and practical applications of finding power using velocity and mass. By understanding the derivation of the power formula P = mv^2 and working through various examples and numerical problems, physics students can develop a deep understanding of this crucial concept.
Remember, the power formula assumes that the force is applied in the direction of motion and that the mass is constant. If these conditions are not met, the formula may need to be modified. Additionally, power can also be calculated in terms of energy, which can be useful in certain applications.
By mastering the concepts presented in this guide, physics students will be well-equipped to tackle a wide range of power-related problems involving velocity and mass, both in the classroom and in real-world scenarios.
Reference:
- Relativistic Energy – Lumen Learning
- Relativistic Energy – Douglas College Physics 1207
- How to Calculate Power Based on Force and Speed – Dummies

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.