Mastering the Art of Finding Power with Resistance and Current

In the realm of electrical circuits, understanding the relationship between power, resistance, and current is crucial for engineers, physicists, and anyone working with electrical systems. This comprehensive guide will delve into the intricacies of calculating power using the formulas P = I^2R and P = V^2/R, providing you with a deep understanding of the underlying principles and practical applications.

Ohm’s Law and the Power Formula

The foundation of our exploration lies in Ohm’s Law, which states that the current (I) flowing through a conductor is directly proportional to the voltage (V) applied across it and inversely proportional to the resistance (R) of the conductor. This relationship can be expressed mathematically as:

V = IR

From this fundamental equation, we can derive the power formula, which describes the relationship between power (P), current (I), and resistance (R):

P = IV

Substituting Ohm’s Law into the power formula, we can arrive at the two formulas for calculating power:

1. P = I^2R: This formula allows us to calculate power when the current (I) and resistance (R) are known.
2. P = V^2/R: This formula allows us to calculate power when the voltage (V) and resistance (R) are known.

Calculating Power Using P = I^2R

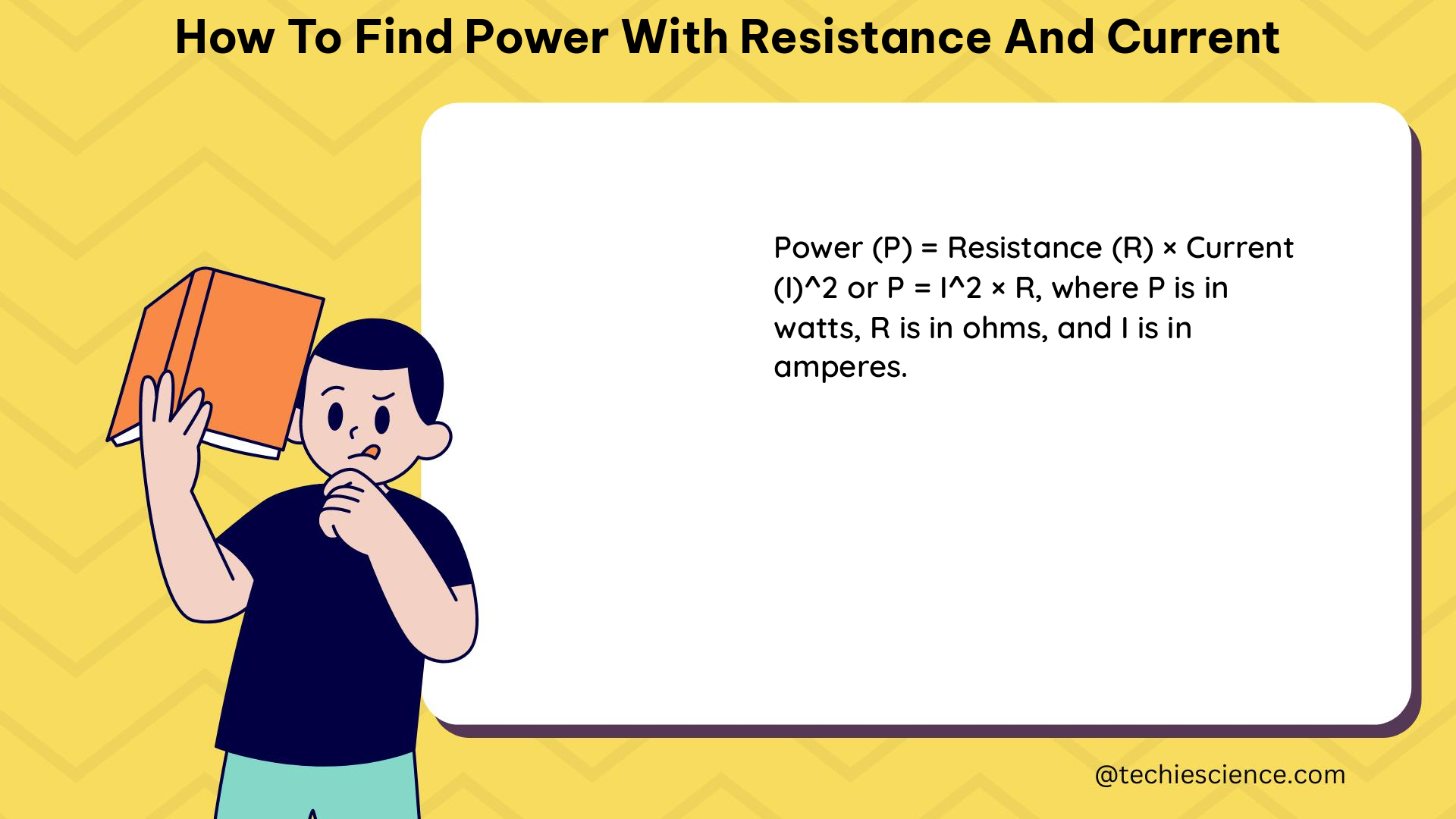
To use the formula P = I^2R, you need to know the current (I) flowing through the circuit and the resistance (R) of the circuit. The steps to calculate power are as follows:

1. Identify the current (I) and resistance (R) values in the circuit.
2. Square the current value (I^2).
3. Multiply the squared current value (I^2) by the resistance (R).
4. The result is the power (P) in the circuit, expressed in watts (W).

Example 1: A circuit has a current of 5 amps and a resistance of 10 ohms. Calculate the power in the circuit.

1. Current (I) = 5 amps
2. Resistance (R) = 10 ohms
3. P = I^2R
   P = (5 A)^2 × 10 Ω
   P = 250 W

Therefore, the power in the circuit is 250 watts.

Calculating Power Using P = V^2/R

To use the formula P = V^2/R, you need to know the voltage (V) applied across the circuit and the resistance (R) of the circuit. The steps to calculate power are as follows:

1. Identify the voltage (V) and resistance (R) values in the circuit.
2. Square the voltage value (V^2).
3. Divide the squared voltage value (V^2) by the resistance (R).
4. The result is the power (P) in the circuit, expressed in watts (W).

Example 2: A circuit has a voltage of 120 volts and a resistance of 40 ohms. Calculate the power in the circuit.

1. Voltage (V) = 120 volts
2. Resistance (R) = 40 ohms
3. P = V^2/R
   P = (120 V)^2 / 40 Ω
   P = 360 W

Therefore, the power in the circuit is 360 watts.

Limitations and Considerations

It’s important to note that the formulas P = I^2R and P = V^2/R are applicable only to resistive circuits, where the resistance remains constant and does not change with the current or voltage. In reactive circuits, where capacitors or inductors are present, the power calculation becomes more complex and requires knowledge of the phase relationship between the current and voltage.

Additionally, when working with power calculations, it’s crucial to use the appropriate units. The unit of power is the watt (W), which is equal to one joule per second (J/s). In some applications, other units of power may be used, such as horsepower (hp) or kilowatts (kW), and it’s essential to be aware of these conversions.

Advanced Concepts and Applications

Beyond the basic formulas, there are several advanced concepts and applications related to finding power with resistance and current:

1. Power Dissipation in Resistors: When current flows through a resistor, the resistor dissipates power in the form of heat. The power dissipated in a resistor can be calculated using the formula P = I^2R.

2. Electrical Efficiency: The efficiency of an electrical system is the ratio of the output power to the input power. Calculating the efficiency is crucial for optimizing the performance of electrical devices and systems.

3. Power Transmission and Distribution: In the context of power transmission and distribution, the formulas P = I^2R and P = V^2/R are used to analyze the power losses and optimize the design of electrical grids and transmission lines.

4. Electric Heating and Heating Elements: The power dissipated in a resistive heating element can be calculated using the formulas, and this knowledge is essential for the design and operation of electric heaters, ovens, and other heating appliances.

5. Battery and Energy Storage Systems: The power formulas are used to analyze the performance and efficiency of battery-powered devices and energy storage systems, such as in electric vehicles and renewable energy applications.

6. Electrical Safety and Circuit Protection: Understanding the power dissipation in electrical circuits is crucial for designing effective circuit protection devices, such as fuses and circuit breakers, to prevent overloads and potential hazards.

Practical Examples and Numerical Problems

To solidify your understanding, let’s explore some practical examples and numerical problems related to finding power with resistance and current:

Example 3: A resistor with a resistance of 100 ohms is connected to a 12-volt power source. Calculate the power dissipated in the resistor.

1. Voltage (V) = 12 volts
2. Resistance (R) = 100 ohms
3. P = V^2/R
   P = (12 V)^2 / 100 Ω
   P = 1.44 W

Therefore, the power dissipated in the resistor is 1.44 watts.

Example 4: A motor in an electric vehicle has a current of 20 amps and a resistance of 0.5 ohms. Calculate the power consumed by the motor.

1. Current (I) = 20 amps
2. Resistance (R) = 0.5 ohms
3. P = I^2R
   P = (20 A)^2 × 0.5 Ω
   P = 200 W

Therefore, the power consumed by the motor is 200 watts.

Example 5: A heating element in an electric oven has a resistance of 20 ohms and is connected to a 240-volt power source. Calculate the power dissipated by the heating element.

1. Voltage (V) = 240 volts
2. Resistance (R) = 20 ohms
3. P = V^2/R
   P = (240 V)^2 / 20 Ω
   P = 2,880 W

Therefore, the power dissipated by the heating element is 2,880 watts.

These examples demonstrate the practical application of the power formulas in various electrical systems and devices. By working through these problems, you can develop a deeper understanding of how to calculate power using resistance and current.

Conclusion

In this comprehensive guide, we have explored the fundamental principles and practical applications of finding power with resistance and current. By mastering the formulas P = I^2R and P = V^2/R, you now have the tools to analyze and calculate power in a wide range of electrical circuits and systems.

Remember to always consider the limitations of these formulas, such as their applicability to resistive circuits, and be mindful of the appropriate units when performing power calculations. Additionally, explore the advanced concepts and applications to deepen your understanding and apply these principles in real-world scenarios.

With the knowledge and skills gained from this guide, you are now equipped to tackle power-related challenges in your electrical engineering, physics, or any other field that involves electrical circuits and systems.

Reference:

1. Ohm’s Law and Electrical Power Calculations
2. Electrical Power and Energy
3. Power in Electrical Circuits