How to Find Net Acceleration in Circular Motion

In circular motion, the net acceleration is the vector sum of two types of acceleration: tangential acceleration and radial acceleration. Understanding the concepts and formulas behind these two components is crucial to accurately determining the net acceleration in circular motion.

Tangential Acceleration

Tangential acceleration is the acceleration in the direction of motion, perpendicular to the radius of the circular path. It is given by the derivative of the velocity vector with respect to time, or dv/dt. Tangential acceleration represents the change in the speed of the object as it moves along the circular path.

Radial Acceleration (Centripetal Acceleration)

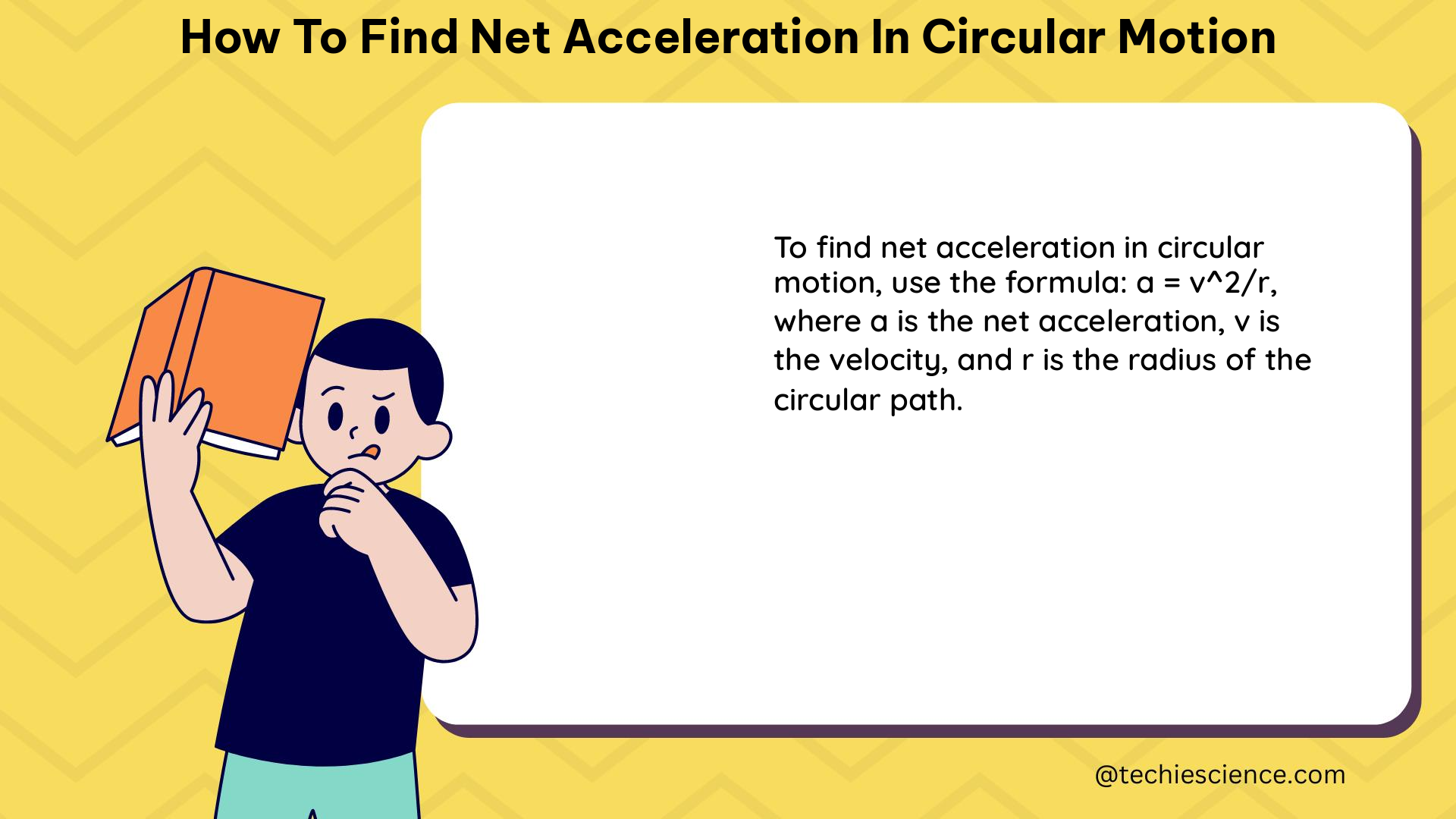
Radial acceleration, also known as centripetal acceleration, is the acceleration directed towards the center of the circular path. It is given by the formula a_c = v^2/r, where v is the velocity of the object and r is the radius of the circular path. Centripetal acceleration is responsible for the object’s change in direction, keeping it on the circular trajectory.

Net Acceleration

The net acceleration in circular motion is the vector sum of the tangential and radial accelerations, given by the formula:

a_net = sqrt(a_t^2 + a_c^2)

where a_t is the tangential acceleration and a_c is the radial (centripetal) acceleration.

Example 1: Fairground Ride

Let’s consider a fairground ride with a radius of 8.00 m. We want to find the number of revolutions per minute (RPM) that would result in a centripetal acceleration of 1.50 times the acceleration due to gravity (g = 9.8 m/s^2).

1. Calculate the centripetal acceleration:
   a_c = 1.50 * g = 1.50 * 9.8 m/s^2 = 14.7 m/s^2

2. Use the formula a_c = v^2/r to solve for the velocity:
   v = sqrt(a_c * r) = sqrt((14.7 m/s^2) * (8.00 m)) = 14.14 m/s

3. Calculate the number of revolutions per minute (RPM) using the formula:
   RPM = (v / (2 * π * r)) * 60 = (14.14 m/s / (2 * π * 8.00 m)) * 60 = 6.42 RPM

Example 2: Runner on a Curved Track

Consider a runner in a 200 m dash who must run around the end of a track with a radius of curvature of 30 m. The runner completes the race in 23.2 seconds and runs at a constant speed.

1. Calculate the runner’s velocity:
   v = distance / time = 200 m / 23.2 s = 8.62 m/s

2. Use the formula a_c = v^2/r to find the centripetal acceleration:
   a_c = (8.62 m/s)^2 / 30 m = 2.48 m/s^2

In this case, the net acceleration is equal to the centripetal acceleration, as the tangential acceleration is zero due to the constant speed.

Additional Considerations

• The net acceleration in circular motion can be used to determine the force acting on the object, using Newton’s second law: F = m * a_net.
• The direction of the net acceleration is always towards the center of the circular path, as it is the vector sum of the tangential and radial accelerations.
• In some cases, the tangential acceleration may be non-zero, such as when the object’s speed is changing. In such cases, the net acceleration will have both tangential and radial components.
• The formulas and examples provided here assume uniform circular motion, where the speed and radius of the circular path remain constant.

Reference:

1. Net acceleration in circular motion – Physics Stack Exchange: https://physics.stackexchange.com/questions/427293/net-acceleration-in-circular-motion
2. Centripetal Acceleration | Physics – Lumen Learning: https://courses.lumenlearning.com/suny-physics/chapter/6-2-centripetal-acceleration/
3. 6.2 Uniform Circular Motion – acceleration – Texas Gateway: https://www.texasgateway.org/resource/62-uniform-circular-motion
4. 6.2 Uniform Circular Motion – Physics | OpenStax: https://openstax.org/books/physics/pages/6-2-uniform-circular-motion
5. Net Acceleration of a Car (Circular Motion) #11 – YouTube: https://www.youtube.com/watch?v=iNUZZq0DOAQ