Mastering the Art of Finding Momentum from Kinetic Energy

Kinetic energy and momentum are two fundamental concepts in classical mechanics, and understanding the relationship between them is crucial for solving a wide range of physics problems. In this comprehensive guide, we will delve into the intricacies of finding momentum from kinetic energy, providing you with a step-by-step approach and a wealth of technical details to help you become a true master of this topic.

The Kinetic Energy-Momentum Connection

The connection between kinetic energy and momentum is rooted in the fundamental equations of motion. Kinetic energy, denoted as KE, is defined as the energy an object possesses due to its motion, and it is given by the formula:

KE = 1/2 * m * v^2

where m is the mass of the object and v is its velocity.

On the other hand, momentum, denoted as p, is a vector quantity that represents the product of an object’s mass and its velocity:

p = m * v

The key to finding momentum from kinetic energy lies in the relationship between these two quantities. By rearranging the kinetic energy formula, we can express the velocity in terms of the kinetic energy and the mass:

v = sqrt(2 * KE / m)

Once we have the velocity, we can simply plug it into the momentum formula to obtain the object’s momentum:

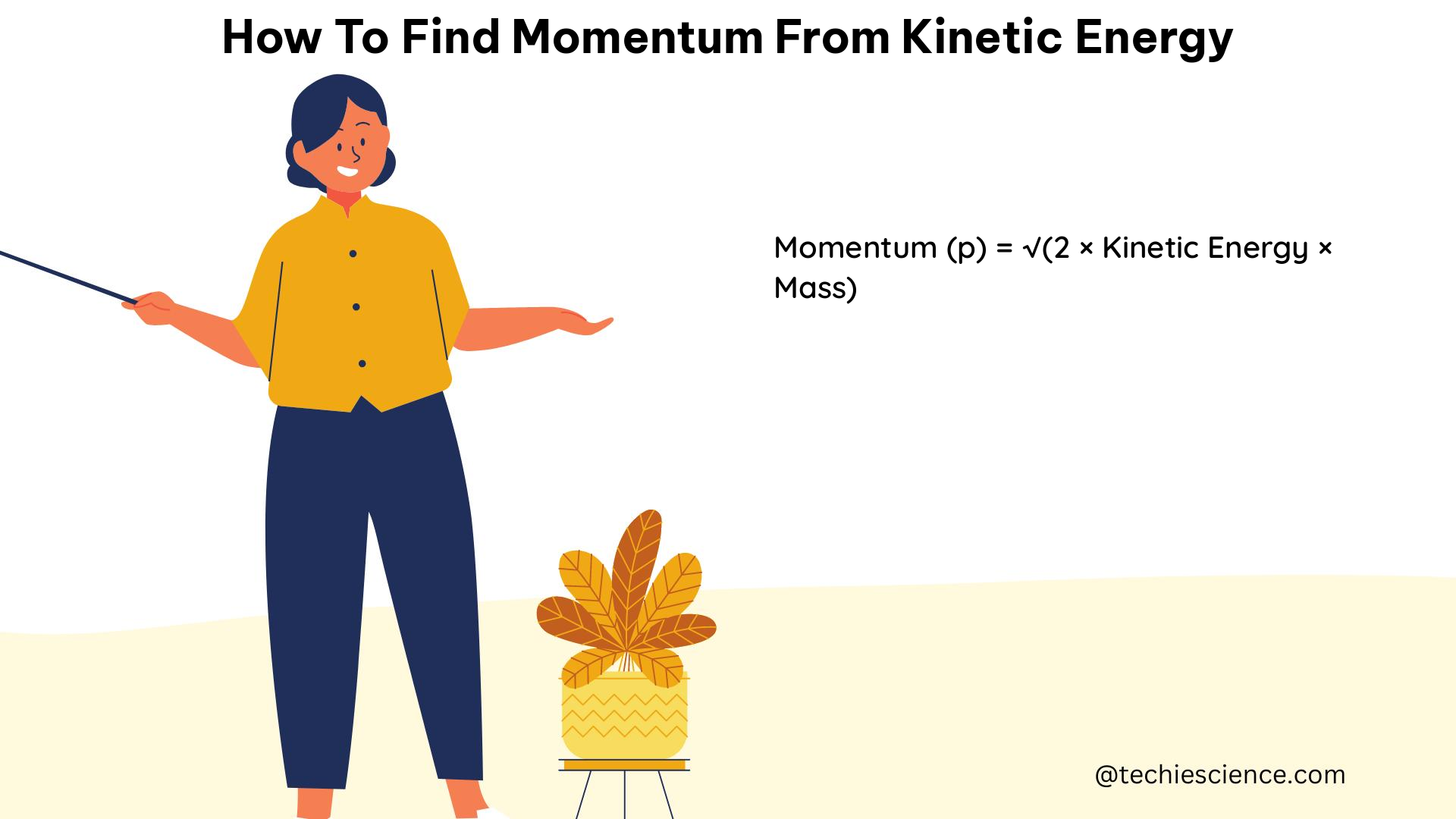
p = m * v = m * sqrt(2 * KE / m) = sqrt(2 * m * KE)

Practical Examples and Numerical Problems

Let’s consider a few practical examples to solidify our understanding of finding momentum from kinetic energy.

Example 1: A 5 kg Object with 100 J of Kinetic Energy

Given:
– Mass, m = 5 kg
– Kinetic Energy, KE = 100 J

Step 1: Calculate the velocity using the kinetic energy formula.

v = sqrt(2 * KE / m)
v = sqrt(2 * 100 J / 5 kg)
v = 4 m/s

Step 2: Calculate the momentum using the momentum formula.

p = m * v
p = 5 kg * 4 m/s
p = 20 kg * m/s

Therefore, the momentum of the 5 kg object with 100 J of kinetic energy is 20 kg * m/s.

Example 2: A 10 kg Object with 400 J of Kinetic Energy

Given:
– Mass, m = 10 kg
– Kinetic Energy, KE = 400 J

Step 1: Calculate the velocity using the kinetic energy formula.

v = sqrt(2 * KE / m)
v = sqrt(2 * 400 J / 10 kg)
v = 8 m/s

Step 2: Calculate the momentum using the momentum formula.

p = m * v
p = 10 kg * 8 m/s
p = 80 kg * m/s

Therefore, the momentum of the 10 kg object with 400 J of kinetic energy is 80 kg * m/s.

Numerical Problems

1. An object with a mass of 2 kg has a kinetic energy of 50 J. Find the object’s momentum.
2. A 3 kg object has a momentum of 12 kg * m/s. Calculate the object’s kinetic energy.
3. A 500 g object is moving with a velocity of 20 m/s. Determine the object’s kinetic energy and momentum.

Graphical Representation and Relationships

To further enhance your understanding, let’s explore the graphical representation of the relationship between kinetic energy and momentum.

Kinetic Energy vs. Velocity Graph

The kinetic energy of an object is proportional to the square of its velocity, as shown in the graph below. This relationship is evident from the kinetic energy formula, KE = 1/2 * m * v^2.

Momentum vs. Velocity Graph

In contrast, the momentum of an object is linearly proportional to its velocity, as depicted in the graph below. This is evident from the momentum formula, p = m * v.

These graphical representations highlight the fundamental differences between kinetic energy and momentum, despite their close relationship. Understanding these distinctions is crucial for correctly applying the appropriate formulas and solving physics problems involving these concepts.

Advanced Considerations and Relativistic Effects

While the classical mechanics approach we’ve discussed so far is sufficient for most everyday situations, it’s important to note that at extremely high velocities, the effects of special relativity become significant. In such cases, the relationships between kinetic energy, momentum, and velocity must be modified to account for relativistic effects.

The relativistic kinetic energy formula is given by:

KE = (γ - 1) * m * c^2

where γ (gamma) is the Lorentz factor, defined as:

γ = 1 / sqrt(1 - (v/c)^2)

and c is the speed of light.

Similarly, the relativistic momentum formula is:

p = γ * m * v

These relativistic formulas become increasingly important as the velocity of the object approaches the speed of light, and they are essential for understanding the behavior of high-energy particles in fields such as particle physics and astrophysics.

Conclusion

In this comprehensive guide, we have explored the intricate relationship between kinetic energy and momentum, providing you with the necessary tools and techniques to master the art of finding momentum from kinetic energy. By understanding the underlying formulas, working through practical examples, and exploring the graphical representations, you are now equipped with a deep understanding of this fundamental concept in classical mechanics.

Remember, the key to success in physics is not just memorizing formulas, but rather developing a strong conceptual grasp of the underlying principles. By applying the knowledge gained in this guide, you will be able to tackle a wide range of physics problems with confidence and ease.

Happy learning!

References

1. “Momentum, Kinetic Energy and Arrow Penetration” by Dr. Ed Ashby
2. “Relativistic Energy” from Lumen Learning
3. “Kinetic energy, mass, velocity, momentum, online calculators—let’s get it right” from Archery Talk
4. “How to find momentum when kinetic energy increase or decrease?” from Socratic
5. “Kinetic Energy and Velocity Lab” from Arbor Scientific