How to Find Maximum Acceleration in Simple Harmonic Motion

Summary

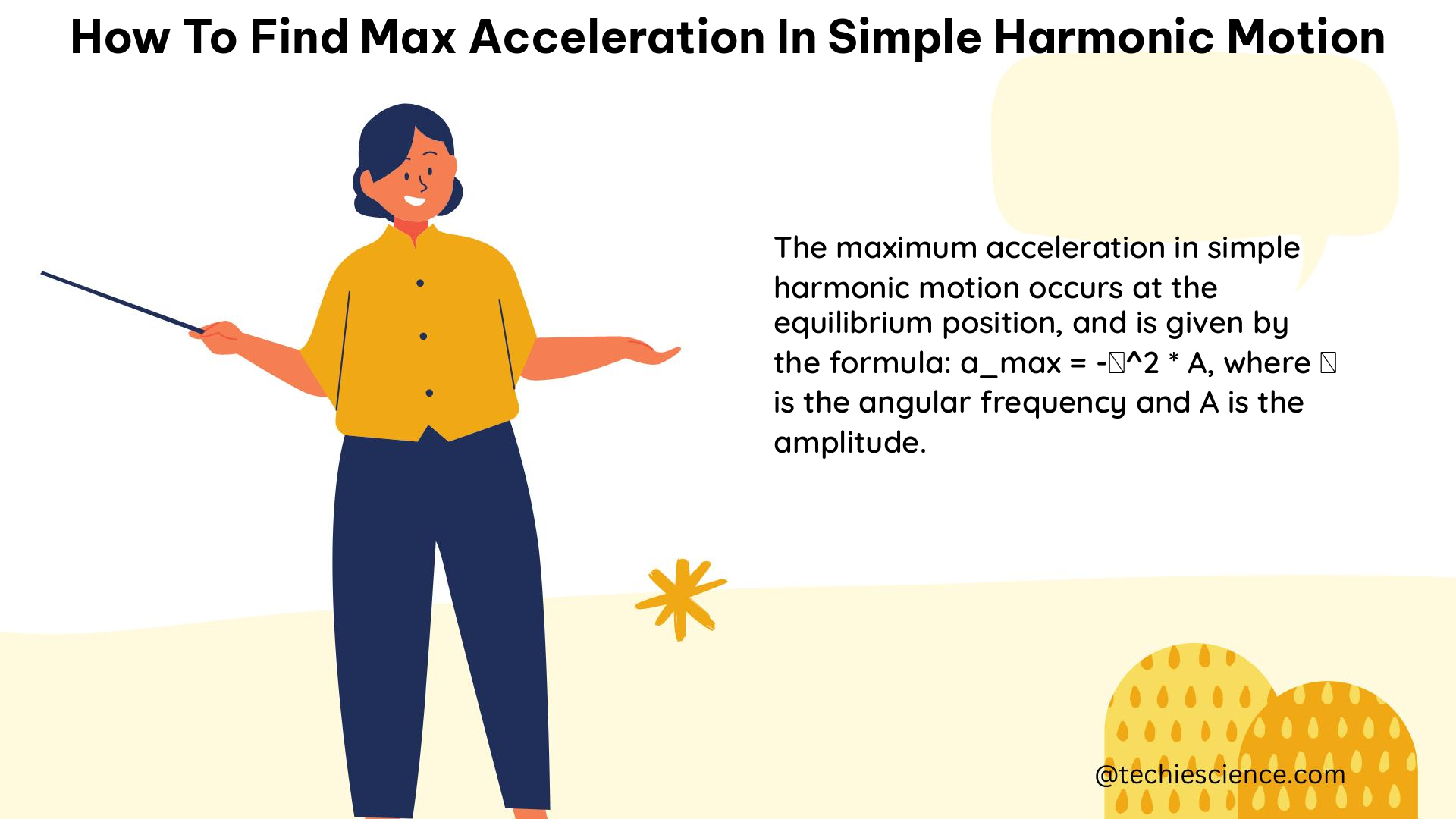
In simple harmonic motion, the maximum acceleration can be calculated using the equation a = Aω², where a is the maximum acceleration, A is the amplitude of the motion, and ω is the angular frequency. The angular frequency can be determined from the period T of the motion using the formula ω = 2π/T. This blog post provides a comprehensive guide on the theoretical background, formulas, examples, and problem-solving techniques to find the maximum acceleration in simple harmonic motion.

Understanding Simple Harmonic Motion

Simple harmonic motion (SHM) is a type of periodic motion where the restoring force is proportional to the displacement from the equilibrium position. This motion is commonly observed in various physical systems, such as a mass-spring system, a pendulum, or the vibration of atoms in a molecule.

The key characteristics of simple harmonic motion are:

1. Periodic Motion: The object undergoes a repeating pattern of motion, with a constant period T.
2. Restoring Force: The object experiences a force that is proportional to its displacement from the equilibrium position, directed towards the equilibrium.
3. Sinusoidal Displacement: The displacement of the object as a function of time follows a sinusoidal pattern.

Formulas for Simple Harmonic Motion

To find the maximum acceleration in simple harmonic motion, we need to understand the following formulas:

1. Displacement: The displacement x of the object from the equilibrium position is given by the equation:
   x = A cos(ωt)
   where A is the amplitude of the motion and ω is the angular frequency.

2. Velocity: The velocity v of the object is the time derivative of the displacement:
   v = -Aω sin(ωt)

3. Acceleration: The acceleration a of the object is the time derivative of the velocity:
   a = -Aω² cos(ωt)

4. Angular Frequency: The angular frequency ω is related to the period T of the motion by the formula:
   ω = 2π/T

Finding the Maximum Acceleration

To find the maximum acceleration in simple harmonic motion, we can use the equation:

a = Aω²

where:
– a is the maximum acceleration
– A is the amplitude of the motion
– ω is the angular frequency

Let’s consider an example to illustrate the process:

Example: A block attached to a spring on a frictionless table oscillates with an amplitude of 0.1 meters and a period of 2 seconds. Find the maximum acceleration of the block.

Given:
– Amplitude A = 0.1 m
– Period T = 2 s

Step 1: Calculate the angular frequency ω.

ω = 2π/T = 2π/2 s = π s⁻¹

Step 2: Calculate the maximum acceleration a.

a = Aω² = (0.1 m)(π s⁻¹)² = 0.314 m/s²

Therefore, the maximum acceleration of the block is 0.314 m/s².

Graphical Representation

The relationship between the displacement, velocity, and acceleration in simple harmonic motion can be visualized using graphs. The following figures show the graphs of these quantities:

As shown in the graphs, the acceleration is maximum at the points of maximum displacement, which correspond to the equilibrium position of the object.

Numerical Problems

1. A mass-spring system has a spring constant of 50 N/m and a mass of 2 kg. If the amplitude of the motion is 0.05 m, find the maximum acceleration of the mass.

Given:
– Spring constant k = 50 N/m
– Mass m = 2 kg
– Amplitude A = 0.05 m

Step 1: Calculate the angular frequency ω.
ω = √(k/m) = √(50 N/m) / (2 kg) = 5 rad/s

Step 2: Calculate the maximum acceleration a.
a = Aω² = (0.05 m)(5 rad/s)² = 1.25 m/s²

Therefore, the maximum acceleration of the mass is 1.25 m/s².

1. A simple pendulum has a length of 0.5 m. If the amplitude of the motion is 10 degrees, find the maximum acceleration of the pendulum.

Given:
– Length of the pendulum l = 0.5 m
– Amplitude θ = 10 degrees

Step 1: Convert the amplitude to radians.
θ = 10 degrees × (π/180 rad/degree) = 0.175 rad

Step 2: Calculate the angular frequency ω.
ω = √(g/l) = √(9.8 m/s²) / (0.5 m) = 4.43 rad/s

Step 3: Calculate the maximum acceleration a.
a = Aω² = (0.175 rad)(4.43 rad/s)² = 3.41 m/s²

Therefore, the maximum acceleration of the pendulum is 3.41 m/s².

Conclusion

In this blog post, we have explored the concept of simple harmonic motion and the methods to find the maximum acceleration in such a system. By understanding the key formulas, including the displacement, velocity, and acceleration equations, as well as the relationship between angular frequency and period, we can effectively calculate the maximum acceleration for various simple harmonic motion scenarios. The examples and numerical problems provided offer a practical guide for physics students to apply these principles and enhance their understanding of this fundamental topic in classical mechanics.

References

1. Serway, R. A., & Jewett, J. W. (2018). Physics for Scientists and Engineers with Modern Physics (10th ed.). Cengage Learning.
2. Halliday, D., Resnick, R., & Walker, J. (2013). Fundamentals of Physics (10th ed.). Wiley.
3. Giancoli, D. C. (2014). Physics for Scientists and Engineers with Modern Physics (4th ed.). Pearson.
4. OpenStax. (2016). University Physics Volume 1. OpenStax.
5. Dummies.com. (n.d.). Calculating the Acceleration of an Object in Simple Harmonic Motion. Retrieved from https://www.dummies.com/article/academics-the-arts/science/physics/calculating-the-acceleration-of-an-object-in-simple-harmonic-motion-174021/
6. Study.com. (n.d.). Calculating the Maximum Acceleration of an Object in Simple Harmonic Motion. Retrieved from https://study.com/skill/learn/calculating-the-maximum-acceleration-of-an-object-in-simple-harmonic-motion-explanation.html