How to Find Kinetic Energy Lost in an Inelastic Collision: A Comprehensive Guide

When two objects collide in an inelastic manner, a portion of their initial kinetic energy is lost, often in the form of heat, sound, or deformation. Understanding how to calculate this energy loss is crucial for analyzing the dynamics of various physical systems, from car crashes to the motion of celestial bodies. In this comprehensive guide, we will delve into the step-by-step process of determining the kinetic energy lost in an inelastic collision.

Defining Inelastic Collision

An inelastic collision is a type of collision where the colliding objects stick together after the impact, or they move with a combined velocity. This is in contrast to an elastic collision, where the objects bounce off each other, and the total kinetic energy is conserved. In an inelastic collision, a portion of the initial kinetic energy is converted into other forms of energy, such as heat or sound.

Calculating Initial Kinetic Energy

The first step in finding the kinetic energy lost in an inelastic collision is to determine the initial kinetic energy of the objects involved. The formula for calculating the kinetic energy of an object is:

KE = 1/2 mv^2

where:
– KE is the kinetic energy of the object
– m is the mass of the object
– v is the velocity of the object

To find the total initial kinetic energy, you would need to calculate the kinetic energy for each object and then add them together.

Calculating Final Kinetic Energy

After the collision, the objects may stick together or move with a combined velocity. To calculate the final kinetic energy, you can use the formula:

KE = 1/2 (m1 + m2)v^2

where:
– m1 and m2 are the masses of the two objects
– v is the combined velocity of the objects after the collision

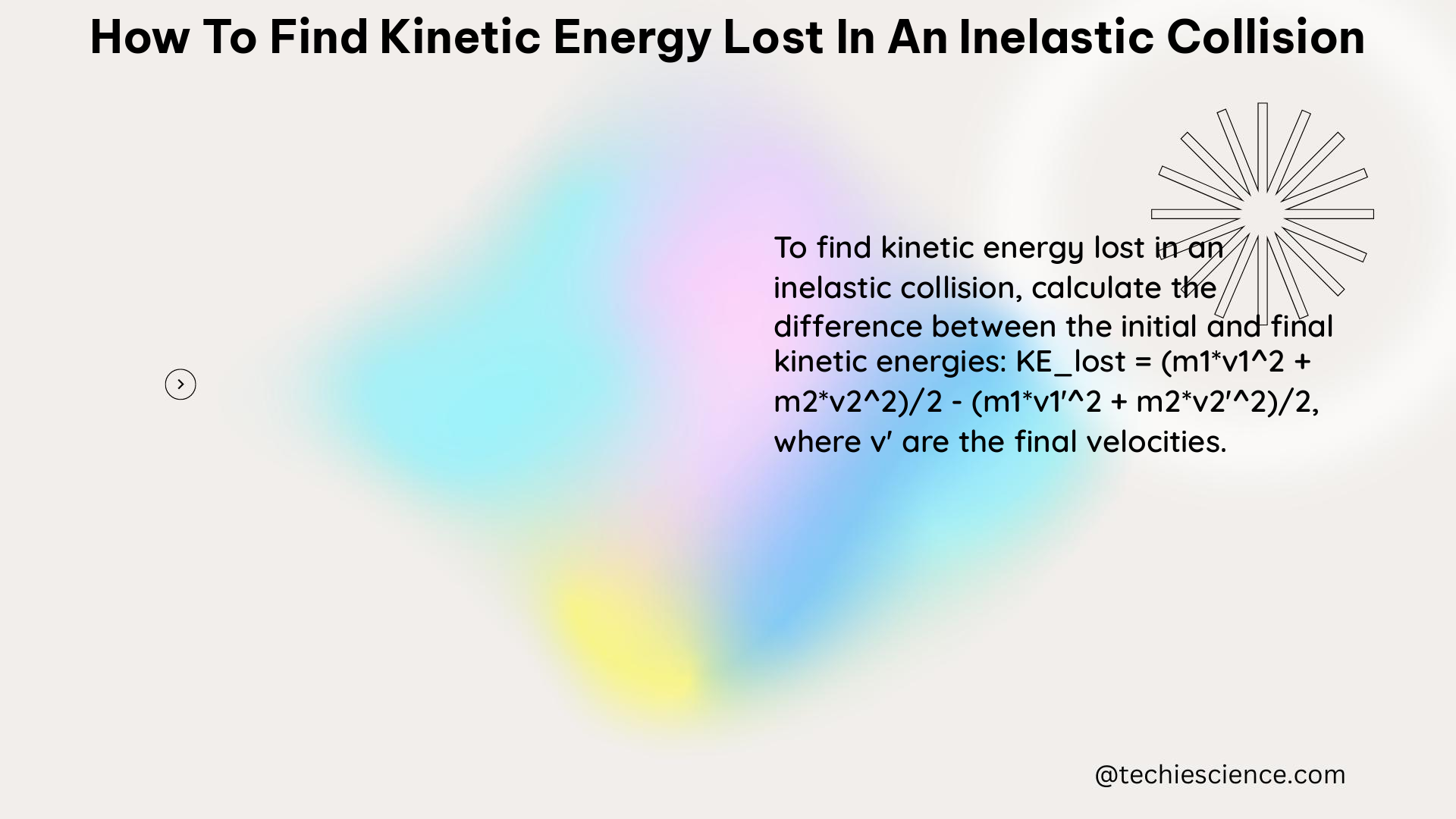
Determining Kinetic Energy Loss

The kinetic energy lost in the inelastic collision is the difference between the initial kinetic energy and the final kinetic energy:

ΔKE = Initial KE - Final KE

This represents the amount of energy that was converted into other forms, such as heat or sound, during the collision.

Using the Coefficient of Restitution

Another way to calculate the kinetic energy lost in an inelastic collision is by using the coefficient of restitution (e), which is a measure of the energy lost during the collision. The coefficient of restitution is defined as the ratio of the relative velocity of the objects after the collision to their relative velocity before the collision.

The formula for calculating the kinetic energy lost using the coefficient of restitution is:

ΔKE = 1/2 m1v1^2 + 1/2 m2v2^2 - 1/2 (m1 + m2)ve^2

where:
– m1 and m2 are the masses of the objects
– v1 and v2 are the velocities of the objects before the collision
– ve is the velocity of the objects after the collision
– e is the coefficient of restitution

The coefficient of restitution can range from 0 (for a perfectly inelastic collision) to 1 (for a perfectly elastic collision).

Factors Affecting Kinetic Energy Loss

The kinetic energy lost in an inelastic collision can be influenced by various factors, including:

1. Friction: Friction between the colliding objects can dissipate energy and contribute to the overall kinetic energy loss.
2. Sound and Heat: Some of the initial kinetic energy may be converted into sound or heat energy during the collision.
3. Deformation: If the colliding objects undergo significant deformation, the energy required to deform the objects can also contribute to the kinetic energy loss.
4. Elasticity and Stiffness: The physical properties of the colliding objects, such as their elasticity and stiffness, can affect the energy dissipation during the collision.

It’s important to note that the equations presented in this guide do not directly account for these additional factors, but they provide a general solution for the kinetic energy lost in an inelastic collision based on the masses and velocities of the objects.

Examples and Numerical Problems

To better illustrate the concepts, let’s consider a few examples and numerical problems:

1. Example 1: Two objects with masses m1 = 2 kg and m2 = 3 kg are moving towards each other with velocities v1 = 5 m/s and v2 = -3 m/s, respectively. After the collision, the objects stick together and move with a combined velocity of v = 1 m/s. Calculate the kinetic energy lost in the collision.

Solution:
– Initial kinetic energy: KE_initial = 1/2 * (2 kg * 5 m/s^2 + 3 kg * (-3 m/s)^2) = 17.5 J
– Final kinetic energy: KE_final = 1/2 * (2 kg + 3 kg) * (1 m/s)^2 = 2.5 J
– Kinetic energy lost: ΔKE = KE_initial - KE_final = 17.5 J - 2.5 J = 15 J

1. Example 2: Two objects with masses m1 = 4 kg and m2 = 6 kg are moving towards each other with velocities v1 = 8 m/s and v2 = -6 m/s, respectively. The coefficient of restitution for the collision is e = 0.3. Calculate the kinetic energy lost in the collision.

Solution:
– Initial kinetic energy: KE_initial = 1/2 * (4 kg * 8 m/s^2 + 6 kg * (-6 m/s)^2) = 160 J
– Final velocity: ve = -e * (v1 - v2) = -0.3 * (8 m/s - (-6 m/s)) = 4.2 m/s
– Final kinetic energy: KE_final = 1/2 * (4 kg + 6 kg) * (4.2 m/s)^2 = 42 J
– Kinetic energy lost: ΔKE = KE_initial - KE_final = 160 J - 42 J = 118 J

These examples demonstrate how to apply the formulas and principles discussed in this guide to calculate the kinetic energy lost in inelastic collisions.

Conclusion

Determining the kinetic energy lost in an inelastic collision is a crucial step in understanding the dynamics of various physical systems. By following the step-by-step process outlined in this guide, you can accurately calculate the energy lost during an inelastic collision, taking into account the initial and final kinetic energies, as well as the coefficient of restitution. Remember that additional factors, such as friction, sound, and deformation, can also contribute to the overall energy loss, but the equations provided in this guide offer a general solution based on the masses and velocities of the colliding objects.

Reference:

1. What factors contribute to the loss of kinetic energy in a partially inelastic collision
2. Inelastic Collisions
3. Determining Kinetic Energy Lost in Inelastic Collisions – Brilliant