Mastering the Art of Calculating Kinetic Energy 2: A Comprehensive Guide

In the realm of physics, understanding the concept of kinetic energy is crucial for analyzing the motion and behavior of objects. Kinetic energy, denoted as KE, is the energy an object possesses due to its motion. When it comes to calculating kinetic energy, the formula KE = 1/2 * m * v^2 is the fundamental equation that governs this phenomenon. In this comprehensive guide, we will delve into the intricacies of finding kinetic energy 2, providing you with a deep understanding of the underlying principles and practical applications.

The Kinetic Energy Formula: Unlocking the Secrets

The formula for kinetic energy is derived from the principles of classical mechanics and is a direct consequence of the work-energy theorem. The formula is expressed as:

KE = 1/2 * m * v^2

Where:
– KE is the kinetic energy of the object, measured in Joules (J)
– m is the mass of the object, measured in kilograms (kg)
– v is the velocity of the object, measured in meters per second (m/s)

This formula is the cornerstone of understanding and calculating kinetic energy, and it is essential to master its application in various scenarios.

Applying the Kinetic Energy Formula: Step-by-Step Approach

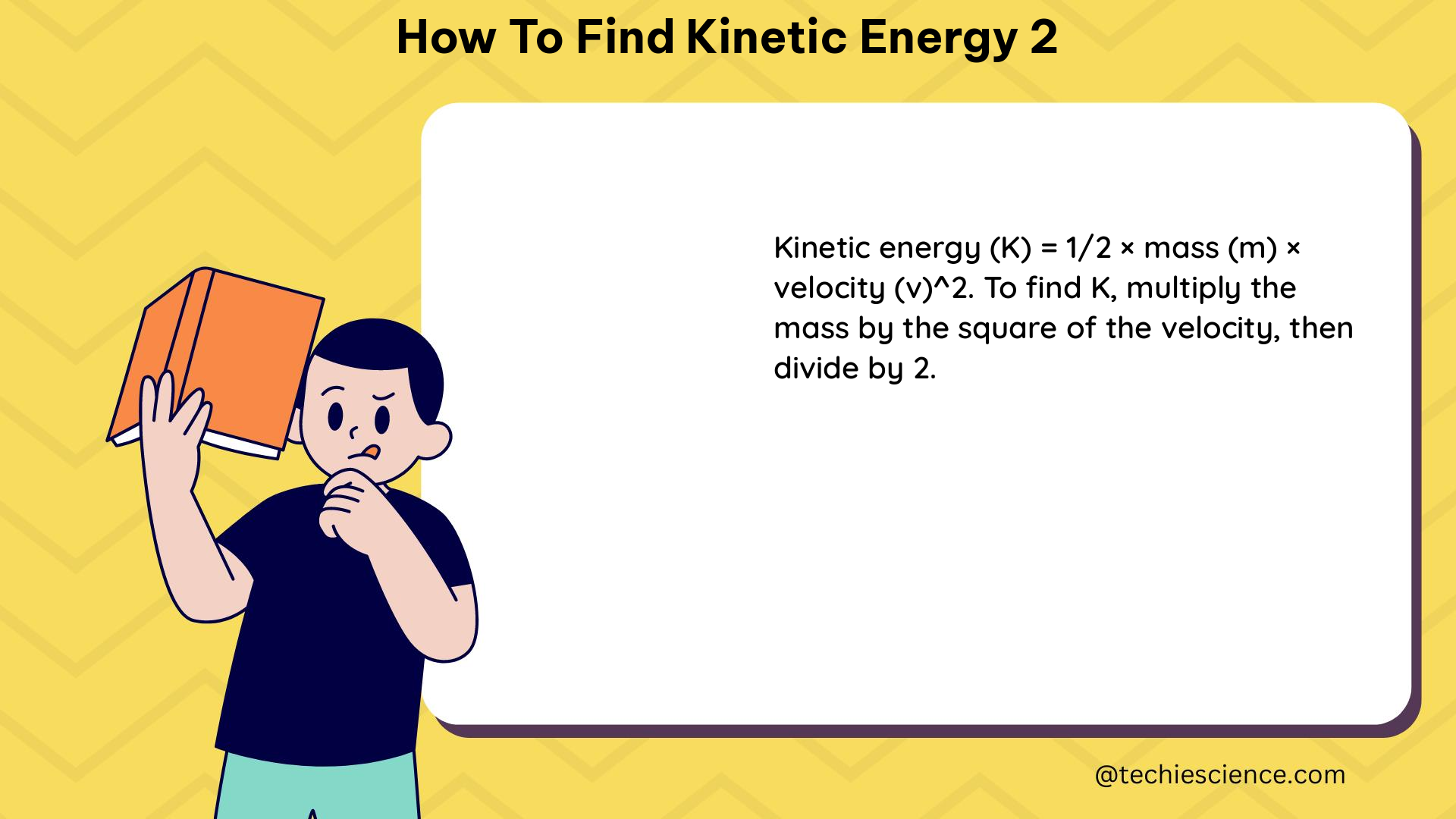
To find the kinetic energy of an object, you need to follow these steps:

1. Identify the Mass (m): Determine the mass of the object in kilograms (kg). This information may be provided in the problem statement or can be measured using appropriate equipment.

2. Determine the Velocity (v): Identify the velocity of the object in meters per second (m/s). This value can be given or calculated based on the information provided in the problem.

3. Substitute the Values: Plug the values of mass (m) and velocity (v) into the kinetic energy formula: KE = 1/2 * m * v^2.

4. Calculate the Kinetic Energy: Perform the mathematical operations to find the value of the kinetic energy (KE) in Joules (J).

Let’s illustrate this process with an example:

Example 1: An object with a mass of 5 kg is moving at a velocity of 10 m/s. Calculate its kinetic energy.

Given:
– Mass (m) = 5 kg
– Velocity (v) = 10 m/s

Substituting the values into the formula:
KE = 1/2 * m * v^2
KE = 1/2 * 5 kg * (10 m/s)^2
KE = 1/2 * 5 * 100
KE = 250 J

Therefore, the kinetic energy of the object is 250 Joules.

Inverse Kinetic Energy Calculation: Determining Velocity

In some cases, you may be given the kinetic energy and the mass of an object, and you need to find the velocity. To do this, you can rearrange the kinetic energy formula to solve for the velocity:

v = sqrt(2 * KE / m)

Where:
– v is the velocity of the object, measured in meters per second (m/s)
– KE is the kinetic energy of the object, measured in Joules (J)
– m is the mass of the object, measured in kilograms (kg)

Let’s consider another example:

Example 2: An object with a mass of 10 kg has a kinetic energy of 500 Joules. Calculate the velocity of the object.

Given:
– Mass (m) = 10 kg
– Kinetic Energy (KE) = 500 J

Substituting the values into the formula:
v = sqrt(2 * KE / m)
v = sqrt(2 * 500 J / 10 kg)
v = sqrt(100)
v = 10 m/s

Therefore, the velocity of the object is 10 meters per second.

Advanced Kinetic Energy Concepts

As you delve deeper into the world of kinetic energy, you may encounter more complex scenarios and applications. Here are some advanced concepts you should be familiar with:

1. Rotational Kinetic Energy: When an object is rotating, it possesses rotational kinetic energy, which is calculated using the formula: KE = 1/2 * I * ω^2, where I is the moment of inertia and ω is the angular velocity.

2. Kinetic Energy and Momentum: Kinetic energy is closely related to the concept of momentum, which is the product of an object’s mass and velocity. The relationship between kinetic energy and momentum is given by: KE = p^2 / (2m), where p is the momentum of the object.

3. Kinetic Energy and Work: The work done on an object is equal to the change in its kinetic energy, as described by the work-energy theorem: W = ΔKE, where W is the work done and ΔKE is the change in kinetic energy.

4. Kinetic Energy and Collisions: In the study of collisions, kinetic energy plays a crucial role in understanding the energy transformations and the conservation of energy principles.

5. Relativistic Kinetic Energy: In the realm of special relativity, the formula for kinetic energy needs to be modified to account for the effects of high-speed motion, leading to the relativistic kinetic energy formula: KE = (γ – 1) * mc^2, where γ is the Lorentz factor and c is the speed of light.

These advanced concepts provide a deeper understanding of the role of kinetic energy in various physical phenomena and can be explored further through additional resources and problem-solving exercises.

Conclusion

Mastering the calculation of kinetic energy 2 is a fundamental skill in the study of physics. By understanding the underlying formula, applying it step-by-step, and exploring advanced concepts, you can develop a comprehensive understanding of this essential topic. Remember to practice regularly, experiment with different scenarios, and seek out additional resources to deepen your knowledge. With dedication and persistence, you will become proficient in the art of calculating kinetic energy 2.

Reference:

1. Kinetic Energy and Velocity Lab – Arbor Scientific
2. How was the formula for kinetic energy found, and who found it? – Physics Stack Exchange
3. Kinetic & Potential Energy | Definition, Formula & Calculations – Study.com