How To Find Final Velocity : With Force, Mass, Time, Distance, Momentum etc And Problems

Finding the final velocity is an essential part of understanding the motion of an object. Whether you’re studying physics or simply curious about the speed at which an object is moving, knowing how to calculate the final velocity is key. In this blog post, we’ll explore various methods and formulas to find the final velocity in different scenarios. From basic calculations to more advanced techniques, let’s dive into the world of finding final velocity!

Basic Formula to Calculate Final Velocity

how to find final velocity — Image by NSF – Wikimedia Commons, Wikimedia Commons, Licensed under CC BY 4.0.

A. The Standard Formula

The standard formula to calculate final velocity is:

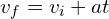
Where:
– (v_f) represents the final velocity
– (v_i) is the initial velocity
– (a) stands for acceleration
– (t) denotes time

B. Explanation of Variables in the Formula

To understand the formula better, let’s break down the variables involved:

Initial Velocity ((v_i)): This is the speed at which an object is moving at the beginning of a given time interval.
Acceleration ((a)): Acceleration refers to the rate at which an object’s velocity changes over time. It can be positive (speeding up) or negative (slowing down).
Time ((t)): Time represents the duration for which an object is in motion or the interval during which we want to calculate the final velocity.

By plugging in the values of initial velocity, acceleration, and time into the formula, we can determine the final velocity accurately.

How to Determine Final Velocity with Given Parameters

Now, let’s explore different scenarios and methods for finding the final velocity when specific parameters are given.

A. Finding Final Velocity with Initial Velocity, Acceleration, and Time

If you already know the values of the initial velocity, acceleration, and time, you can use the standard formula mentioned earlier to calculate the final velocity. Let’s look at an example:

Example 1: A car starts from rest and accelerates at 5 m/s² for 10 seconds. What is its final velocity?

Using the formula (v_f = v_i + at), we can substitute the given values:

$v_f = 0 + (5 \, \text{m/s²}\cdot (10 \, \text{s}))$

Simplifying the equation, we find:

$v_f = 50 \, \text{m/s}$

Therefore, the car’s final velocity is 50 m/s.

B. Calculating Final Velocity with Distance and Time

In some cases, you may be given the distance traveled by an object instead of the initial velocity or acceleration. In such situations, you can use the following formula to find the final velocity:

Where:
– (v_f) represents the final velocity
– (v_i) is the initial velocity
– (a) stands for acceleration
– (d) denotes the distance traveled

To better understand this formula, let’s go through an example:

Example 2: A ball is dropped from a height of 10 meters. What is its final velocity just before hitting the ground? Consider the acceleration due to gravity as $(9.8 \, \text{m/s²})$ .

Using the formula $v_f = \sqrt{v_i^2 + 2ad}$ , we can substitute the given values:

$v_f = \sqrt{0^2 + 2 \cdot (9.8 \, \text{m/s²}\cdot (10 \, \text{m})})$

Simplifying the equation, we find:

$v_f \approx 14 \, \text{m/s}$

Thus, the ball’s final velocity just before hitting the ground is approximately 14 m/s.

C. Determining Final Velocity with Initial Velocity and Distance

If you have the initial velocity and the distance traveled, you can use the following formula to find the final velocity:

This formula is similar to the one we used in the previous section but eliminates the need for acceleration. Let’s look at an example:

Example 3: A rider on a bike is traveling at 20 m/s. If the rider applies brakes and comes to a stop after traveling a distance of 50 meters, what is the final velocity?

Using the formula $v_f = \sqrt{v_i^2 + 2ad}$ , we can substitute the given values:

$v_f = \sqrt{(20 \, \text{m/s}^2 + 2 \cdot (0) \cdot (50 \, \text{m})})$

Simplifying the equation, we find:

$v_f = 20 \, \text{m/s}$

Therefore, the rider’s final velocity is 20 m/s.

Special Cases in Finding Final Velocity

Image by Rwolf01 – Wikimedia Commons, Wikimedia Commons, Licensed under CC BY-SA 4.0.

A. How to Calculate Final Velocity in Projectile Motion

In projectile motion, an object follows a curved path under the influence of gravity. To calculate the final velocity of a projectile, we need to consider the horizontal and vertical components of its motion separately and then combine them using vector addition. The final velocity will have both a magnitude and a direction.

B. Determining Final Velocity without Initial Velocity

In some cases, you may need to find the final velocity without knowing the initial velocity. In such scenarios, you can use equations related to conservation of energy, such as the principle of conservation of mechanical energy or the work-energy theorem. These equations allow you to calculate the final velocity based on other known parameters like potential energy, kinetic energy, or work done.

C. Finding Final Velocity without Time and Acceleration

If you don’t have information about time and acceleration, it becomes challenging to directly calculate the final velocity. However, you can still analyze the motion using other parameters like displacement, initial velocity, or equations of motion to find the final velocity indirectly.

Advanced Methods to Determine Final Velocity

A. Calculating Final Velocity with Kinetic Energy

The kinetic energy of an object is directly related to its velocity. By utilizing the equation for kinetic energy, you can find the final velocity of an object when given its mass and initial kinetic energy. This method can be particularly useful when other parameters are unknown or difficult to measure directly.

B. Finding Final Velocity with Impulse

Impulse is the change in momentum experienced by an object. By using the impulse-momentum principle, which states that the impulse acting on an object is equal to the change in its momentum, you can determine the final velocity of an object after a collision or an interaction with an external force.

C. Determining Final Velocity after Collision

When two objects collide, their final velocities can be calculated using the principles of conservation of momentum and energy. By considering the masses, initial velocities, and coefficients of restitution of the objects involved, you can determine their final velocities after the collision.

Practical Examples of Finding Final Velocity

A. Worked out Example: Final Velocity in Free Fall

Let’s consider an example of an object in free fall due to gravity. Suppose an object is dropped from rest and falls for 5 seconds. Using the formula (v_f = v_i + at), we can calculate the final velocity.

Given:
– $v_i = 0 \, \text{m/s}$ (initial velocity)
– $(a = 9.8 \, \text{m/s²})$ (acceleration due to gravity)
– $(t = 5 \, \text{s}) (time)$

Using the formula (v_f = v_i + at), we have:
$v_f = 0 + (9.8 \, \text{m/s²} \cdot (5 \, \text{s}) = 49 \, \text{m/s})$

Therefore, the final velocity of the object in free fall after 5 seconds is 49 m/s.

B. Worked out Example: Final Velocity in Elastic Collision

Let’s consider a scenario where two objects collide elastically. Suppose a 2 kg ball moving at 5 m/s collides head-on with a stationary 1 kg ball. By applying the principles of conservation of momentum and energy, we can find the final velocities of the balls.

Given:
– Mass of ball 1 $m_1$ = 2 kg
– Mass of ball 2 $m_2$ = 1 kg
– Initial velocity of ball 1 $v_{i1}$ = 5 m/s
– Initial velocity of ball 2 $v_{i2}$ = 0 m/s

Using the conservation of momentum equation:
$(m_1v_{i1} + m_2v_{i2} = m_1v_{f1} + m_2v_{f2})$

And the conservation of kinetic energy equation:
$({2}m_1v_{i1}^2 + {2}m_2v_{i2}^2 = {2}m_1v_{f1}^2 + {2}m_2v_{f2}^2)$

By solving these equations simultaneously, we find:
$v_{f1} = {m_1 + m_2}v_{i1} = {2 + 1}(5) = {3}(5) = {3} \, \text{m/s}$
$v_{f2} = {m_1 + m_2}v_{i1} = {2 + 1}(5) = {3}(5) = {3} \, \text{m/s}$

Therefore, after the elastic collision, the final velocity of the 2 kg ball is $({3} \, \text{m/s})$ and the final velocity of the 1 kg ball is $({3} \, \text{m/s})$ .

C. Worked out Example: Final Velocity with Constant Acceleration

Let’s consider an object with a constant acceleration of 2 m/s². If its initial velocity is 10 m/s and it travels a distance of 100 meters, we can calculate the final velocity using the formula $v_f = \sqrt{v_i^2 + 2ad}$ .

Given:
– $v_i = 10 \, \text{m/s}$ (initial velocity)
– $(a = 2 \, \text{m/s²})$ (acceleration)
– $(d = 100 \, \text{m})$ (distance)

Using the formula $v_f = \sqrt{v_i^2 + 2ad}$ , we have:
$v_f = \sqrt{10^2 + 2 \cdot (2 \cdot (100)} = \sqrt{100 + 400} = \sqrt{500} \approx 22.36 \, \text{m/s})$

Therefore, the object’s final velocity after traveling 100 meters with a constant acceleration of 2 m/s² is approximately 22.36 m/s.

Common Mistakes and Misconceptions in Finding Final Velocity

When finding the final velocity, certain mistakes or misconceptions can occur. It’s important to be aware of these to avoid errors in calculations:

Forgetting to include the appropriate units in the final velocity.
Neglecting to consider the direction of the final velocity, especially in cases of projectile motion or collisions.
Failing to use the correct formula or equations based on the given parameters.
Misinterpreting or misusing the signs of variables, especially when dealing with acceleration or distance.
Overlooking the effects of external forces, such as friction or air resistance, which may impact the final velocity.

By keeping these common mistakes in mind, you can ensure accurate calculations and a better understanding of finding final velocity.

And that concludes our exploration of finding final velocity! From the basic formulas to more advanced methods, we’ve covered various scenarios and techniques. Remember to practice these concepts with different examples to strengthen your understanding. The ability to calculate the final velocity is a valuable skill that will enhance your comprehension of motion and its dynamics. Keep studying and exploring the fascinating world of physics and mathematics!

Also Read:

SAKSHI KM

I am Sakshi Sharma, I have completed my post-graduation in applied physics. I like to explore in different areas and article writing is one of them. In my articles, I try to present physics in most understanding manner for the readers.