Summary
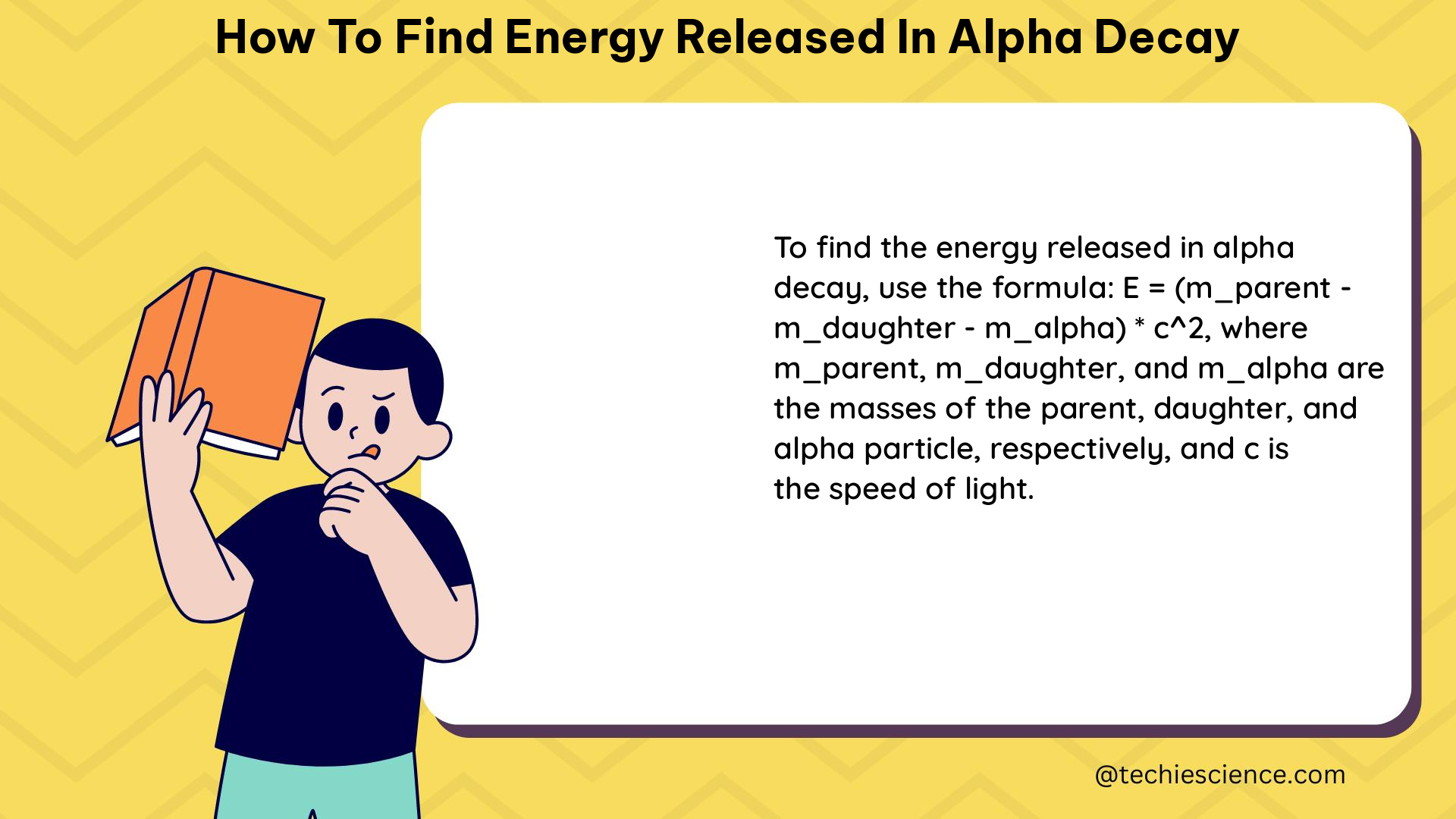
To find the energy released in alpha decay, we can use the formula E = (Δm)c^2, where Δm is the difference in mass between the parent nucleus and the products of the decay. This can be calculated by finding the masses of the parent nucleus and the products of the decay, and then subtracting the mass of the parent nucleus from the sum of the masses of the products. The energy released will be in the MeV range, which is about 10^6 times as great as typical chemical reaction energies.
Understanding Alpha Decay
Alpha decay is a type of radioactive decay in which a nucleus emits an alpha particle (a helium-4 nucleus) and transforms into a different element. This process occurs when the parent nucleus is unstable and has an excess of protons and neutrons, making it energetically favorable to undergo this transformation.
The energy released in alpha decay is a result of the difference in mass between the parent nucleus and the products of the decay. This energy is primarily converted into the kinetic energy of the emitted alpha particle, with a smaller portion going towards the recoil of the daughter nucleus.
Calculating the Energy Released
To calculate the energy released in an alpha decay process, we can use the formula:
E = (Δm)c^2
Where:
– E is the energy released in the decay (in MeV)
– Δm is the difference in mass between the parent nucleus and the products of the decay (in atomic mass units, u)
– c is the speed of light (approximately 3 × 10^8 m/s)
The steps to find the energy released in alpha decay are as follows:
- Identify the parent nucleus and the products of the decay.
- Determine the masses of the parent nucleus and the products of the decay using the values provided in Appendix A.
- Calculate the difference in mass (Δm) between the parent nucleus and the products of the decay.
- Substitute the value of Δm into the formula E = (Δm)c^2 to find the energy released in the decay.
Let’s go through an example to illustrate the process.
Example: Alpha Decay of 239Pu
In the alpha decay of 239Pu, the parent nucleus is 239Pu, and the products of the decay are 235U (the daughter nucleus) and an alpha particle (4He).
Using the masses provided in Appendix A, we can calculate the energy released as follows:
Δm = (mass of 235U + mass of 4He) – mass of 239Pu
Δm = (235.043924 u + 4.002602 u) – 239.052157 u
Δm = 239.046526 u
Now, we can substitute the value of Δm into the formula:
E = (Δm)c^2
E = (239.046526 u)(931.5 MeV/u)^2
E = 221.8 MeV
Therefore, the energy emitted in the alpha decay of 239Pu is 221.8 MeV.
It’s important to note that the masses given in Appendix A are atomic masses of neutral atoms, including their electrons. The mass of the electrons is the same before and after alpha decay, and so their masses subtract out when finding Δm.
Factors Affecting the Energy Released
The energy released in alpha decay is primarily determined by the difference in mass between the parent nucleus and the products of the decay. However, there are a few other factors that can influence the energy released:
- Nuclear Binding Energy: The energy released in alpha decay is related to the binding energy of the nucleus. Nuclei with higher binding energies are more stable and have less energy available for release during decay.
- Nuclear Shell Structure: The stability of a nucleus is also influenced by its shell structure, which can affect the energy released in alpha decay.
- Decay Modes: In some cases, a nucleus may undergo multiple decay modes, such as alpha decay and beta decay. The energy released can vary depending on the specific decay mode.
Practical Applications
The energy released in alpha decay has several practical applications in various fields, including:
- Nuclear Power: The energy released in alpha decay can be harnessed for the production of nuclear power, where the heat generated by the decay process is used to generate steam and drive turbines.
- Medical Imaging and Therapy: Certain radioactive isotopes that undergo alpha decay are used in medical imaging techniques, such as positron emission tomography (PET) and in targeted cancer therapies.
- Radiometric Dating: The predictable nature of radioactive decay, including alpha decay, is used in radiometric dating techniques to determine the age of geological and archaeological samples.
Conclusion
Understanding the process of finding the energy released in alpha decay is crucial for various applications in physics, nuclear engineering, and other related fields. By following the steps outlined in this guide and applying the relevant formulas and principles, you can accurately calculate the energy released in alpha decay processes.
References
- Radioactive Decay – an overview | ScienceDirect Topics
- Alpha Decay – an overview | ScienceDirect Topics
- Energy Released in Alpha Decay – YouTube
- Nuclear Decay and Conservation Laws | Physics – Lumen Learning
- Alpha Decay – Physics LibreTexts

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.