How to Find Energy Produced in Nuclear Reactions: A Comprehensive Guide

Nuclear reactions are fundamental processes that power various applications, from nuclear energy generation to medical diagnostics. Understanding the energy released in these reactions is crucial for understanding the underlying physics and optimizing their practical applications. In this comprehensive guide, we will delve into the step-by-step process of calculating the energy produced in nuclear reactions.

1. Calculate the Mass Defect

The first step in determining the energy produced in a nuclear reaction is to calculate the mass defect. The mass defect is the difference between the sum of the masses of the reactants and the sum of the masses of the products.

To calculate the mass defect, follow these steps:

1. Identify the reactants and products involved in the nuclear reaction.
2. Determine the masses of each reactant and product in unified atomic mass units (amu).
3. Add up the masses of all the reactants.
4. Add up the masses of all the products.
5. Subtract the sum of the product masses from the sum of the reactant masses to obtain the mass defect.

The mass defect can be expressed mathematically as:

Mass Defect = Σ(Masses of Reactants) - Σ(Masses of Products)

2. Convert Mass Defect to Energy

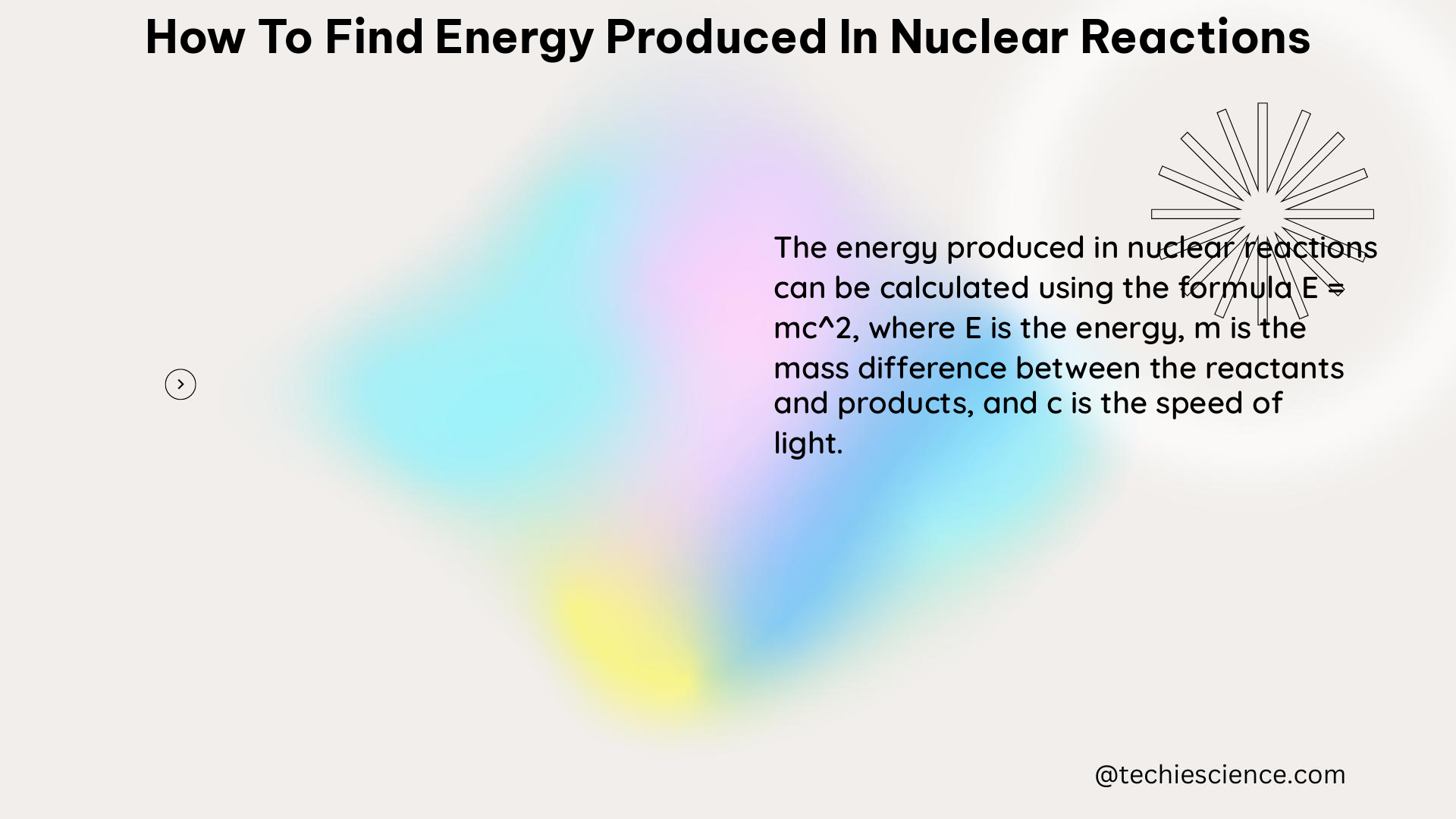
Once the mass defect has been calculated, the next step is to convert it to the corresponding energy released in the nuclear reaction. This is done using Einstein’s mass-energy equivalence equation:

E = mc^2

where:
– E is the energy released (in joules)
– m is the mass defect (in kilograms)
– c is the speed of light (3 × 10^8 m/s)

To convert the mass defect from amu to kilograms, you can use the conversion factor:

1 amu = 1.660539 × 10^-27 kg

Once you have the mass defect in kilograms, simply plug it into the mass-energy equivalence equation to calculate the energy released.

3. Scale Up for One Mole

If you want to find the energy released per mole of reactants, you can multiply the energy released for one nucleus by the Avogadro constant:

Energy Released per Mole = Energy Released per Nucleus × Avogadro Constant

where the Avogadro constant is 6.022 × 10^23 particles/mol.

Example Calculations

Let’s apply the steps outlined above to two common nuclear reactions: fusion and fission.

Fusion Reaction: 2H + 3H → 4He + 1n

1. Calculate the Mass Defect:
2. Reactant masses: 2H = 2.0141 amu, 3H = 3.0160 amu
3. Product masses: 4He = 4.0026 amu, 1n = 1.0087 amu

4. Mass defect = (2.0141 + 3.0160) – (4.0026 + 1.0087) = 0.0198 amu

5. Convert Mass Defect to Energy:

6. Convert mass defect to kilograms: 0.0198 amu × (1 kg / 6.022 × 10^23 amu) = 3.23 × 10^-29 kg

7. Energy released: E = mc^2 = 3.23 × 10^-29 kg × (3 × 10^8 m/s)^2 = 2.91 × 10^-12 J

8. Scale Up for One Mole:

9. Energy released per mole: 2.91 × 10^-12 J × 6.022 × 10^23 = 1.75 × 10^9 J/mol

Fission Reaction: 235U → 141Ba + 92Kr + 3 1n

1. Calculate the Mass Defect:
2. Reactant mass: 235U = 235.0439 amu
3. Product masses: 141Ba = 140.9144 amu, 92Kr = 91.9262 amu, 1n = 1.0087 amu

4. Mass defect = 235.0439 – (140.9144 + 91.9262 + 3 × 1.0087) = 0.1858 amu

5. Convert Mass Defect to Energy:

6. Convert mass defect to kilograms: 0.1858 amu × (1 kg / 6.022 × 10^23 amu) = 3.08 × 10^-28 kg

7. Energy released: E = mc^2 = 3.08 × 10^-28 kg × (3 × 10^8 m/s)^2 = 2.78 × 10^11 J

8. Scale Up for One Mole:

9. Energy released per mole: 2.78 × 10^11 J × 6.022 × 10^23 = 1.67 × 10^10 J/mol

Key Formulas and Values

• Einstein’s Mass-Energy Equivalence: E = mc^2
• c = 3 × 10^8 m/s (speed of light)
• 1 amu = 1.660539 × 10^-27 kg (conversion factor)

• 1 MeV = 1.602176 × 10^-13 J (conversion factor)

• Avogadro Constant: 6.022 × 10^23 particles/mol

References

1. Purdue University. (n.d.). Determining the Energy Change of a Nuclear Reaction. Retrieved from https://www.chem.purdue.edu/gchelp/howtosolveit/Nuclear/Energy_of_Nuclear_Change.htm
2. AstroNuclPhysics. (n.d.). Nuclear reactions and nuclear energy. Retrieved from https://astronuclphysics.info/JadRadFyzika3.htm
3. LibreTexts. (2020). 21.6: Energy Changes in Nuclear Reactions. Retrieved from https://chem.libretexts.org/Courses/University_of_Missouri/MU:__1330H_%28Keller%29/21:_Nuclear_Chemistry/21.6:_Energy_Changes_in_Nuclear_Reactions
4. YouTube. (2016). C.7 Calculating energy released in nuclear reactions (HL). Retrieved from https://www.youtube.com/watch?v=XzcgTbl8ClU
5. ScienceFlip. (n.d.). Quantitative Analysis of Nuclear Reactions. Retrieved from https://www.scienceflip.com.au/subjects/physics/fromtheuniversetotheatom/learn13/