A Comprehensive Guide on How to Find Average Acceleration

Summary

Average acceleration is a crucial concept in physics, as it helps us understand the rate of change in an object’s velocity over a given time interval. This comprehensive guide will provide you with a deep understanding of the formula, its applications, and step-by-step examples to help you master the art of finding average acceleration.

Understanding the Average Acceleration Formula

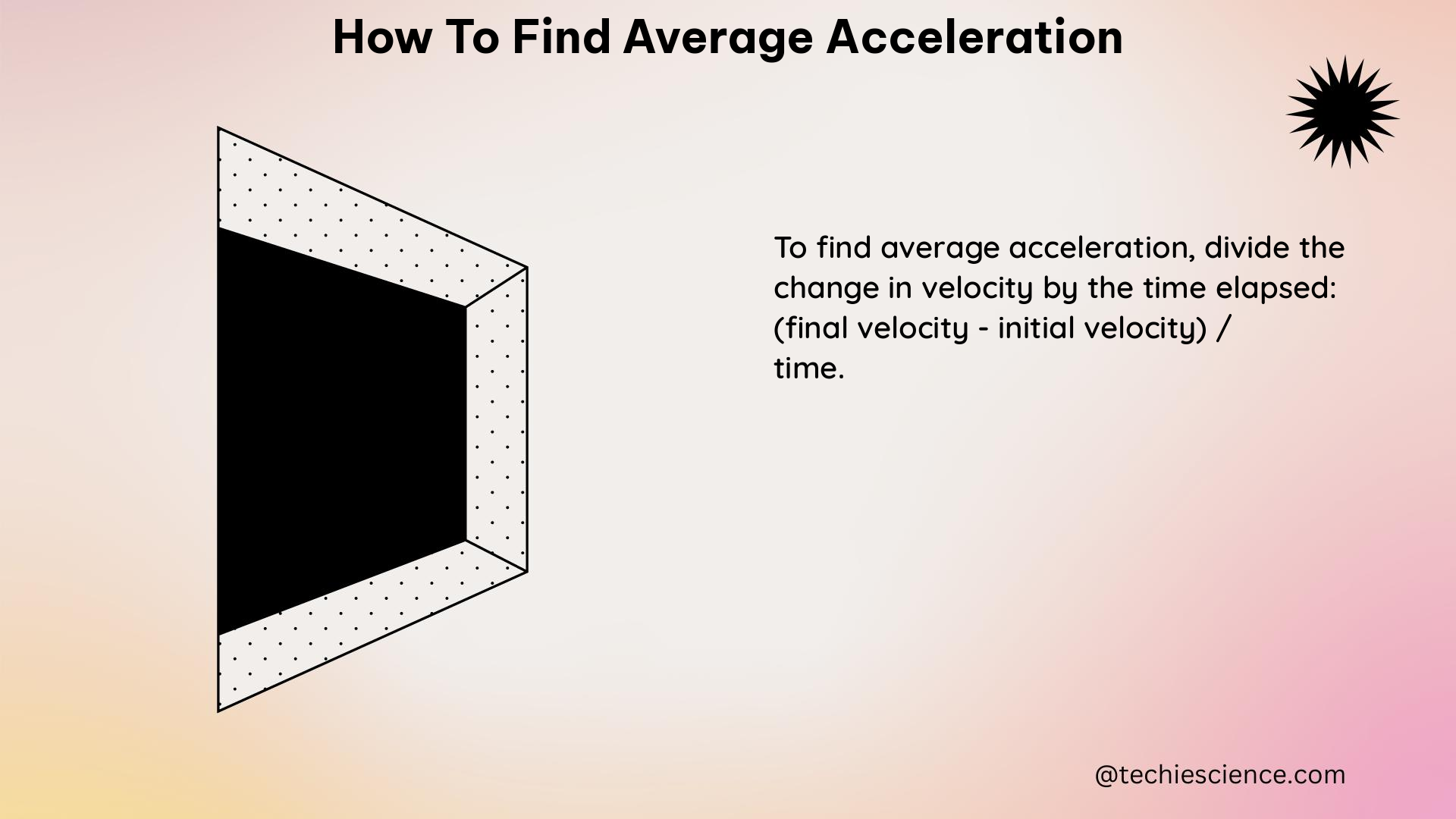
The formula for calculating average acceleration is:

a_av = (v_f - v_i) / t

Where:
– a_av is the average acceleration
– v_f is the final velocity
– v_i is the initial velocity
– t is the time interval

The units for average acceleration are meters per second squared (m/s^2).

Applying the Formula: Examples and Explanations

Example 1: Constant Acceleration

A car starts from rest and accelerates at a constant rate for 10 seconds, reaching a final velocity of 50 m/s. What is the car’s average acceleration?

To solve this problem, we can plug the given values into the average acceleration formula:

a_av = (v_f - v_i) / t
a_av = (50 m/s - 0 m/s) / 10 s
a_av = 5 m/s^2

This means that the car’s average acceleration is 5 m/s^2.

Example 2: Changing Velocity Direction

A ball is thrown straight up into the air with an initial velocity of 20 m/s. It reaches a maximum height and then falls back down to the ground. If the ball is in the air for a total of 6 seconds, what is its average acceleration?

In this case, the ball’s velocity changes direction, so we need to consider its velocity as positive when it is moving up and negative when it is moving down. At the maximum height, the ball’s velocity is 0 m/s. We can estimate that the ball reaches its maximum height after 2 seconds and then falls back down for the remaining 4 seconds.

a_av = (v_f - v_i) / t
a_av = (0 m/s - 20 m/s) / 6 s
a_av = -3.3 m/s^2

The negative sign indicates that the ball is decelerating (slowing down) as it falls back to the ground.

Example 3: Constant Velocity

A car is traveling at a constant speed of 60 m/s. What is its average acceleration?

Since the car is not changing its velocity, its average acceleration is 0 m/s^2.

a_av = (v_f - v_i) / t
a_av = (60 m/s - 60 m/s) / t
a_av = 0 m/s^2

Theorem and Derivation

The average acceleration formula can be derived from the basic kinematic equations of motion. The derivation is as follows:

Let’s consider an object that starts with an initial velocity v_i and reaches a final velocity v_f after a time interval t.

The displacement d of the object can be calculated using the equation:

d = v_i * t + 1/2 * a * t^2

Where a is the acceleration of the object.

Rearranging the equation, we get:

a = (v_f^2 - v_i^2) / (2 * d)

Now, if we consider the average acceleration over the time interval t, we can write:

a_av = (v_f - v_i) / t

This is the formula for average acceleration that we have been using throughout this guide.

Physics Formulas and Relationships

In addition to the average acceleration formula, there are several other important physics formulas and relationships that are relevant to this topic:

1. Velocity-time relationship:
   v = v_i + a * t

2. Displacement-time relationship:
   d = v_i * t + 1/2 * a * t^2

3. Velocity-displacement relationship:
   v^2 = v_i^2 + 2 * a * d

4. Relationship between average velocity and initial and final velocities:
   v_av = (v_i + v_f) / 2

These formulas and relationships can be used in conjunction with the average acceleration formula to solve more complex problems in kinematics.

Physics Numerical Problems

1. A car accelerates from 0 m/s to 20 m/s in 5 seconds. Calculate the average acceleration.

2. A ball is thrown vertically upward with an initial velocity of 30 m/s. If the ball reaches a maximum height of 45 m, what is the average acceleration of the ball during its upward motion?

3. A cyclist starts from rest and reaches a velocity of 12 m/s in 4 seconds. What is the average acceleration of the cyclist?

4. A car traveling at 50 m/s suddenly applies its brakes, and its velocity decreases to 20 m/s in 10 seconds. Calculate the average acceleration of the car during the braking process.

5. A skydiver jumps from a plane and falls for 8 seconds before opening their parachute. If the skydiver’s initial velocity is 0 m/s and their final velocity just before opening the parachute is 60 m/s, what is the average acceleration of the skydiver during the free fall?

Figures and Data Points

To better illustrate the concept of average acceleration, let’s consider the following motion diagram:

In this diagram, we can see the position of an object at different time intervals. By analyzing the changes in position and time, we can calculate the average acceleration using the formula.

Additionally, here are some data points that can be used to practice finding average acceleration:

Time (s)	Initial Velocity (m/s)	Final Velocity (m/s)	Average Acceleration (m/s^2)
2	0	10	5
5	20	0	-4
8	30	50	2.5
12	40	60	1.67
15	0	45	3

Conclusion

In this comprehensive guide, we have explored the concept of average acceleration, its formula, and various examples and applications. By understanding the underlying principles and practicing the numerical problems, you can develop a strong foundation in this crucial area of physics. Remember to always consider the direction of motion and use the appropriate sign when calculating average acceleration. With this knowledge, you’ll be well-equipped to tackle a wide range of kinematic problems.

References

1. How to Find Average Acceleration – Explanation
2. How to Determine the Average Acceleration of an Object Using a Motion Diagram – Explanation
3. Average Acceleration Over an Interval