How to Find Acceleration from Velocity: A Comprehensive Guide

Summary

Determining acceleration from velocity is a fundamental concept in physics, allowing us to understand the motion of objects and the forces acting upon them. This comprehensive guide will delve into the mathematical formulas, practical examples, and problem-solving techniques to help you master the art of finding acceleration from velocity.

Understanding the Acceleration-Velocity Relationship

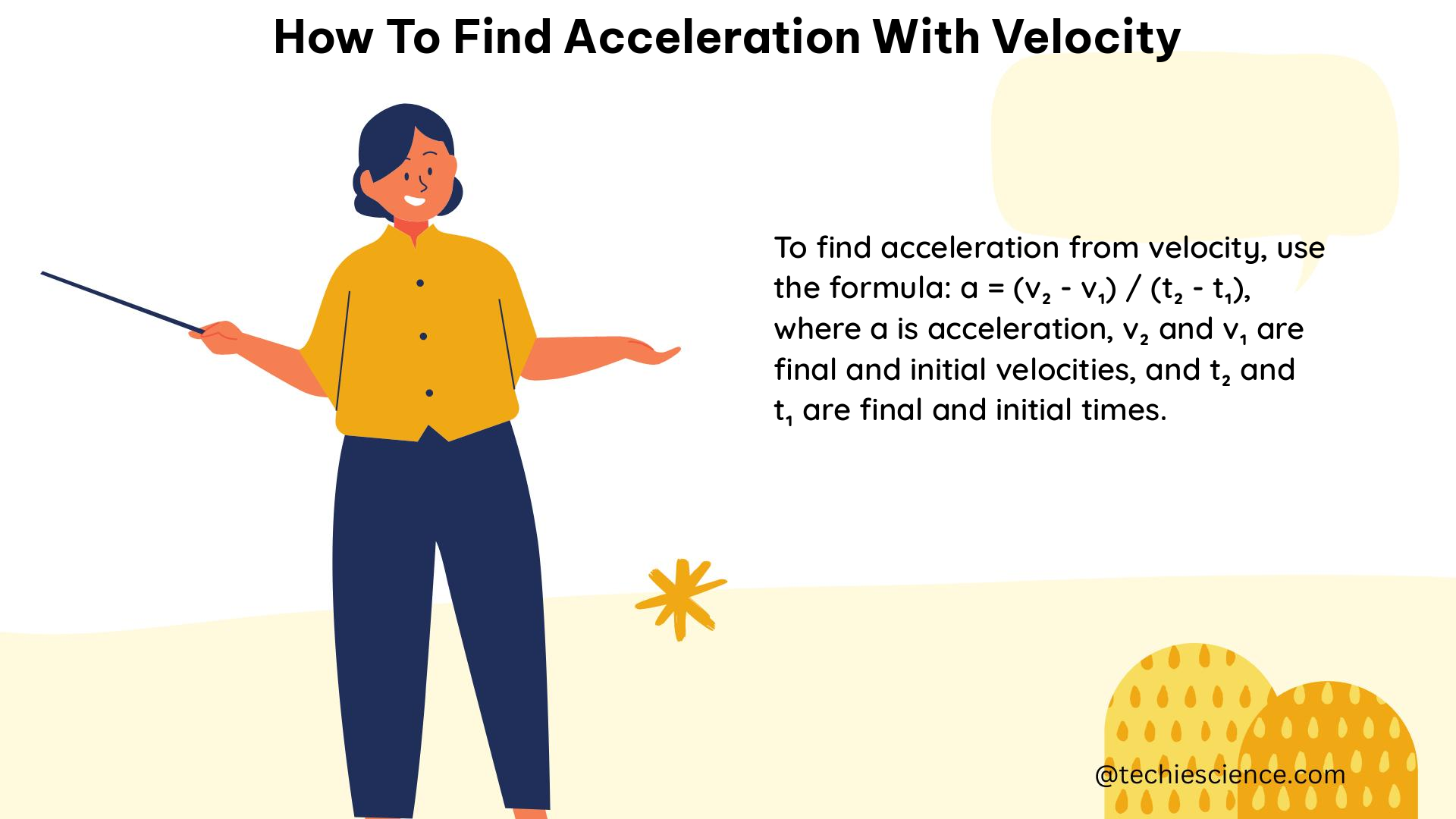
The relationship between acceleration and velocity is governed by the formula:

a = Δv / Δt

Where:
– a is the acceleration (in m/s²)
– Δv is the change in velocity (in m/s)
– Δt is the change in time (in s)

This formula allows us to calculate the average acceleration of an object by finding the net force and applying Newton’s Second Law of Motion. Once the acceleration is known, we can then determine how the velocity and position of the object change over time using kinematics and the kinematic equations.

Calculating Acceleration from Velocity Data

To find the acceleration from velocity data, follow these steps:

1. Identify the Initial and Final Velocities: Determine the starting velocity (v₀) and the ending velocity (v) of the object over the time interval.

2. Calculate the Change in Velocity: Find the difference between the final and initial velocities, Δv = v - v₀.

3. Determine the Time Interval: Identify the time interval (Δt) over which the velocity change occurred.

4. Apply the Acceleration Formula: Plug the values of Δv and Δt into the formula a = Δv / Δt to calculate the acceleration.

Here’s an example:

Suppose an object has an initial velocity of 10 m/s and a final velocity of 30 m/s, and the time interval is 4 seconds. We can calculate the acceleration as follows:

Δv = 30 m/s - 10 m/s = 20 m/s
Δt = 4 s
a = Δv / Δt = 20 m/s / 4 s = 5 m/s²

Therefore, the acceleration of the object is 5 m/s².

Graphical Representation of Acceleration

The relationship between velocity and acceleration can also be visualized using graphs. The slope of a velocity-time graph represents the acceleration of the object. Specifically:

• If the velocity-time graph is a straight line, the acceleration is constant.
• If the velocity-time graph is curved, the acceleration is changing.

By analyzing the slope of the velocity-time graph, you can determine the acceleration of the object at any given point.

Kinematic Equations and Acceleration

The kinematic equations, which describe the motion of an object, can be used in conjunction with the acceleration-velocity relationship to solve a variety of physics problems. The kinematic equations are:

v = v₀ + at
s = v₀t + (1/2)at²
v² = v₀² + 2as

Where:
– v is the final velocity (in m/s)
– v₀ is the initial velocity (in m/s)
– a is the acceleration (in m/s²)
– t is the time (in s)
– s is the displacement (in m)

By rearranging these equations and substituting the known values, you can solve for the unknown variables, such as the final velocity, displacement, or time.

Practical Applications and Examples

The ability to find acceleration from velocity data has numerous practical applications in various fields, including:

1. Transportation: Analyzing the acceleration and deceleration of vehicles to improve safety, fuel efficiency, and performance.
2. Sports: Evaluating the acceleration of athletes to optimize training and performance.
3. Engineering: Designing and analyzing the motion of mechanical systems, such as robots and machinery.
4. Astronomy: Studying the acceleration of celestial bodies, such as planets and comets, to understand their motion and the forces acting upon them.

Here’s an example problem:

A car accelerates from rest (0 m/s) to a final velocity of 20 m/s over a distance of 50 meters. Calculate the acceleration of the car.

Given:
– Initial velocity, v₀ = 0 m/s
– Final velocity, v = 20 m/s
– Displacement, s = 50 m

Using the kinematic equation v² = v₀² + 2as, we can solve for the acceleration:

v² = v₀² + 2as
(20 m/s)² = (0 m/s)² + 2a(50 m)
a = (20 m/s)² / (2 × 50 m)
a = 4 m/s²

Therefore, the acceleration of the car is 4 m/s².

Advanced Techniques and Considerations

1. Instantaneous Acceleration: While the formula a = Δv / Δt provides the average acceleration over a time interval, you can also calculate the instantaneous acceleration by taking the derivative of the velocity function with respect to time.

2. Acceleration Profiles: Analyzing the acceleration profile of an object, which describes how the acceleration changes over time, can provide valuable insights into the forces and dynamics involved.

3. Coordinate Systems and Vectors: When dealing with motion in multiple dimensions, it’s important to consider the acceleration in each coordinate axis and represent it as a vector quantity.

4. Numerical Integration and Differentiation: For more complex motion patterns, you may need to use numerical integration or differentiation techniques to determine the acceleration from velocity data.

5. Experimental Measurements: In real-world situations, you may need to use specialized equipment, such as accelerometers or velocity sensors, to accurately measure the acceleration and velocity of an object.

Conclusion

Finding acceleration from velocity is a fundamental skill in physics, with applications across various fields. By understanding the mathematical relationships, graphical representations, and practical examples, you can develop a comprehensive understanding of this concept and apply it to solve a wide range of problems. Remember to practice regularly, explore advanced techniques, and stay curious about the fascinating world of motion and dynamics.

Reference:

1. How To Find The Acceleration From a Velocity Time Graph – Physics
2. Velocity & Acceleration as Functions – Lesson – Study.com
3. Speed, Velocity & Acceleration | Difference & Relationship – Lesson