How to Find Acceleration with Force and Mass: A Comprehensive Guide

Summary

Calculating the acceleration of an object using the given force and mass is a fundamental concept in classical mechanics. This comprehensive guide will walk you through the step-by-step process of finding acceleration using the formula a = F/m, where a is the acceleration, F is the force, and m is the mass. We’ll also explore the impact of factors like friction and air resistance on the acceleration, and provide practical examples and numerical problems to help you master this essential skill.

Understanding the Acceleration Formula

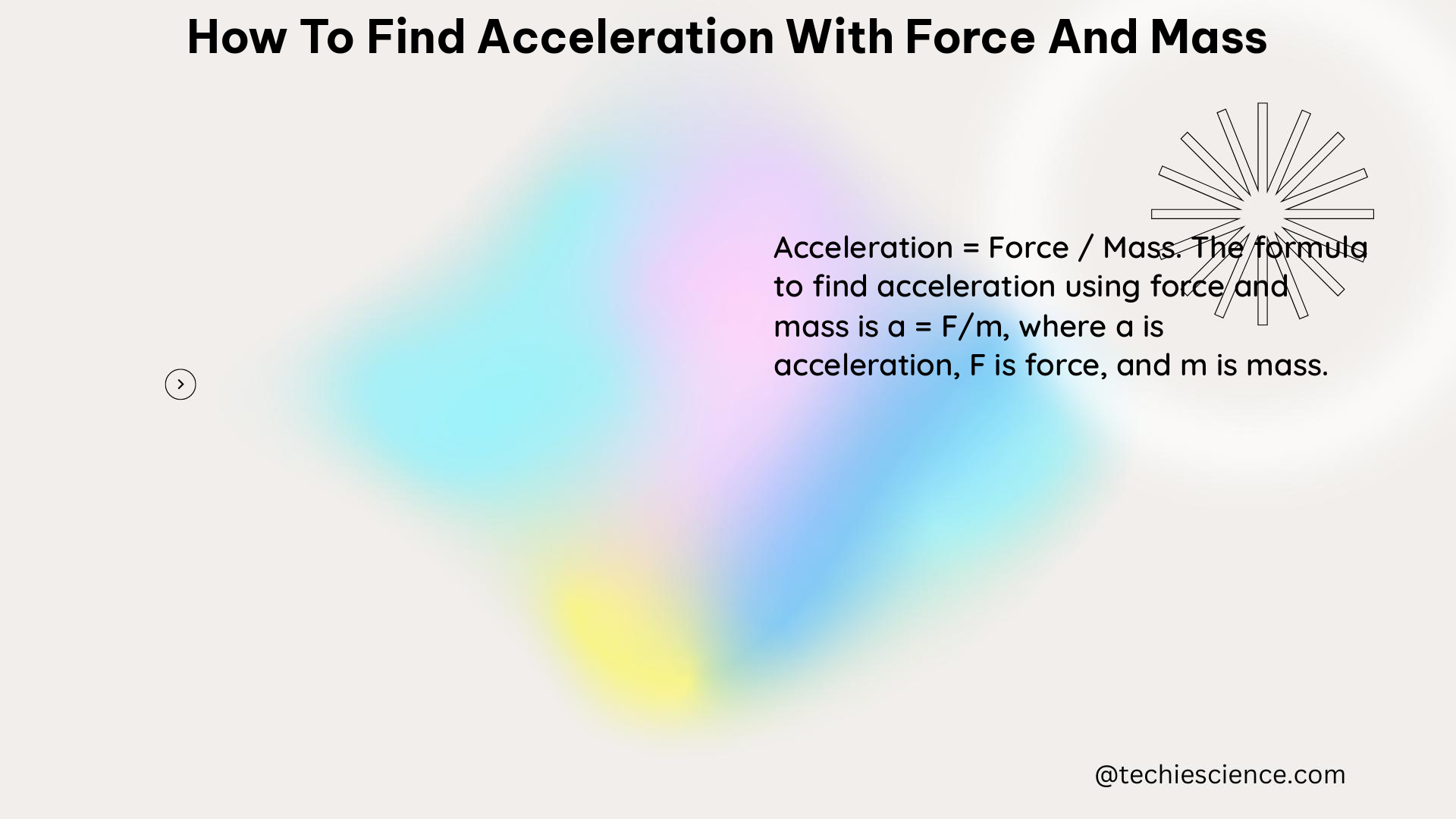
The formula for calculating acceleration using force and mass is:

a = F / m

Where:
– a is the acceleration (in meters per second squared, m/s²)
– F is the force (in Newtons, N)
– m is the mass (in kilograms, kg)

This formula is derived from Newton’s second law of motion, which states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass.

Theorem: Newton’s Second Law of Motion

The second law of motion states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. Mathematically, this can be expressed as:

F_net = m * a

Where:
– F_net is the net force acting on the object (in Newtons, N)
– m is the mass of the object (in kilograms, kg)
– a is the acceleration of the object (in meters per second squared, m/s²)

Rearranging this equation, we can derive the formula for calculating acceleration:

a = F_net / m

This is the fundamental equation we will use to find the acceleration of an object given the force and mass.

Calculating Acceleration: Examples and Numerical Problems

Let’s explore some examples and numerical problems to demonstrate how to apply the acceleration formula.

Example 1: Calculating Acceleration with a Given Force and Mass

Suppose we have a force of 50 N acting on an object with a mass of 10 kg. What is the acceleration of the object?

Using the formula:
a = F / m
a = 50 N / 10 kg
a = 5 m/s²

Therefore, the acceleration of the object is 5 meters per second squared (m/s²).

Numerical Problem 1

A force of 80 N is applied to an object with a mass of 20 kg. Calculate the acceleration of the object.

Given:
– Force, F = 80 N
– Mass, m = 20 kg

Using the formula:
a = F / m
a = 80 N / 20 kg
a = 4 m/s²

The acceleration of the object is 4 meters per second squared (m/s²).

Example 2: Accounting for Friction

In the previous examples, we assumed that there were no other forces acting on the object besides the applied force. However, in real-world scenarios, there are often additional forces, such as friction, that can affect the acceleration.

Let’s consider an example where a force of 50 N is applied to an object with a mass of 10 kg, and there is a frictional force of 10 N acting on the object.

To calculate the acceleration, we need to first find the net force acting on the object by subtracting the frictional force from the applied force:

F_net = F_applied - F_friction
F_net = 50 N - 10 N
F_net = 40 N

Now, we can use the net force to calculate the acceleration:

a = F_net / m
a = 40 N / 10 kg
a = 4 m/s²

In this case, the frictional force reduces the acceleration of the object from 5 m/s² (as calculated in the previous example) to 4 m/s².

Numerical Problem 2

An object with a mass of 15 kg is subjected to a force of 60 N. If there is a frictional force of 8 N acting on the object, calculate the acceleration of the object.

Given:
– Force, F_applied = 60 N
– Mass, m = 15 kg
– Frictional force, F_friction = 8 N

Step 1: Calculate the net force acting on the object.
F_net = F_applied - F_friction
F_net = 60 N - 8 N
F_net = 52 N

Step 2: Calculate the acceleration using the net force.
a = F_net / m
a = 52 N / 15 kg
a = 3.47 m/s²

Therefore, the acceleration of the object is approximately 3.47 meters per second squared (m/s²).

Factors Affecting Acceleration

While the formula a = F/m provides a straightforward way to calculate acceleration, there are other factors that can influence the acceleration of an object. These factors include:

1. Friction: As demonstrated in the previous examples, the presence of frictional forces can reduce the net force acting on the object, leading to a lower acceleration.

2. Air resistance (drag): When an object moves through a fluid, such as air, it experiences a force called air resistance or drag. This force can significantly affect the acceleration, especially at high speeds.

3. Inclined surfaces: When an object is placed on an inclined surface, the acceleration will depend on the angle of the incline and the coefficient of friction between the object and the surface.

4. Tension and normal forces: In more complex systems, such as pulleys or inclined planes, the acceleration may also depend on the tension in ropes or cables, as well as the normal force acting on the object.

To account for these additional factors, you may need to modify the acceleration formula or use more advanced techniques, such as free-body diagrams and the application of Newton’s laws of motion.

Advanced Concepts and Applications

As you delve deeper into the study of classical mechanics, you may encounter more advanced concepts and applications related to finding acceleration using force and mass. Some of these include:

1. Circular motion: When an object is moving in a circular path, the acceleration can be divided into two components: tangential acceleration and centripetal acceleration. The formula for calculating these accelerations involves the force, mass, and the radius of the circular path.

2. Rotational dynamics: In the case of rotational motion, the acceleration is related to the torque acting on the object and its moment of inertia, rather than just the force and mass.

3. Impulse and momentum: The relationship between force, time, and the change in momentum can be used to calculate the acceleration of an object, especially in situations involving collisions or sudden changes in motion.

4. Projectile motion: When an object is launched with an initial velocity and experiences only the force of gravity, the acceleration due to gravity can be used to predict the object’s trajectory and various properties, such as the maximum height and range.

These advanced topics build upon the fundamental principles of finding acceleration using force and mass, and they are essential for understanding more complex physical systems and phenomena.

Conclusion

In this comprehensive guide, we have explored the fundamental formula for calculating acceleration using force and mass, as well as the impact of additional factors like friction and air resistance. By working through examples and numerical problems, you should now have a solid understanding of how to apply this formula in various scenarios.

Remember, the ability to accurately determine the acceleration of an object is a crucial skill in classical mechanics and is essential for understanding the motion of objects in the physical world. As you continue your studies in physics, be sure to practice applying the acceleration formula and exploring the more advanced concepts and applications discussed in this guide.

Reference:

1. Calculating Acceleration with Force and Mass
2. Newton’s Second Law of Motion
3. Calculating Horizontal Acceleration in Systems with Friction