Summary
Determining the acceleration of an electron is a fundamental task in the field of physics. This comprehensive guide will walk you through the step-by-step process of calculating the acceleration of an electron, covering both theoretical and experimental approaches. We’ll delve into the underlying physics principles, relevant formulas, and practical examples to provide you with a thorough understanding of this topic.
Understanding the Fundamentals
Charge and Mass of an Electron
The charge of an electron is a fundamental constant, denoted as e, with a value of 1.6 × 10^-19 C. The mass of an electron, denoted as m, is also a constant with a value of 9.11 × 10^-31 kg.
Force on an Electron in an Electric Field
The force acting on an electron in an electric field is given by the equation:
F = q × E
where F is the force on the electron, q is the charge of the electron, and E is the electric field strength.
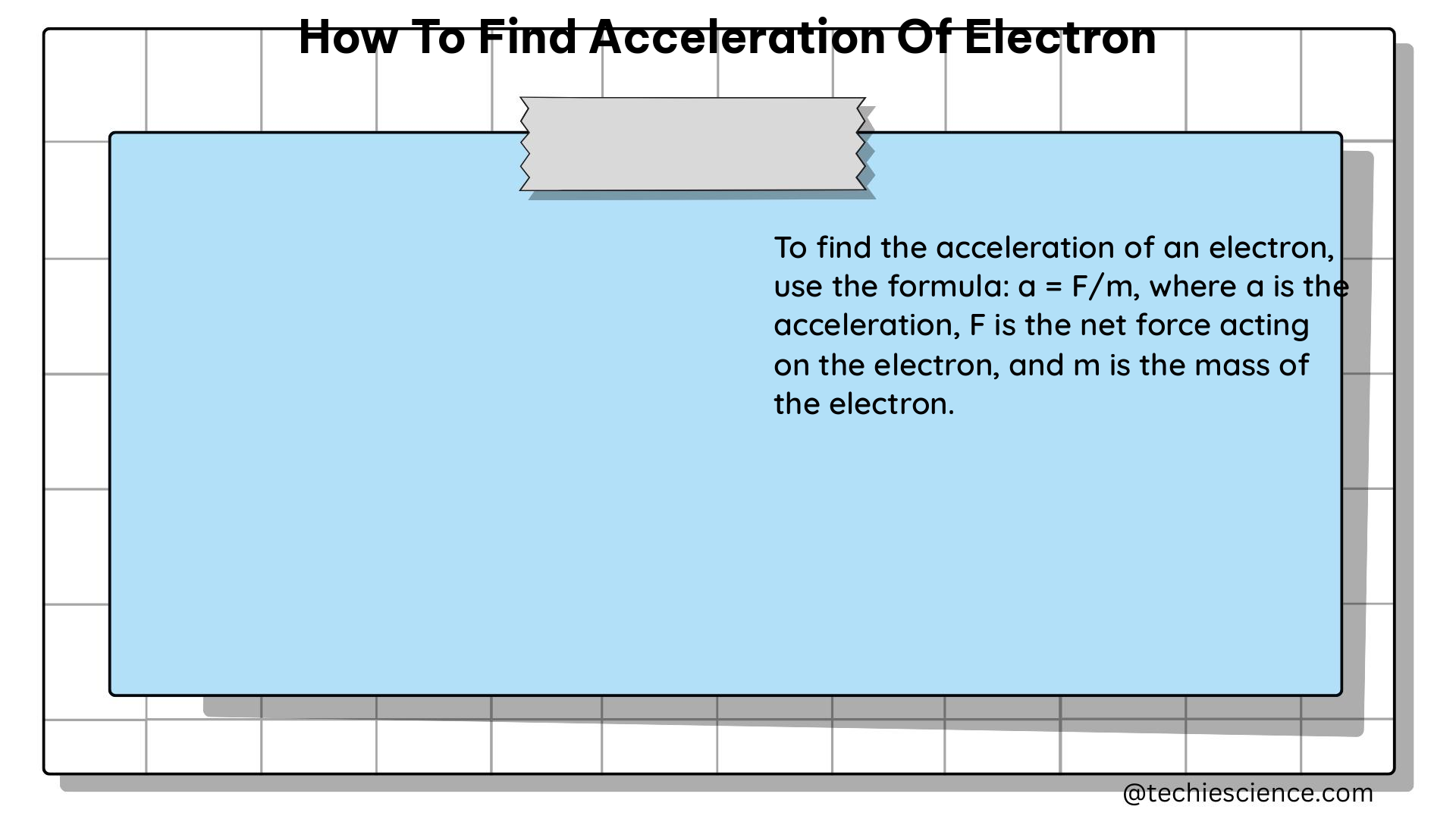
Newton’s Second Law
Once we have the force acting on the electron, we can use Newton’s second law to calculate the acceleration:
a = F / m
where a is the acceleration of the electron, F is the force, and m is the mass of the electron.
Calculating Acceleration in an Electric Field
Let’s consider an example where an electron is placed in an electric field with a magnitude of 756 N/C.
-
Calculate the force on the electron:
F = q × E
F = (1.6 × 10^-19 C) × (756 N/C)
F = 1.21 × 10^-16 N -
Calculate the acceleration of the electron:
a = F / m
a = (1.21 × 10^-16 N) / (9.11 × 10^-31 kg)
a = 1.33 × 10^14 m/s^2
Therefore, the acceleration of the electron in this electric field is 1.33 × 10^14 m/s^2, with the direction of acceleration being opposite to the direction of the electric field.
Experimental Determination of Electron Acceleration
In a laboratory setting, the acceleration of an electron can be determined experimentally by measuring the radius of its circular path in a magnetic field. This method utilizes the equation:
(m × v^2) / r = q × v × B
where m is the mass of the electron, v is its velocity, r is the radius of its path, q is the charge of the electron, and B is the magnetic field strength.
Rearranging the equation, we can solve for the acceleration:
a = q × B × r / m
Let’s consider an example where the radius of the electron’s path is measured to be 0.1 m, and the magnetic field strength is 1 T.
- Calculate the acceleration of the electron:
a = q × B × r / m
a = (1.6 × 10^-19 C) × (1 T) × (0.1 m) / (9.11 × 10^-31 kg)
a = 1.76 × 10^11 m/s^2
Therefore, the acceleration of the electron in this magnetic field is 1.76 × 10^11 m/s^2.
Additional Considerations
- The acceleration of an electron can also be affected by other forces, such as gravitational and centrifugal forces, depending on the specific experimental setup.
- In the case of relativistic electrons, the mass of the electron becomes dependent on its velocity, and the equations need to be modified accordingly.
- The experimental determination of electron acceleration can be used to measure the charge-to-mass ratio (e/m) of the electron, which is a fundamental constant in physics.
Conclusion
Determining the acceleration of an electron is a crucial step in understanding the behavior of charged particles in electric and magnetic fields. By applying the principles of electromagnetism and Newton’s laws of motion, we can calculate the acceleration of an electron both theoretically and experimentally. This guide has provided you with the necessary tools and examples to confidently tackle problems related to the acceleration of electrons.
References
- Vaia, “Physics Principles with Applications,” 7th edition, Chapter 16: Electric Charge and Electric Field, Problem 23P.
- Socratic, “An electron is released from rest in a uniform electric of magnitude 2.00×104 N/C. Calculate the acceleration of the electron (Ignore gravitation)?”
- Purdue University, “Physics 342 Laboratory: The Determination of e/m for Electrons”
- YouTube, “Finding the acceleration of an electron.”
- Lumen Learning, “Relativistic Energy | Physics.”

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.