How to Find Acceleration Due to Gravity Using a Pendulum: A Comprehensive Guide

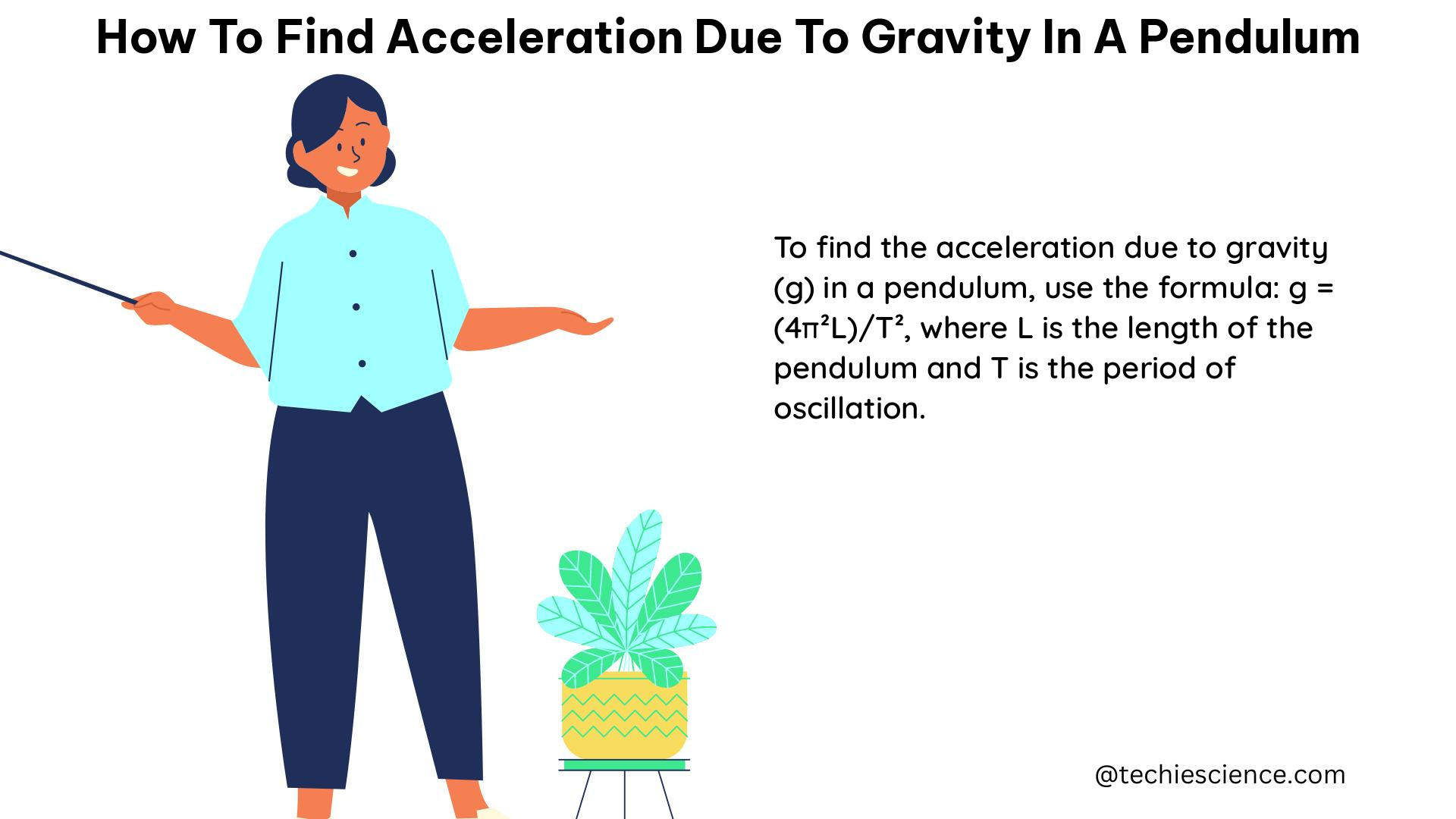
Summary

Determining the acceleration due to gravity (g) using a simple pendulum is a classic experiment in physics. By measuring the period of oscillation and the length of the pendulum, you can calculate the value of g with high accuracy. This comprehensive guide will walk you through the step-by-step process, providing detailed explanations, formulas, examples, and practical tips to help you master this technique.

Constructing a Simple Pendulum

The first step in finding the acceleration due to gravity using a pendulum is to set up a simple pendulum. This involves attaching a mass (such as a ball or weight) to a lightweight, rigid rod or string, and suspending it from a fixed point. The mass should be able to swing freely, with minimal friction or air resistance.

When the pendulum is displaced from its equilibrium position and released, it will oscillate back and forth due to the force of gravity. The time it takes for the pendulum to complete one full cycle of oscillation is known as the period (T).

Measuring the Period of Oscillation

Accurately measuring the period of the pendulum is crucial for determining the acceleration due to gravity. There are several methods you can use to measure the period:

1. Stopwatch Method: Displace the pendulum and start a stopwatch as it passes through the equilibrium position. Count the number of oscillations (N) and stop the stopwatch when the pendulum has completed N cycles. The period is then calculated as T = t/N, where t is the total time measured.

2. Photogate Method: Use a photogate or light sensor to detect the passage of the pendulum through a specific point. The time between successive passages can be measured electronically, providing a direct measurement of the period.

3. Video Analysis: Record the pendulum’s motion with a high-speed camera or smartphone, and then analyze the video frame by frame to determine the period of oscillation.

Regardless of the method used, it’s important to take multiple measurements and calculate the average period to improve the accuracy of your results.

The Pendulum Equation and Solving for Acceleration Due to Gravity

The relationship between the period of a simple pendulum, its length, and the acceleration due to gravity is given by the pendulum equation:

T = 2π√(L/g)

Where:
– T is the period of oscillation (in seconds)
– L is the length of the pendulum (in meters)
– g is the acceleration due to gravity (in m/s²)

To solve for the acceleration due to gravity (g), we can rearrange the equation:

g = 4π²L/T²

By substituting the measured values of the period (T) and the length of the pendulum (L) into this equation, you can calculate the value of the acceleration due to gravity (g).

Example Calculation

Suppose you have a pendulum with a length of 1.2 meters, and the measured period of oscillation is 2.2 seconds. Using the pendulum equation, you can calculate the acceleration due to gravity as follows:

g = 4π²L/T²
g = 4 × (3.14159)²× 1.2 / (2.2)²
g = 9.81 m/s²

This result is very close to the accepted value of the acceleration due to gravity on Earth, which is approximately 9.81 m/s².

Improving Accuracy

To increase the accuracy of your measurements and the calculated value of the acceleration due to gravity, you can consider the following techniques:

1. Use a Longer Pendulum: The period of a pendulum is proportional to the square root of its length. Using a longer pendulum will result in a longer period, making it easier to measure accurately.

2. Increase the Number of Oscillations: By allowing the pendulum to oscillate for a longer time and counting the number of cycles, you can estimate the period more precisely.

3. Minimize Friction and Air Resistance: Ensure that the pendulum is swinging freely, without any significant friction or air resistance that could affect its motion. This can be achieved by using a low-friction suspension point and minimizing air currents in the experiment area.

4. Repeat Measurements: Take multiple measurements of the period and length, and calculate the average values to reduce the impact of random errors.

5. Use Higher-Precision Instruments: Employ digital stopwatches, micrometers, or other high-precision measurement tools to obtain more accurate values for the period and length of the pendulum.

Practical Considerations and Limitations

While the pendulum method is a reliable way to determine the acceleration due to gravity, there are a few practical considerations and limitations to keep in mind:

1. Pendulum Amplitude: The pendulum equation assumes small-angle oscillations, where the amplitude of the swing is less than about 10 degrees. Larger amplitudes can introduce errors due to the non-linear nature of the motion.

2. Air Resistance and Friction: As mentioned earlier, air resistance and friction can affect the pendulum’s motion and introduce errors in the period measurement. Minimizing these effects is crucial for accurate results.

3. Pendulum Length Measurement: Accurately measuring the length of the pendulum is important, as the period is proportional to the square root of the length. Any errors in the length measurement will be amplified in the final calculation of g.

4. Environmental Factors: Changes in temperature, humidity, or air pressure can slightly affect the value of the acceleration due to gravity, which should be considered when interpreting the results.

5. Comparison to Other Methods: While the pendulum method is a classic and widely used technique, it is not the only way to determine the acceleration due to gravity. Other methods, such as using a free-fall experiment or a gravimeter, may provide additional insights or higher accuracy in certain situations.

Conclusion

Determining the acceleration due to gravity using a simple pendulum is a fundamental experiment in physics that allows you to apply your understanding of classical mechanics and gain hands-on experience with experimental techniques. By following the step-by-step process outlined in this guide, you can accurately measure the value of g and deepen your appreciation for the fundamental forces that govern the physical world around us.

References

1. Measuring acceleration due to gravity using a pendulum – YouTube
2. Gravity: Notes: Pendulum Measurements – Pamela Burnley UNLV
3. 16.4 The Simple Pendulum | Texas Gateway
4. How to Calculate an Acceleration Due to Gravity Using the Pendulum Equation – Study.com
5. Measuring gravitational acceleration with a pendulum – Don Cross