How to Estimate Magnetic Energy in Fusion Reactors

Estimating the magnetic energy in fusion reactors is a crucial step in understanding the performance and potential of these advanced energy systems. This comprehensive guide will provide you with the necessary tools and techniques to accurately estimate the magnetic energy in fusion reactors, with a focus on the International Thermonuclear Experimental Reactor (ITER) as a case study.

Understanding the Magnetic Energy Formula

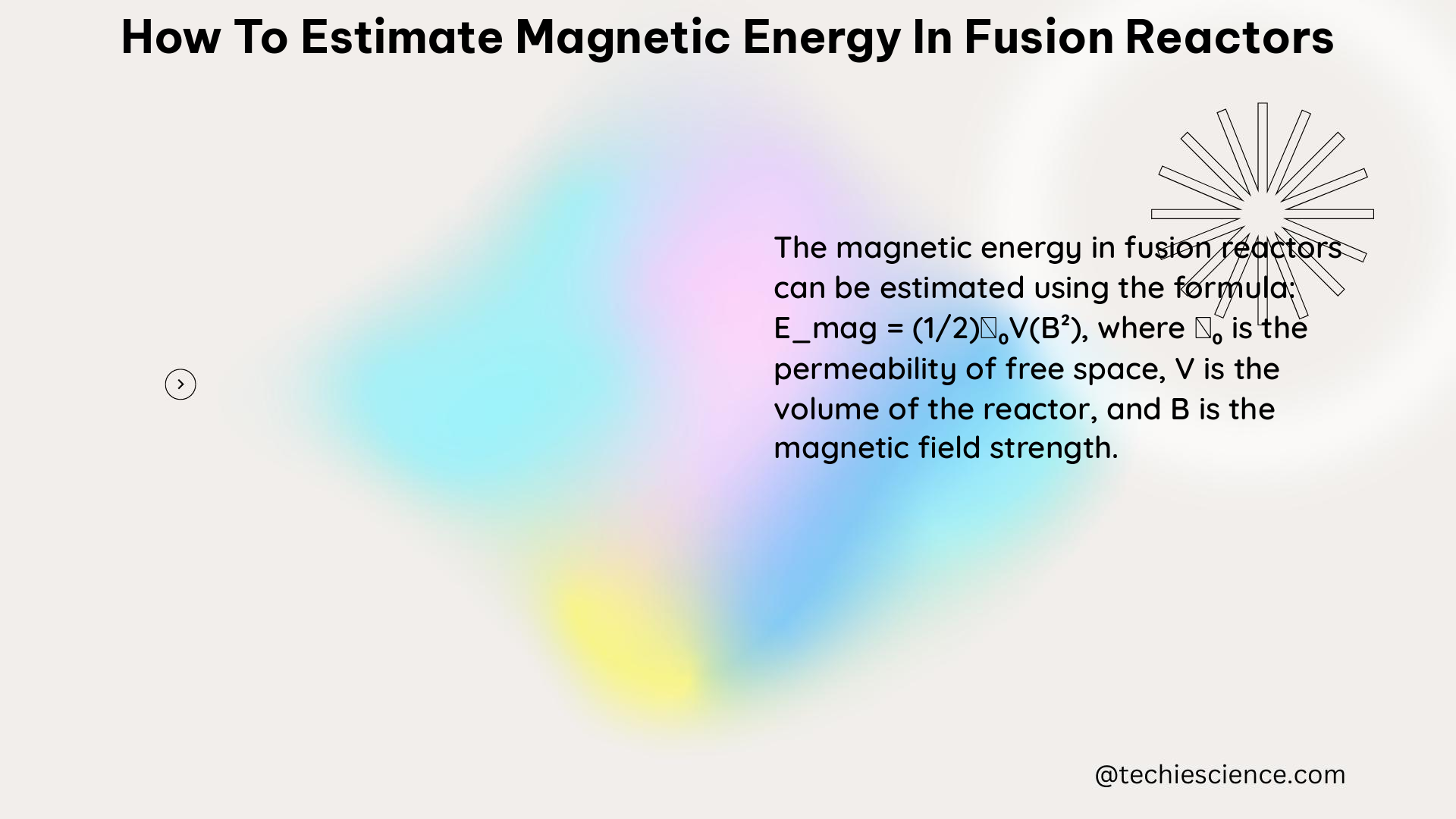
The magnetic energy (U) in a fusion reactor can be calculated using the following formula:

U = (1/2) × B^2 × V

Where:
– B is the magnetic field strength, measured in Tesla (T)
– V is the volume of the plasma, measured in cubic meters (m^3)

This formula is based on the principle that the magnetic energy stored in a volume is proportional to the square of the magnetic field strength and the volume of the confined plasma.

Determining the Magnetic Field Strength

The magnetic field strength in fusion reactors can vary depending on the specific design and stage of operation. In the case of ITER, the magnetic field strength is expected to reach up to 13 Tesla.

To calculate the magnetic field strength, you can use the following formula:

B = μ₀ × I / (2 × π × r)

Where:
– μ₀ is the permeability of free space (4π × 10^-7 H/m)
– I is the current in the magnetic coils, measured in Amperes (A)
– r is the radius of the magnetic coil, measured in meters (m)

By inputting the relevant parameters for ITER, you can determine the magnetic field strength.

Estimating the Plasma Volume

In tokamak-style fusion reactors, the plasma is typically confined within a toroidal (doughnut-shaped) vacuum vessel. To estimate the volume of the plasma, you need to consider the major and minor radii of the torus, as well as the aspect ratio (the ratio of the major radius to the minor radius).

The formula for the plasma volume (V) in a tokamak reactor is:

V = (1/2) × π^2 × r_major × r_minor^2 × aspect_ratio

Where:
– r_major is the major radius of the torus, measured in meters (m)
– r_minor is the minor radius of the torus, measured in meters (m)
– aspect_ratio is the ratio of the major radius to the minor radius (dimensionless)

For ITER, the major radius is 6.2 meters, the minor radius is 2 meters, and the aspect ratio is 3.1.

Calculating the Magnetic Energy

Now that we have the magnetic field strength (B) and the plasma volume (V), we can calculate the magnetic energy (U) using the formula:

U = (1/2) × B^2 × V

Plugging in the values for ITER:
– B = 13 T
– V = (1/2) × π^2 × 6.2 m × (2 m)^2 × 3.1 = 251.6 m^3

U = (1/2) × (13 T)^2 × 251.6 m^3
U = 22.5 GJ

Therefore, the estimated magnetic energy in ITER is 22.5 Gigajoules (GJ).

Considering Plasma Pressure and Beta Value

In addition to the magnetic energy, it is important to consider the plasma pressure and the beta value (the ratio of the plasma pressure to the magnetic pressure) in fusion reactors. These values can affect the stability and confinement of the plasma, and therefore the overall performance of the fusion reactor.

The plasma pressure can be estimated using the ideal gas law:

p = n × k × T

Where:
– p is the plasma pressure, measured in Pascals (Pa)
– n is the plasma density, measured in particles per cubic meter (m^-3)
– k is the Boltzmann constant (1.38 × 10^-23 J/K)
– T is the plasma temperature, measured in Kelvin (K)

The beta value (β) can be calculated as:

β = (2 × plasma_pressure) / (B^2)

Where:
– plasma_pressure is measured in Pascals (Pa)
– B is the magnetic field strength, measured in Tesla (T)

For ITER, the plasma pressure is expected to reach up to 5.3 × 10^6 Pa. Using this value, we can calculate the beta value as:

β = (2 × 5.3 × 10^6 Pa) / (13 T)^2
β = 0.07

Therefore, the estimated beta value in ITER is 0.07.

Conclusion

Estimating the magnetic energy in fusion reactors is a crucial step in understanding the performance and potential of these advanced energy systems. By using the formulas and techniques outlined in this guide, you can accurately calculate the magnetic energy, plasma pressure, and beta value for fusion reactors like ITER. These values provide important insights that can help researchers and engineers optimize the design and operation of fusion reactors.

References

1. Maisonnier, D., Cook, I., Sardain, P., Andreani, R., Di Pace, L., Forrest, R., Giancarli, L., Hermsmeyer, S., Norajitra, P., Taylor, N., et al. (2005). A conceptual study of commercial fusion power plants: Final report of the European Fusion Power Plant Conceptual Study (PPCS). European Fusion Development Agreement.
2. Turnbull, D. (2013). Identifying new saturation mechanisms hindering the development of plasma-based laser amplifiers utilizing stimulated Raman backscattering. Ph.D. Dissertation. Princeton University, USA.
3. Courtesy of Bernard Bigot (director of ITER). Presentation at the 2015 Annual Meeting of the Fusion Power Associates, 16 December 2015.