How to Estimate Energy Production in Fusion Reactors: A Comprehensive Guide

Estimating the energy production in fusion reactors requires a deep understanding of the underlying physics principles and mathematical models. This comprehensive guide will walk you through the step-by-step process of calculating the energy output in a fusion reactor, covering everything from the fundamental nuclear fusion reactions to the practical considerations of plasma confinement and reactor design.

Understanding Nuclear Fusion Reactions

At the heart of fusion energy production is the process of nuclear fusion, where two light nuclei are combined to form a heavier nucleus, releasing a significant amount of energy in the process. The energy released in a fusion reaction can be calculated using Einstein’s mass-energy equivalence formula, E = mc^2, where E is the energy released, m is the mass difference between the reactant and product nuclei, and c is the speed of light.

For example, in the fusion of deuterium (D) and tritium (T) nuclei, the reactant nuclei have a combined mass of 5.008249 u, while the product nucleus (helium-4) has a mass of 4.002603 u. The mass difference is 1.005646 u, which can be plugged into the formula to calculate the energy released:

E = (1.005646 u) × (1.66054 × 10^-27 kg/u) × (3.00 × 10^8 m/s)^2
E = 3.37 × 10^-12 J

This energy is released in a single fusion reaction, which typically occurs on the timescale of 10^-20 seconds. To calculate the power produced in a single fusion reaction, we divide the energy by the reaction time:

P = E/t
P = (3.37 × 10^-12 J) / (10^-20 s)
P = 3.37 × 10^8 W

Estimating the Number of Fusion Reactions

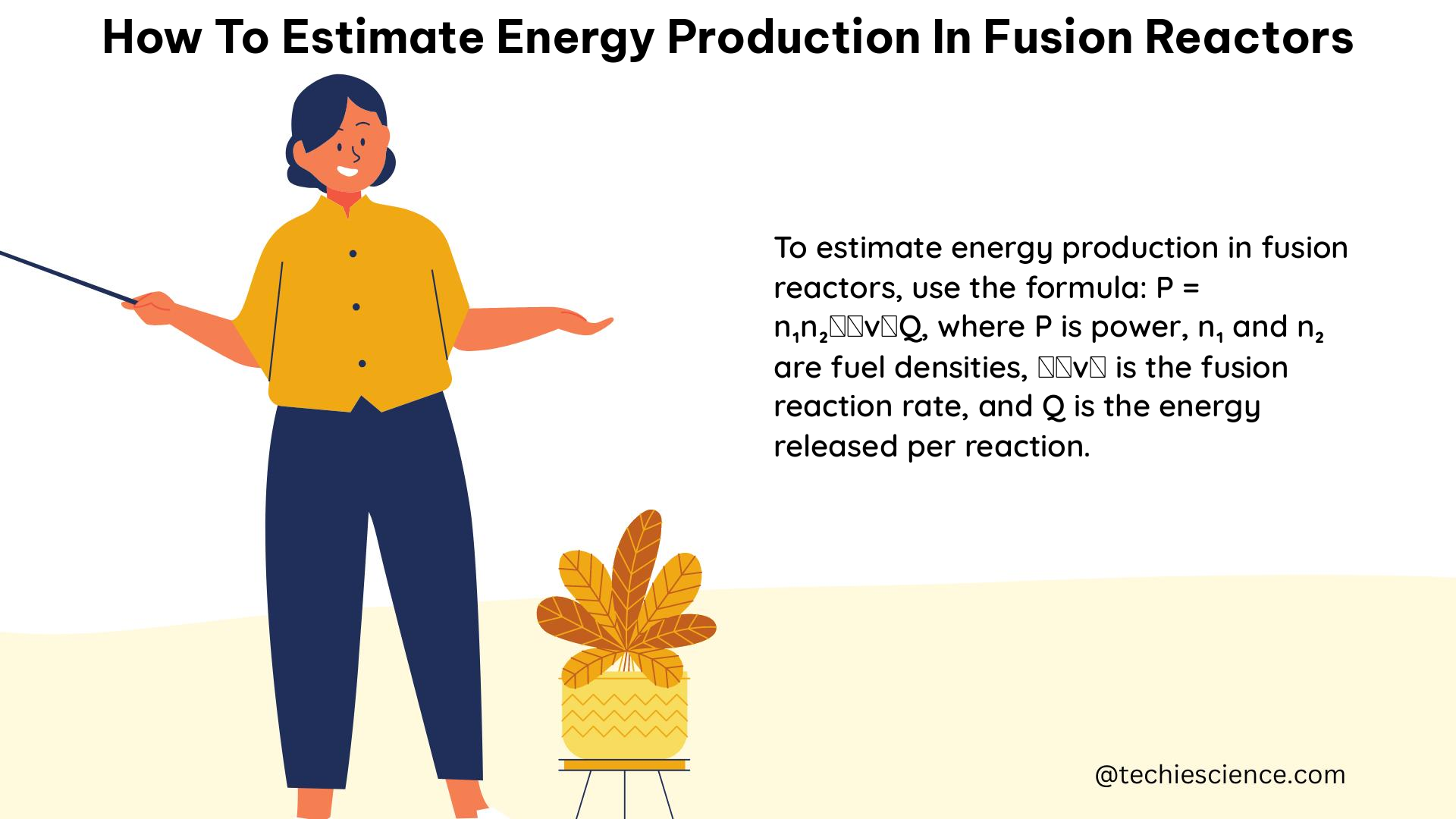
To estimate the total energy production in a fusion reactor, we need to consider the number of fusion reactions that occur per second. This is determined by the plasma density, the volume of the plasma, and the confinement time.

The plasma density is typically measured in particles per cubic meter, and the volume of the plasma is determined by the size of the reactor. The confinement time is the amount of time that the plasma is confined in the reactor, and it is typically measured in seconds.

For example, let’s assume a fusion reactor with the following parameters:
– Plasma density: 10^20 particles/m^3
– Plasma volume: 1000 m^3
– Confinement time: 1 second

The number of fusion reactions that occur per second can be calculated as:

N = n × V × t
N = (10^20 particles/m^3) × (1000 m^3) × (1 s)
N = 10^23 reactions

Calculating the Total Energy Production

Now that we have the power produced in a single fusion reaction and the number of fusion reactions per second, we can calculate the total energy production in the reactor:

E = P × N
E = (3.37 × 10^8 W) × (10^23)
E = 3.37 × 10^31 J

This is the energy production in a single second. To calculate the energy production per hour, we need to multiply this value by the number of seconds in an hour (3600 s):

E = (3.37 × 10^31 J) × (3600 s)
E = 1.22 × 10^34 J

Factors Affecting Energy Production

The energy production in a fusion reactor can be influenced by several factors, including:

1. Plasma Density: A higher plasma density will result in more fusion reactions per unit volume, leading to increased energy output.
2. Plasma Volume: A larger plasma volume will accommodate more fusion reactions, contributing to higher overall energy production.
3. Confinement Time: Longer confinement times allow for more fusion reactions to occur, increasing the energy output.
4. Fusion Reaction Cross-section: The probability of fusion reactions occurring is determined by the reaction cross-section, which is influenced by factors like temperature and fuel composition.
5. Reactor Design: The design of the fusion reactor, including the magnetic confinement system and the materials used, can impact the efficiency and energy output of the system.

Practical Considerations and Challenges

Estimating the energy production in fusion reactors is not a straightforward task, as there are numerous practical considerations and challenges that must be addressed:

1. Plasma Stability and Confinement: Maintaining a stable and well-confined plasma is crucial for achieving high energy output, but it requires advanced magnetic confinement systems and precise control of plasma parameters.
2. Plasma Heating and Ignition: Heating the plasma to the required temperatures (typically in the range of 100-200 million degrees Celsius) and achieving ignition, where the fusion reactions are self-sustaining, is a significant technical challenge.
3. Fuel Cycle and Tritium Breeding: The fusion reaction between deuterium and tritium is the most promising for current fusion reactor designs, but the limited availability of tritium necessitates the development of efficient tritium breeding systems.
4. Materials and Engineering Challenges: The extreme conditions inside a fusion reactor, including high temperatures, intense neutron fluxes, and corrosive plasma, pose significant materials and engineering challenges.
5. Scaling and Commercialization: Transitioning from experimental fusion reactors to commercially viable power plants requires overcoming various technical, economic, and regulatory hurdles.

Conclusion

Estimating the energy production in fusion reactors is a complex task that requires a deep understanding of nuclear physics, plasma physics, and engineering principles. By following the step-by-step approach outlined in this guide, you can develop a comprehensive understanding of the factors that influence energy output and the practical challenges that must be addressed to realize the full potential of fusion energy.

References

1. How to Calculate the Power Produced in Nuclear Fusion – Study.com
2. Fusion Book.pdf – NUCLEUS information resources
3. Measuring Progress in Fusion Energy: The Triple Product
4. Fusion Energy: The Path to Sustainable Power – IAEA
5. Fusion Energy: Challenges and Opportunities – MIT Energy Initiative