Summary
Determining the velocity of a fluid under pressure is a fundamental concept in fluid dynamics. This comprehensive guide will walk you through the step-by-step process of using Bernoulli’s equation to calculate the velocity of a fluid at different points in a flow field, considering factors such as pressure, height, and cross-sectional area. With detailed explanations, formulas, examples, and references, this article aims to provide a thorough understanding of this essential topic for physics students.
Understanding Bernoulli’s Equation
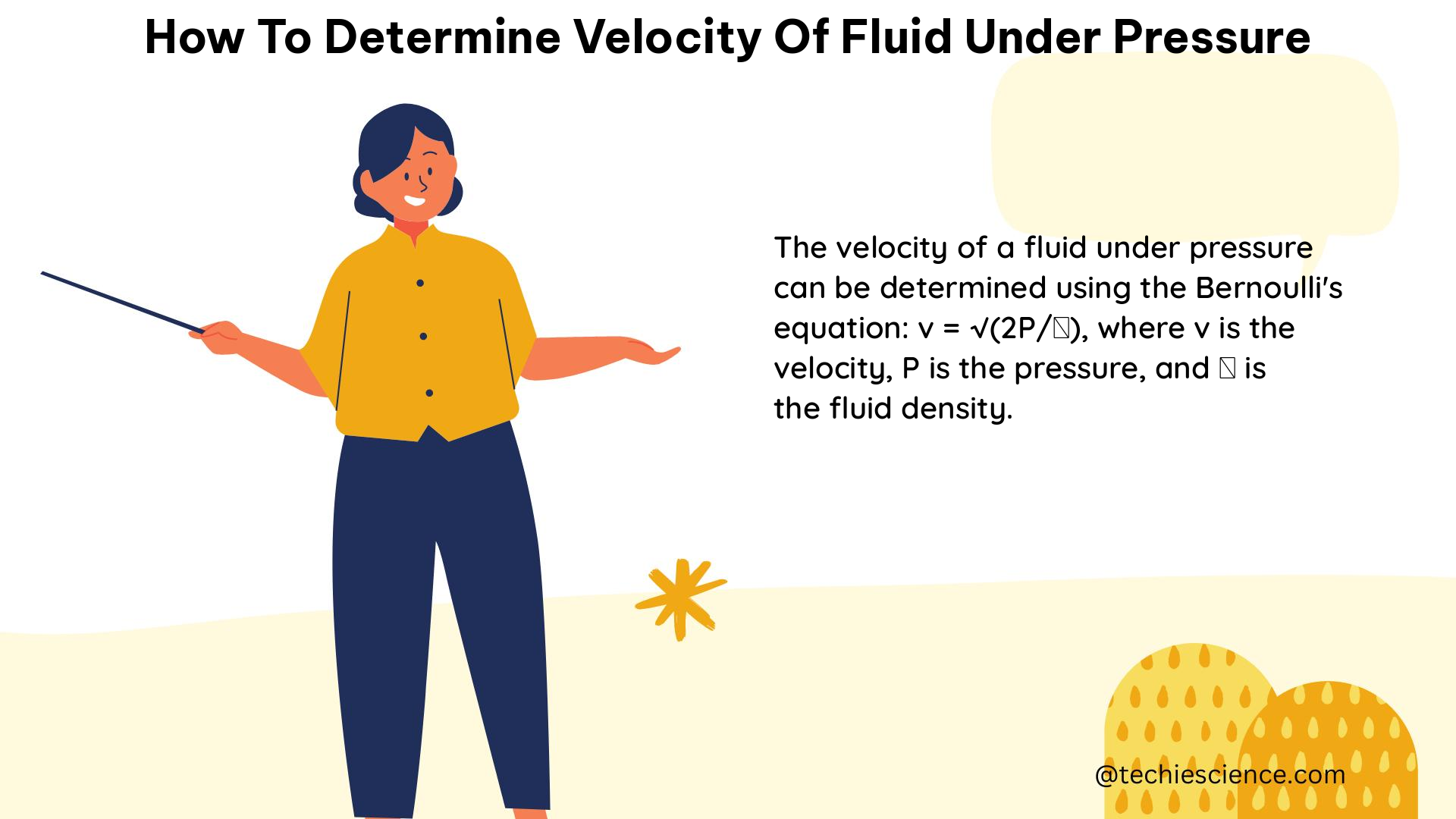
Bernoulli’s equation is a fundamental principle in fluid dynamics that relates the pressure, velocity, and height of a fluid at two different points in a flow field. The equation can be expressed as:
$P_1 + \frac{1}{2}\rho v_1^2 + \rho gh_1 = P_2 + \frac{1}{2}\rho v_2^2 + \rho gh_2$
Where:
– $P_1$ and $P_2$ are the pressures at the two points
– $\rho$ is the density of the fluid
– $v_1$ and $v_2$ are the velocities of the fluid at the two points
– $g$ is the acceleration due to gravity
– $h_1$ and $h_2$ are the heights of the fluid at the two points
This equation can be used to determine the velocity of a fluid at a specific point, given the values of the other parameters.
Step-by-Step Guide to Determining Fluid Velocity
-
Identify the Relevant Parameters: Determine the values of the height ($h$), cross-sectional area of the pipe ($A$), and pressure ($P$) at the points of interest. Remember that the gauge pressure ($P_g$) is the difference between the total pressure ($P_t$) and the atmospheric pressure ($P_{atm}$). At the point where the fluid is exposed to the atmosphere, the gauge pressure is 0, and the total pressure is equal to the atmospheric pressure.
-
Write the Bernoulli Equation: Set up the Bernoulli equation for the two points in the flow field:
$P_1gh_1 + P_1 + \frac{1}{2}\rho v_1^2 = P_2gh_2 + P_2 + \frac{1}{2}\rho v_2^2$
This equation provides an initial relation between the velocities at the two points.
- Use the Equation of Continuity: If the Bernoulli equation alone is not sufficient to determine the velocities, you can use the equation of continuity, which states that the volumetric flow rate is constant throughout the flow field:
$A_1v_1 = A_2v_2$
This equation provides a second relation between the velocities at the two points.
- Solve the Equations: By simultaneously solving the Bernoulli equation and the equation of continuity, you can determine the unknown fluid velocities at the two points.
Example Calculation
Let’s consider a practical example to illustrate the process:
Suppose we have a pipe with an inlet and an outlet, and we want to find the velocity of the fluid at the inlet ($v_2$) and the outlet ($v_1$). We know the following:
- The top of the pipe is 10 m high and exposed to the atmosphere, so the total pressure is 1 atm.
- The cross-sectional area at the pipe’s outlet is $A_1 = \pi r_1^2 = 0.09\pi$ cm^2.
- At the pipe’s inlet, the gauge pressure is 200 Pa, so the total pressure is $P_{t2} = 1$ atm + 200 Pa.
- The cross-sectional area at the pipe’s inlet is $A_2 = \pi r_2^2 = 0.25\pi$ cm^2.
- The density of the fluid (water) is $\rho = 1$ kg/m^3.
Using the Bernoulli equation and the equation of continuity, we can solve for the velocities at the inlet and outlet:
$P_1gh_1 + P_1 + \frac{1}{2}\rho v_1^2 = P_2gh_2 + P_2 + \frac{1}{2}\rho v_2^2$
$A_1v_1 = A_2v_2$
Solving these equations simultaneously, we can find the values of $v_1$ and $v_2$.
Additional Considerations
- Fluid Properties: The density ($\rho$) and viscosity ($\mu$) of the fluid are important factors that can affect the velocity and flow patterns.
- Pipe Geometry: The shape and size of the pipe can also influence the fluid velocity, as changes in cross-sectional area can lead to changes in velocity according to the equation of continuity.
- Turbulence and Boundary Layers: In real-world scenarios, factors such as turbulence and boundary layer effects can complicate the analysis and require more advanced techniques, such as computational fluid dynamics (CFD) simulations.
- Limitations of Bernoulli’s Equation: Bernoulli’s equation assumes steady, inviscid, and incompressible flow, which may not always be the case in practical situations. In such cases, more comprehensive approaches, such as the Navier-Stokes equations, may be required.
Conclusion
Determining the velocity of a fluid under pressure is a fundamental concept in fluid dynamics, and Bernoulli’s equation is a powerful tool for this purpose. By following the step-by-step guide and understanding the underlying principles, you can effectively calculate the fluid velocity at different points in a flow field. Remember to consider the various factors that can influence the fluid flow, and be aware of the limitations of the Bernoulli equation when dealing with more complex scenarios.
References
- Fundamental Properties of Fluids – Introduction to Aerospace Flight Vehicles. (n.d.). Retrieved from https://eaglepubs.erau.edu/introductiontoaerospaceflightvehicles/chapter/fundamental-properties-of-fluids/
- Fluid Flow Rate – an overview | ScienceDirect Topics. (n.d.). Retrieved from https://www.sciencedirect.com/topics/engineering/fluid-flow-rate
- Bernoulli’s Equation. (n.d.). Retrieved from https://www.princeton.edu/~asmits/Bicycle_web/Bernoulli.html
- How to Use Bernoulli’s Equation to Calculate a Velocity for Fluid Exposed to Atmosphere. (n.d.). Retrieved from https://study.com/skill/learn/how-to-use-bernoullis-equation-to-calculate-a-velocity-for-fluid-exposed-to-atmosphere-explanation.html
- Flow measurement – Wikipedia. (n.d.). Retrieved from https://en.wikipedia.org/wiki/Flow_measurement

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.