Determining the velocity of electrons within molecular orbitals is a crucial aspect of understanding the behavior and properties of molecules. This comprehensive guide will delve into the theoretical background, calculation methods, quantifiable data, and practical applications of determining velocity in molecular orbitals, providing a valuable resource for physics students and researchers.
Theoretical Background
Molecular Orbital Theory
Molecular orbitals are constructed using the linear combination of atomic orbitals (LCAO) method, where the wave functions of individual atomic orbitals are combined to form new wave functions that describe the distribution of electrons within a molecule. These molecular orbitals can be classified as bonding, antibonding, or non-bonding, depending on their symmetry and the presence of nodal planes.
The energy levels of molecular orbitals can be calculated using the Schrödinger equation, and the resulting molecular orbital diagram provides a visual representation of the energy distribution of these orbitals.
Wave Functions and Energies
The wave functions of molecular orbitals describe the probability distribution of electrons within the molecule. These wave functions are solutions to the Schrödinger equation and can be used to calculate the energies of the molecular orbitals.
The energies of molecular orbitals are typically represented in a molecular orbital diagram, which shows the relative energy levels of the bonding, antibonding, and non-bonding orbitals. Understanding the energy levels of these orbitals is crucial for determining the velocity of electrons within the molecule.
Calculating Velocity
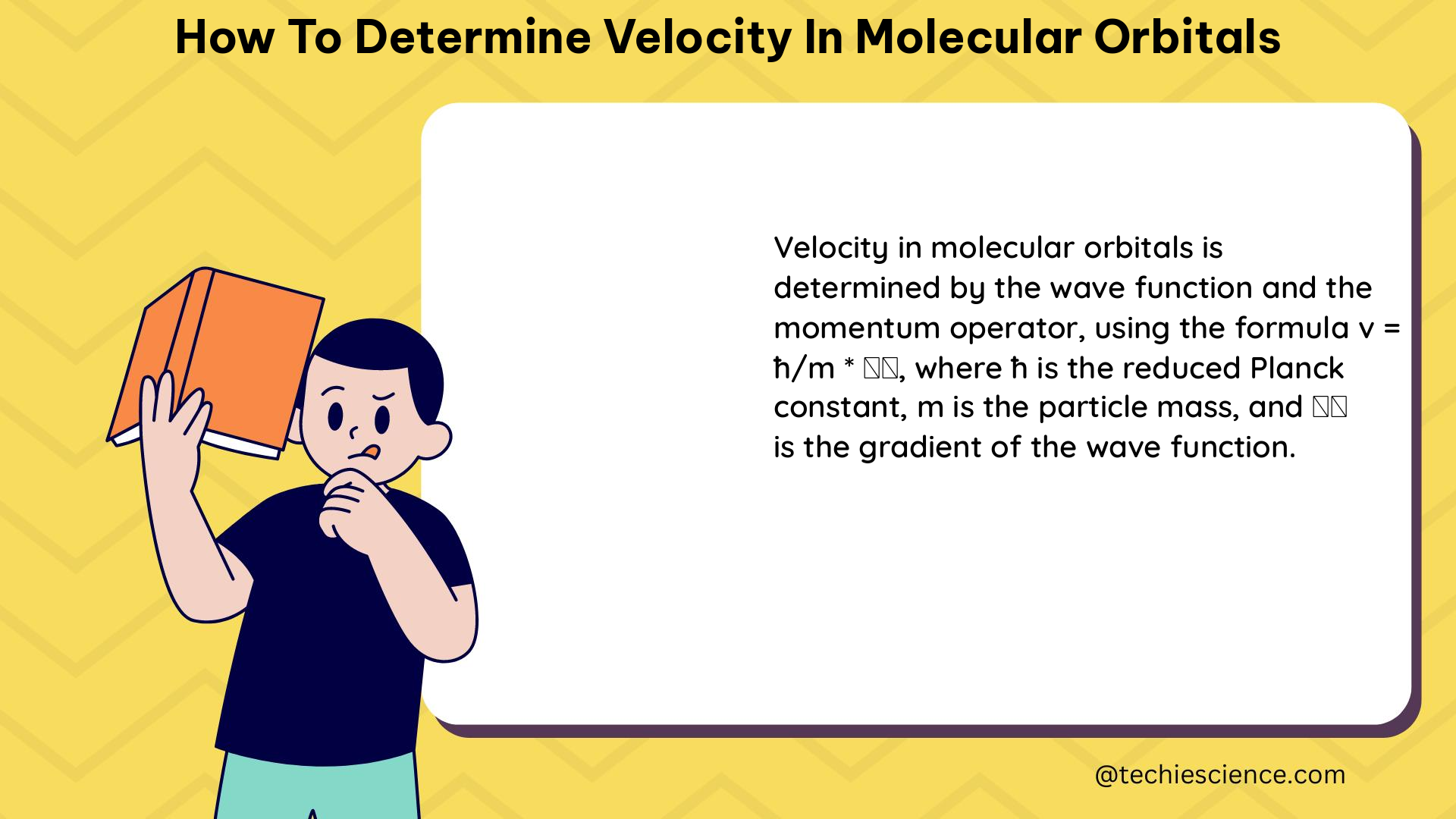
Wave Function Analysis
The velocity of electrons in a molecular orbital can be determined by analyzing the wave function of that orbital. The wave function is a mathematical description of the probability distribution of electrons in space and time, and it can be used to calculate the momentum and velocity of the electrons.
Momentum Space
The momentum density of electrons in a molecular orbital can be calculated by transforming the wave function from position space to momentum space using the Fourier transform. The momentum density is directly related to the velocity of the electrons, as it describes the distribution of electron momenta.
Experimental Techniques
Various spectroscopic techniques, such as homodyne and heterodyne methods, absorption, emission, fluorescence, and interference techniques, can be used to measure the interaction of molecules with photons or electrons. These measurements can provide information about the velocity of electrons in molecular orbitals.
Quantifiable Data
Molecular Orbital Energies
The energies of molecular orbitals can be calculated using computational methods and compared to experimental data. These energy values can be used to determine the velocity of electrons in different molecular orbitals, as the velocity is related to the energy of the orbital.
Momentum Densities
The momentum densities of electrons in molecular orbitals can be calculated and compared to experimental data. These momentum densities provide a quantifiable measure of the velocity of electrons in different molecular orbitals.
Examples and Applications
Photoelectron Spectroscopy
Photoelectron spectroscopy can be used to measure the velocity of electrons in molecular orbitals by analyzing the kinetic energy of ejected electrons. This technique provides a direct way to probe the velocity of electrons in specific molecular orbitals.
Femtochemistry
Femtochemistry involves the study of chemical reactions on the femtosecond timescale, which is relevant to understanding the velocity of electrons in molecular orbitals during chemical processes. By studying the dynamics of electrons in molecular orbitals, researchers can gain insights into the mechanisms of chemical reactions.
Technical Specifications
Software Tools
Software tools like Gaussian and Webmo can be used to calculate molecular orbitals and their energies, providing a valuable resource for determining the velocity of electrons in these orbitals.
Basis Sets
Basis sets, such as 6-311G(d,p), are used to describe the atomic orbitals that make up the molecular orbitals. The choice of basis set can significantly impact the accuracy of the calculations.
Computational Methods
Advanced computational methods, like state-averaged complete active space (SA-CASSCF) and configuration interaction (CI), are used to calculate molecular orbitals and their energies, which are essential for determining the velocity of electrons in these orbitals.
References
- Molecular Orbital Theory and Its Applications
- Molecular Orbitals in General Chemistry
- Ordering Molecular Orbitals by Energy
- Momentum Density in Molecular Orbitals
- Visualizing Molecular Orbitals
By understanding the theoretical background, calculation methods, quantifiable data, and practical applications of determining velocity in molecular orbitals, physics students and researchers can gain a comprehensive understanding of this important topic in quantum chemistry and molecular physics.

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.