Mastering the Art of Determining Velocity in Mechanical Waves

Mechanical waves are a fundamental concept in physics, and understanding how to determine their velocity is crucial for various applications, from engineering to medical diagnostics. In this comprehensive guide, we will delve into the intricacies of calculating the velocity of mechanical waves, providing you with a step-by-step approach, technical specifications, and practical examples to help you become a true expert in this field.

Understanding the Fundamentals of Mechanical Wave Velocity

The velocity of a mechanical wave is determined by the formula v = fλ, where v represents the velocity, f is the frequency, and λ is the wavelength. This relationship is a fundamental principle in wave mechanics and forms the foundation for our exploration.

Measuring Frequency (f)

The frequency of a mechanical wave is the number of cycles or oscillations that occur in one second, measured in Hertz (Hz). To determine the frequency, you can use a variety of instruments, including:

1. Frequency Counter: A frequency counter is a device that directly measures the frequency of a wave by counting the number of cycles within a specific time interval.
2. Spectrometer: A spectrometer is an instrument that analyzes the frequency spectrum of a wave, allowing you to precisely determine its frequency.

Measuring Wavelength (λ)

The wavelength of a mechanical wave is the distance between two consecutive points in the same phase of the wave, measured in meters (m). You can measure the wavelength using the following tools:

1. Ruler or Meter Stick: For larger wavelengths, you can use a ruler or meter stick to directly measure the distance between two consecutive wave crests or troughs.
2. Laser Interferometer: For smaller wavelengths, a laser interferometer can be used to measure the wavelength with high precision by analyzing the interference patterns of the wave.

Calculating Velocity (v)

Once you have determined the frequency and wavelength of the mechanical wave, you can use the formula v = fλ to calculate the velocity. The velocity is measured in meters per second (m/s).

For example, if a wave has a frequency of 2 Hz and a wavelength of 0.5 meters, the velocity can be calculated as:

v = fλ
  = 2 Hz × 0.5 m
  = 1 m/s

Therefore, the velocity of the wave is 1 m/s.

Measuring Mechanical Wave Velocity with Advanced Techniques

In addition to the basic formula and measurements, there are more advanced techniques and instruments available for determining the velocity of mechanical waves. These methods offer increased accuracy and precision, making them valuable for specialized applications.

Laser Doppler Velocimetry

Laser Doppler Velocimetry (LDV) is a non-invasive technique that uses the Doppler effect to measure the velocity of a mechanical wave. The LDV system can measure the velocity of a wave with an accuracy of ±0.1% and a resolution of 0.01 m/s.

Fiber Optic Sensors

Fiber optic sensors are highly sensitive instruments that can measure the velocity of a mechanical wave with an accuracy of ±0.01% and a resolution of 0.001 m/s. These sensors work by detecting the changes in the optical properties of the fiber caused by the wave’s propagation.

Ultrasonic Wave Sensors

Ultrasonic wave sensors use high-frequency sound waves to measure the velocity of a mechanical wave. These sensors can measure the velocity with an accuracy of ±0.5% and a resolution of 0.05 m/s, making them suitable for a wide range of applications.

Practical Examples and Numerical Problems

To further solidify your understanding of determining the velocity of mechanical waves, let’s explore some practical examples and numerical problems.

Example 1: Calculating Velocity from Frequency and Wavelength

Given:
– Frequency (f) = 5 Hz
– Wavelength (λ) = 2 meters

Calculate the velocity (v) of the wave.

Solution:

v = fλ
  = 5 Hz × 2 m
  = 10 m/s

Therefore, the velocity of the wave is 10 m/s.

Example 2: Calculating Frequency from Velocity and Wavelength

Given:
– Velocity (v) = 10 m/s
– Wavelength (λ) = 0.5 meters

Calculate the frequency (f) of the wave.

Solution:

v = fλ
f = v/λ
  = 10 m/s / 0.5 m
  = 20 Hz

Therefore, the frequency of the wave is 20 Hz.

Example 3: Calculating Wavelength from Frequency and Velocity

Given:
– Frequency (f) = 3 Hz
– Velocity (v) = 6 m/s

Calculate the wavelength (λ) of the wave.

Solution:

v = fλ
λ = v/f
  = 6 m/s / 3 Hz
  = 2 m

Therefore, the wavelength of the wave is 2 meters.

Factors Affecting Mechanical Wave Velocity

The velocity of a mechanical wave is not only determined by the frequency and wavelength but also influenced by various properties of the medium through which the wave propagates. Understanding these factors can provide valuable insights into the behavior of mechanical waves.

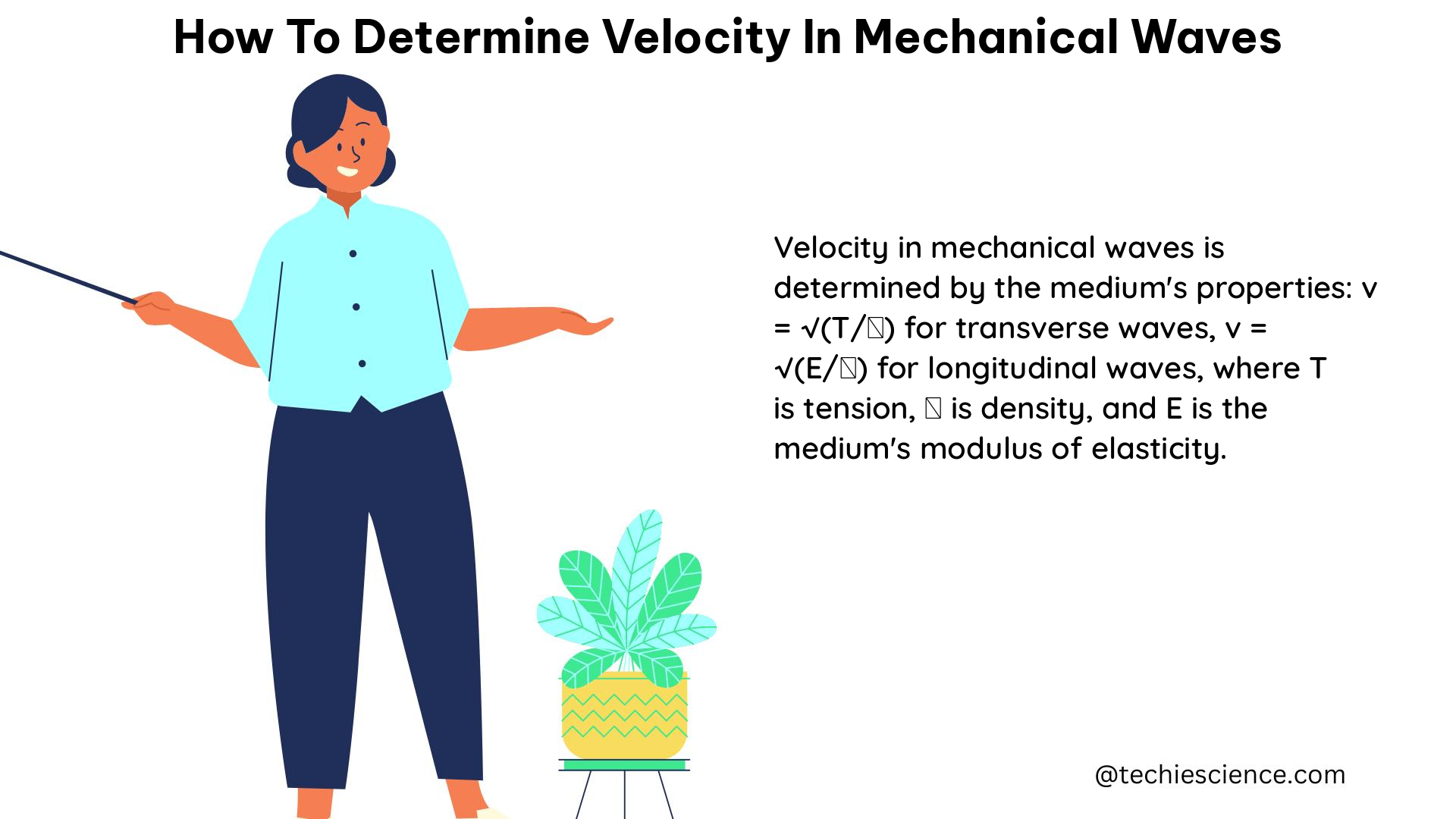
Density of the Medium (ρ)

The density of the medium affects the velocity of a mechanical wave. In general, the velocity of a wave is inversely proportional to the square root of the medium’s density.

Elasticity of the Medium (E)

The elasticity of the medium, often represented by the Young’s modulus or the bulk modulus, also plays a crucial role in determining the velocity of a mechanical wave. The velocity is proportional to the square root of the medium’s elasticity.

Temperature of the Medium (T)

The temperature of the medium can influence the velocity of a mechanical wave. As the temperature changes, the density and elasticity of the medium may also vary, leading to a corresponding change in the wave’s velocity.

By considering these factors, you can gain a deeper understanding of the complex behavior of mechanical waves and how to accurately determine their velocity in various scenarios.

Conclusion

Mastering the art of determining the velocity of mechanical waves is a valuable skill in the field of physics and engineering. By understanding the fundamental formula, measuring the frequency and wavelength, and utilizing advanced techniques, you can accurately calculate the velocity of mechanical waves in a wide range of applications.

Remember, the key to success in this domain lies in your ability to apply the concepts, perform calculations, and interpret the results with precision. Continuous practice and a deep understanding of the underlying principles will empower you to become a true expert in the realm of mechanical wave velocity.

Reference:

1. How to Calculate the Velocity of a Mechanical Wave – Study.com
2. Measuring mechanical wave speed, dispersion, and viscoelastic properties using optical coherence tomography
3. Mechanical Waves – BC Open Textbooks