How to Calculate the Energy of Seismic Waves: A Comprehensive Guide

Seismic waves, also known as earthquake waves, are vibrations that travel through the Earth’s crust as a result of an earthquake or other geological phenomena. These waves carry energy, which can be calculated to understand the magnitude and impact of an earthquake. In this blog post, we will explore how to calculate the energy of seismic waves, step by step, using formulas and examples. Let’s dive in!

How to Calculate the Energy of Seismic Waves

Calculating the Frequency of Seismic Waves

The frequency of a seismic wave refers to the number of cycles or vibrations it completes in a given unit of time. It is an essential parameter in the calculation of wave energy. The formula to calculate the frequency of a seismic wave is:

$\text{Frequency} = \frac{\text{Number of cycles}}{\text{Time}}$

For example, if a seismic wave completes 10 cycles in 2 seconds, the frequency would be:

$\text{Frequency} = \frac{10}{2} = 5 \text{ cycles per second}$

Determining the Speed of Seismic Waves

The speed of a seismic wave is the rate at which it propagates through the Earth’s crust. It is a crucial factor in energy calculation. The formula to determine the speed of a seismic wave is:

$\text{Speed} = \text{Wavelength} \times \text{Frequency}$

where the wavelength is the distance between two consecutive crests or troughs of the wave.

Procedure to Calculate the Energy of Seismic Waves

To calculate the energy of seismic waves, we need to know the amplitude and frequency of the wave, as well as the density of the medium through which the wave is traveling. The formula to calculate the energy of a seismic wave is:

$\text{Energy} = \frac{1}{2} \times \text{Density} \times \text{Amplitude}^2 \times \text{Velocity}$

where the density is the mass per unit volume of the medium, the amplitude is the maximum displacement of the wave from its equilibrium position, and the velocity is the speed at which the wave is propagating.

Worked out Examples on Seismic Waves Energy Calculation

Let’s work through a couple of examples to understand how to calculate the energy of seismic waves.

Example 1:
Given the following parameters:
– Frequency: 10 Hz
– Amplitude: 2 meters
– Density: 2000 kg/m^3
– Velocity: 500 m/s

Using the formula, we can calculate the energy of the seismic wave as follows:

$\text{Energy} = \frac{1}{2} \times 2000 \text{ kg/m}^3 \times (2 \text{ m})^2 \times 500 \text{ m/s}$

$\text{Energy} = 1000 \times 4 \times 500$

$\text{Energy} = 2,000,000 \text{ Joules}$

Example 2:
Given the following parameters:
– Frequency: 5 Hz
– Amplitude: 3 meters
– Density: 2500 kg/m^3
– Velocity: 600 m/s

Using the formula, we can calculate the energy of the seismic wave as follows:

$\text{Energy} = \frac{1}{2} \times 2500 \text{ kg/m}^3 \times (3 \text{ m})^2 \times 600 \text{ m/s}$

$\text{Energy} = 1250 \times 9 \times 600$

$\text{Energy} = 6,750,000 \text{ Joules}$

Application of Seismic Waves Energy Calculation in Geography

Role of Seismic Waves Energy in Earthquake Studies

The calculation of seismic wave energy plays a vital role in earthquake studies. It helps scientists and researchers understand the magnitude and destructive potential of earthquakes. By analyzing the energy released during an earthquake, they can assess the impact on structures, infrastructure, and human life, and devise strategies for mitigating the damage caused by future earthquakes.

Seismic Waves Energy and Earth’s Core Structure

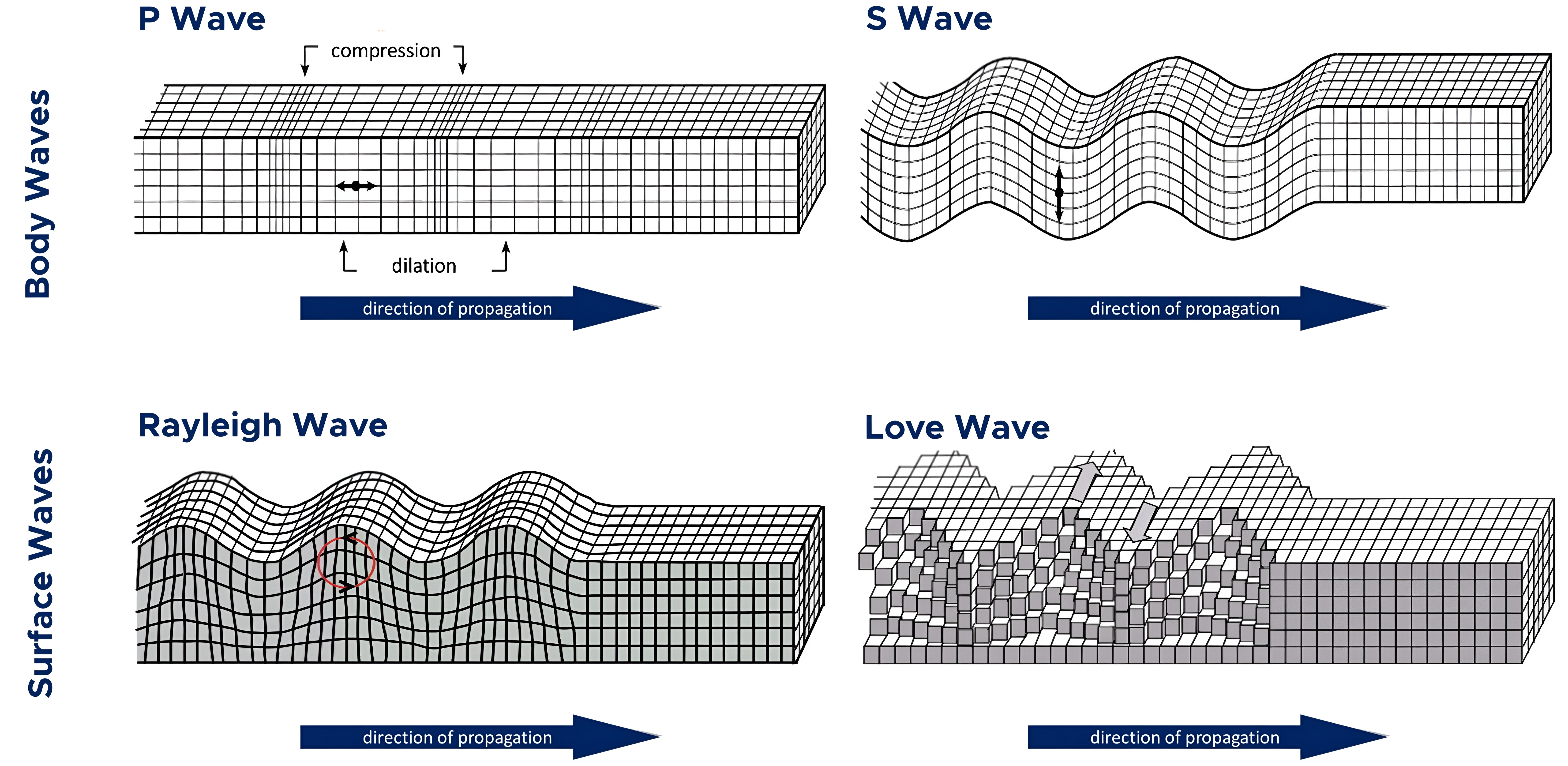
The study of seismic wave energy also provides valuable insights into the structure of the Earth’s core. Different types of seismic waves, such as P-waves and S-waves, travel at different speeds and have varying energy levels. By analyzing the energy distribution and propagation patterns of these waves, scientists can infer the composition and characteristics of the Earth’s core.

Practical Examples of Seismic Waves Energy Application in Geography

Seismic wave energy calculation finds practical applications in various fields of geography. It helps geologists locate and understand the distribution of natural resources such as oil, gas, and minerals. By analyzing the energy spectra of seismic waves, they can identify underground structures and geological formations that may contain valuable resources.

Additionally, seismic wave energy calculation is used in the field of seismology to monitor and predict volcanic eruptions. By measuring the energy released by volcanic tremors and analyzing the corresponding seismic waves, scientists can assess the volcanic activity and issue timely warnings to mitigate the potential risks to nearby communities.

Numerical Problems on How to Calculate the Energy of Seismic Waves

Problem 1

The amplitude of a seismic wave is given by the equation:

$A = \frac{E}{d}$

where $A$ is the amplitude, $E$ is the energy, and $d$ is the distance from the earthquake source.

If the amplitude of a seismic wave is 0.5 meters and the distance from the earthquake source is 1000 meters, calculate the energy of the seismic wave.

Solution:

Given:
Amplitude $\(A$ ) = 0.5 meters,
Distance $\(d$ ) = 1000 meters.

We can rearrange the equation to solve for the energy $\(E$ ):

$E = A \times d$

Substituting the given values into the equation, we have:

$E = 0.5 \times 1000 = 500$

Therefore, the energy of the seismic wave is 500 Joules.

Problem 2

The energy of a seismic wave is given by the equation:

$E = \frac{1}{2} \times k \times A^2$

where $E$ is the energy, $k$ is the spring constant, and $A$ is the amplitude.

If the spring constant is 100 N/m and the amplitude is 0.2 meters, calculate the energy of the seismic wave.

Solution:

Given:
Spring constant $\(k$ ) = 100 N/m,
Amplitude $\(A$ ) = 0.2 meters.

We can substitute the given values into the equation to calculate the energy $\(E$ ):

$E = \frac{1}{2} \times 100 \times (0.2)^2$

Simplifying the equation, we have:

$E = \frac{1}{2} \times 100 \times 0.04 = 2$

Therefore, the energy of the seismic wave is 2 Joules.

Problem 3

The energy of a seismic wave is given by the equation:

$E = \frac{4}{5} \times \pi \times r^2 \times d \times V$

where $E$ is the energy, $r$ is the radius of the seismic source, $d$ is the density of the medium, and $V$ is the velocity of the wave.

If the radius of the seismic source is 10 meters, the density of the medium is 2000 kg/m $^3$ , and the velocity of the wave is 500 m/s, calculate the energy of the seismic wave.

Solution:

Given:
Radius $\(r$ ) = 10 meters,
Density $\(d$ ) = 2000 kg/m $^3$ ,
Velocity $\(V$ ) = 500 m/s.

We can substitute the given values into the equation to calculate the energy $\(E$ ):

$E = \frac{4}{5} \times \pi \times (10)^2 \times 2000 \times 500$

Simplifying the equation, we have:

$E = \frac{4}{5} \times \pi \times 100 \times 2000 \times 500 = 502654824\pi$

Therefore, the energy of the seismic wave is $502654824\pi$ Joules.

Also Read:

Core SME lambdageeks.com

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.

All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.

lambdageeks.com