How to Calculate the Speed of Sound: A Comprehensive Guide

The speed of sound is a fundamental concept in physics, and understanding how to calculate it is crucial for various applications, from acoustics to meteorology. In this comprehensive guide, we will delve into the theoretical and practical aspects of calculating the speed of sound, providing you with a thorough understanding of this important topic.

Understanding the Basics of Sound Waves

Sound is a form of energy that travels through a medium, such as air, water, or solid materials, in the form of longitudinal waves. These waves are characterized by their wavelength (λ), frequency (f), and amplitude. The speed of sound (v) is the rate at which these waves propagate through the medium, and it is determined by the properties of the medium itself.

Calculating the Speed of Sound Using the Formula

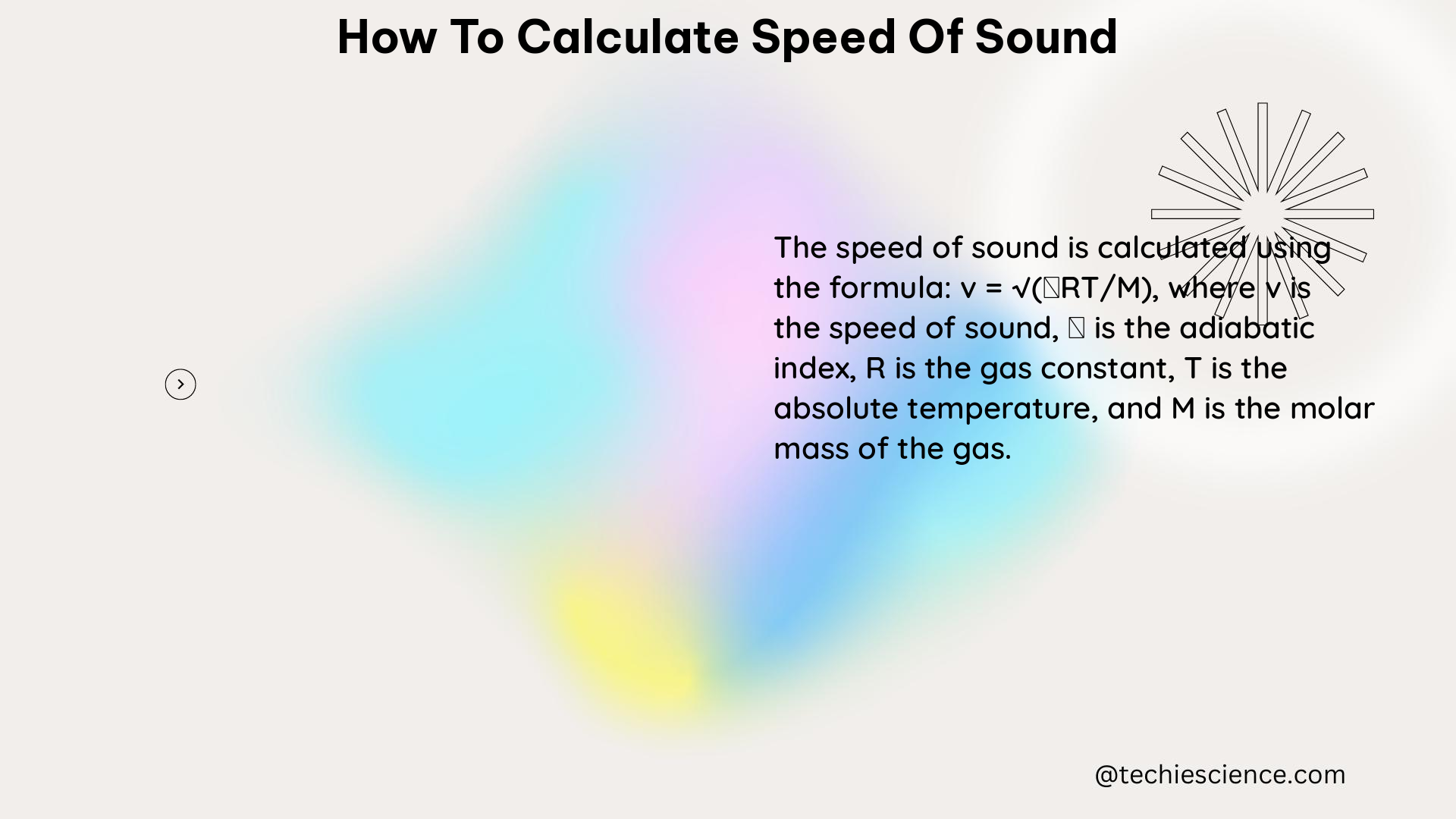
The speed of sound can be calculated using the formula:

v = λ × f

Where:
– v is the speed of sound (in meters per second, m/s)
– λ is the wavelength of the sound wave (in meters, m)
– f is the frequency of the sound wave (in Hertz, Hz)

This formula is based on the wave equation, which describes the relationship between the speed, wavelength, and frequency of a wave.

Example Calculation

Let’s say we have a sound wave with a frequency of 2,000 Hz and a wavelength of 0.35 meters. We can use the formula to calculate the speed of sound:

v = λ × f
v = 0.35 m × 2,000 Hz
v = 700 m/s

So, the speed of sound for this sound wave is 700 meters per second.

Calculating the Speed of Sound Using the Period

Alternatively, you can calculate the speed of sound using the period (T) of the sound wave, which is the reciprocal of the frequency:

v = λ / T

Where:
– v is the speed of sound (in meters per second, m/s)
– λ is the wavelength of the sound wave (in meters, m)
– T is the period of the sound wave (in seconds, s)

Example Calculation

Let’s say we have a sound wave with a wavelength of 0.35 meters and a period of 0.0005 seconds. We can use the formula to calculate the speed of sound:

v = λ / T
v = 0.35 m / 0.0005 s
v = 700 m/s

Again, the speed of sound for this sound wave is 700 meters per second.

Factors Affecting the Speed of Sound

The speed of sound can vary depending on the medium it is traveling through and the environmental conditions. Some of the key factors that affect the speed of sound include:

1. Temperature: The speed of sound increases as the temperature of the medium increases. In dry, cool air at 0°C, the speed of sound is approximately 331 m/s, while in dry, warm air at 20°C, the speed of sound is around 343 m/s.

2. Humidity: The presence of water vapor in the air can slightly increase the speed of sound. In humid air, the speed of sound is typically a few meters per second faster than in dry air at the same temperature.

3. Pressure: The speed of sound is also affected by the pressure of the medium. In general, the speed of sound increases as the pressure increases.

4. Composition of the Medium: The speed of sound can vary depending on the composition of the medium. For example, the speed of sound is faster in water (approximately 1,500 m/s) than in air (approximately 343 m/s at 20°C).

Measuring the Speed of Sound Experimentally

In addition to calculating the speed of sound using the formulas, you can also measure it experimentally. One common method is the “balloon pop” experiment, which involves the following steps:

1. Set up the experiment: Measure a known distance, such as 100 meters, and mark the starting and ending points.
2. Pop the balloon: Have someone pop a balloon at the starting point and start a stopwatch.
3. Measure the time: Stop the stopwatch when you hear the sound of the balloon popping at the ending point.
4. Calculate the speed: Subtract your reaction time from the total time measured, and then use the formula v = d/t to calculate the speed of sound, where v is the speed of sound, d is the distance, and t is the time.

For example, if the distance is 100 meters, the measured time is 0.50 seconds, and your reaction time is 0.23 seconds, the calculation would be:

Actual time = 0.50 s - 0.23 s = 0.27 s
Speed of sound = 100 m / 0.27 s = 370 m/s

You can repeat this experiment several times and take the average to get a more accurate measurement of the speed of sound.

Additional Considerations and Applications

The speed of sound is an important concept in various fields, including:

• Acoustics: Understanding the speed of sound is crucial for the design of musical instruments, concert halls, and other acoustic environments.
• Meteorology: The speed of sound is used to measure wind speed and the distance of lightning strikes.
• Ultrasound Imaging: The speed of sound is a key parameter in the calculation of the depth and location of structures in the body during ultrasound imaging.
• Shock Waves: The speed of sound is a critical factor in the study of shock waves, which are produced by objects moving at supersonic speeds.

Furthermore, the speed of sound can be affected by factors such as the composition of the medium, the presence of obstacles, and the direction of the wind. Understanding these factors is important for accurate calculations and measurements.

Conclusion

Calculating the speed of sound is a fundamental skill in physics, and this comprehensive guide has provided you with the necessary tools and knowledge to do so. By understanding the formulas, the factors that affect the speed of sound, and the experimental methods for measuring it, you can now confidently apply this knowledge to a wide range of applications and scenarios.

Reference Links:

1. The Physics Classroom – The Speed of Sound
2. Study.com – What is Sound? Definition, Wave Parameters, Pitch, Volume
3. LIGO Caltech – Calculating the Speed of Sound
4. NASA – The Speed of Sound
5. HyperPhysics – Speed of Sound