The sun is the primary source of energy for our planet, and understanding the radiant energy it emits is crucial for various scientific and practical applications. This comprehensive guide will walk you through the step-by-step process of calculating the radiant energy from the sun, covering key concepts, formulas, and examples.
Understanding the Solar Constant
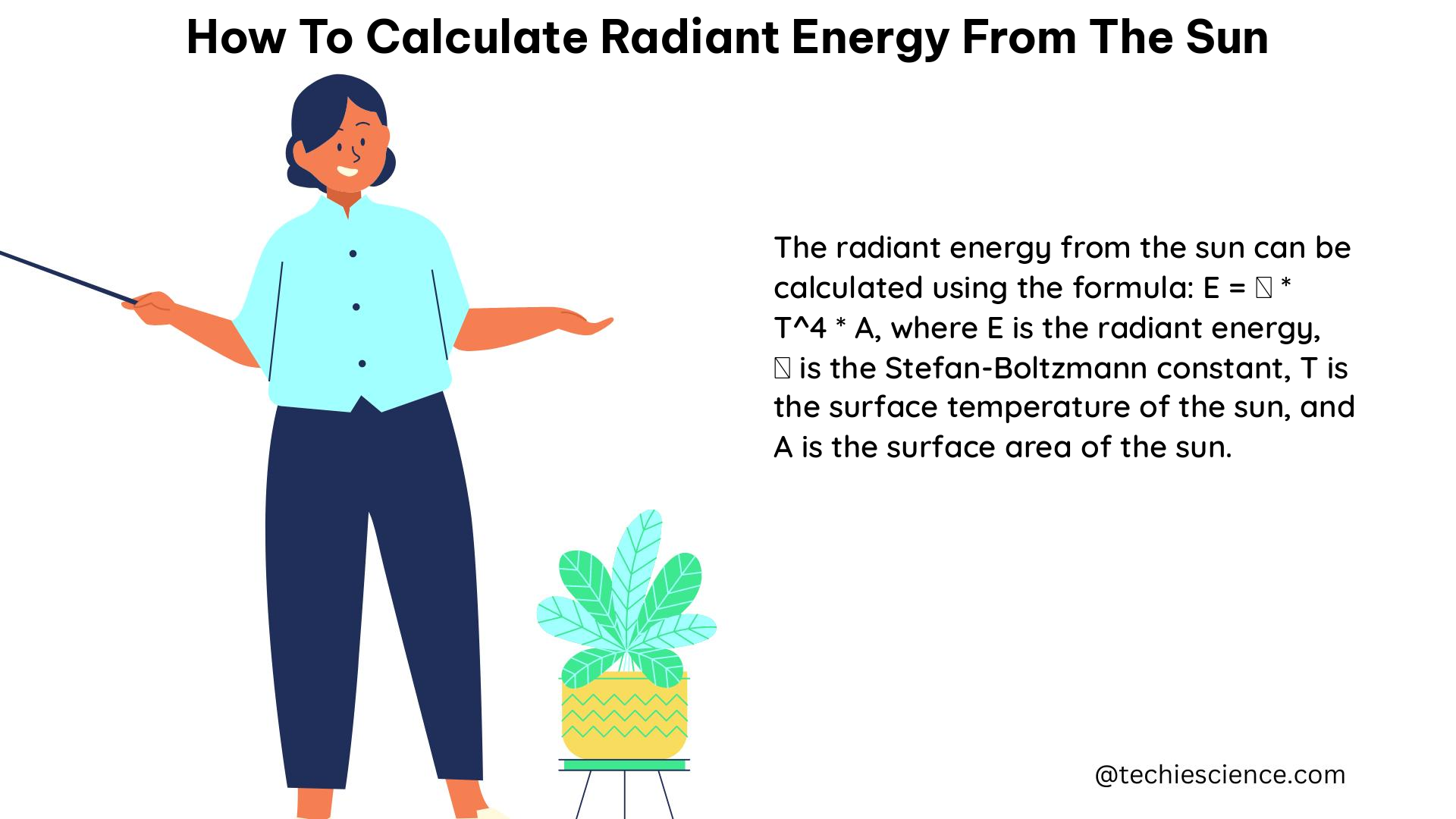
The solar constant is the amount of power per unit area received from the Sun in a straight line outside the Earth’s atmosphere. It is approximately equal to 1.4 kW/m².
The solar constant can be calculated using the following formula:
Solar Constant = E = σT^4
Where:
– E is the total energy emitted per unit surface area of the Sun
– σ is the Stefan-Boltzmann constant, which is equal to 5.67 × 10^-8 W/m² K^4
– T is the surface temperature of the Sun in Kelvin
Determining the Sun’s Surface Temperature
To calculate the radiant energy from the sun, we first need to determine the Sun’s surface temperature. We can do this by measuring the wavelength of the peak energy output and using Wien’s Displacement Law.
Wien’s Displacement Law states that the wavelength at which the radiant energy from a blackbody radiator is at its maximum is inversely proportional to the temperature of the radiator. The formula for the wavelength of maximum emission (λ_max) is:
λ_max = b/T
Where:
– λ_max is the wavelength of maximum emission in meters
– b is Wien’s displacement constant, which is equal to 2.898 × 10^-3 m K
– T is the temperature of the radiator in Kelvin
For example, if the peak wavelength of the Sun’s radiant energy is measured to be 500.0 nm, we can calculate the Sun’s surface temperature as follows:
T = b/λ_max
T = 2.898 × 10^-3 m K / 500.0 × 10^-9 m
T = 5796 K
Calculating the Total Radiant Energy Emitted by the Sun
Once we know the Sun’s surface temperature, we can calculate the total radiant energy emitted by the Sun using the Stefan-Boltzmann Law. The formula is:
E = σT^4
Where:
– E is the total energy emitted per unit surface area of the Sun
– σ is the Stefan-Boltzmann constant, which is equal to 5.67 × 10^-8 W/m² K^4
– T is the surface temperature of the Sun in Kelvin
Substituting the values, we get:
E = 5.67 × 10^-8 W/m² K^4 × (5796 K)^4
E = 6.33 × 10^7 W/m²
This is the total radiant energy emitted by the Sun per unit surface area.
Calculating the Total Radiant Energy Received by the Earth
To calculate the total radiant energy received by the Earth, we need to take into account the distance between the Earth and the Sun and the cross-sectional area of the Earth. The formula is:
E_Earth = E × πr^2 / (4d^2)
Where:
– E_Earth is the total radiant energy received by the Earth
– E is the total energy emitted per unit surface area of the Sun
– r is the radius of the Earth
– d is the distance between the Earth and the Sun
Substituting the values, we get:
E_Earth = 6.33 × 10^7 W/m² × π(6.37 × 10^6 m)^2 / (4 × (1.496 × 10^11 m)^2)
E_Earth = 1.74 × 10^17 W
This is the total radiant energy received by the Earth from the Sun.
Calculating the Radiant Energy Received per Unit Area on the Earth’s Surface
To calculate the radiant energy received per unit area on the Earth’s surface, we need to take into account the cross-sectional area of the Earth and the distance between the Earth and the Sun. The formula is:
E_surface = E_Earth / πr^2
Where:
– E_surface is the radiant energy received per unit area on the Earth’s surface
– E_Earth is the total radiant energy received by the Earth
– r is the radius of the Earth
Substituting the values, we get:
E_surface = 1.74 × 10^17 W / π(6.37 × 10^6 m)^2
E_surface = 1366 W/m²
This is the solar constant, which is the amount of power per unit area received from the Sun in a straight line outside the Earth’s atmosphere.
Calculating the Rate of Mass Loss in the Sun
To calculate the rate at which the Sun’s mass is being lost due to the conversion of mass to energy, we can use the mass-energy equivalence principle, which states that energy (E) is equal to mass (m) times the speed of light (c) squared. The formula is:
E = mc^2
Substituting the values, we get:
E = (1.4 × 10^3 W/m²) × (1.39 × 10^3 m)² / (3 × 10^8 m/s)²
E = 4.2 × 10^9 kg/s
This is the rate at which the Sun’s mass is being lost due to the conversion of mass to energy.
Calculating the Remaining Lifetime of the Sun
To calculate the remaining lifetime of the Sun, we can divide the Sun’s current mass by the rate at which it’s losing this mass. The formula is:
t = m / (dm/dt)
Where:
– t is the remaining lifetime of the Sun
– m is the current mass of the Sun
– dm/dt is the rate at which the Sun’s mass is being lost
Substituting the values, we get:
t = 2 × 10^30 kg / (4.2 × 10^9 kg/s)
t = 4.76 × 10^20 s
This is the remaining lifetime of the Sun in seconds. To convert it to years, we can use the formula:
1 year = 31536000 s
t = (4.76 × 10^20 s) / (31536000 s/year)
t = 1.51 × 10^11 years
This is the remaining lifetime of the Sun in years.
References:
– Vaia, “The radiant energy from the sun reaches its maximum at a wavelength of about 500.0 nm. What is the approximate temperature of the sun’s surface?”, https://www.vaia.com/en-us/textbooks/physics/university-physics-3-edition/chapter-6/problem-60-the-radiant-energy-from-the-sun-reaches-its-maxim/
– IOP Spark, “Measuring the Sun”, https://spark.iop.org/measuring-sun
– Physics Stack Exchange, “Calculating the amount of heat energy radiated by sun”, https://physics.stackexchange.com/questions/57392/calculating-the-amount-of-heat-energy-radiated-by-sun

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.