How to Calculate Potential Energy Change in an Elevator Moving Up a Building

Summary

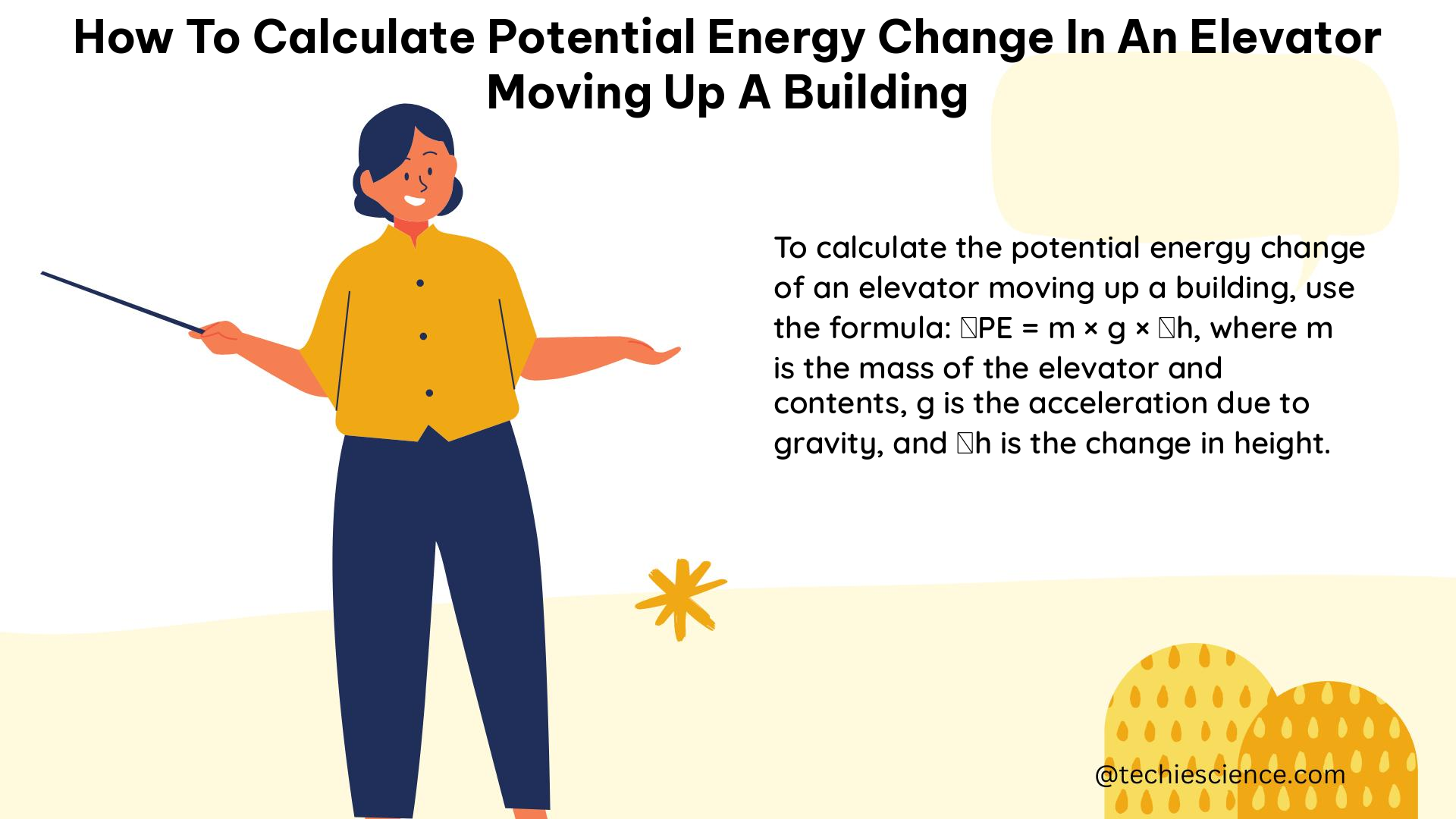
To calculate the potential energy change in an elevator moving up a building, you need to consider the mass of the elevator and its passengers, the acceleration due to gravity, and the height the elevator travels. The formula for potential energy is PE = mgh, where m is mass, g is the acceleration due to gravity, and h is height. This article provides a comprehensive guide on the step-by-step process, including relevant physics formulas, examples, and numerical problems, to help you master the calculation of potential energy change in an elevator.

Understanding Potential Energy

Potential energy is the energy an object possesses due to its position or state. In the case of an elevator moving up a building, the potential energy of the elevator and its passengers increases as they are lifted to a higher position.

The formula for calculating potential energy is:

PE = mgh

Where:
– PE is the potential energy (in Joules, J)
– m is the mass of the elevator and its passengers (in kilograms, kg)
– g is the acceleration due to gravity (9.8 m/s²)
– h is the height the elevator travels (in meters, m)

Calculating Potential Energy Change

To calculate the potential energy change in an elevator moving up a building, follow these steps:

1. Determine the Mass: Identify the total mass of the elevator and its passengers. This includes the mass of the elevator car, the counterweight, and the people inside.

2. Measure the Height: Determine the height the elevator travels, which is the difference between the starting and ending floor levels.

3. Apply the Potential Energy Formula: Plug the mass and height values into the potential energy formula:

PE = mgh

This will give you the change in potential energy as the elevator moves up the building.

Example Calculation

Let’s consider an example where an elevator with a total mass of 1000 kg (including passengers) ascends 50 meters in a building.

PE = mgh
PE = (1000 kg) * (9.8 m/s²) * (50 m)
PE = 490,000 Joules (J)

In this case, the elevator has gained 490,000 Joules of potential energy as it ascended 50 meters in the building.

Factors Affecting Potential Energy Change

There are a few additional factors to consider when calculating the potential energy change in an elevator:

1. Constant Speed: If the elevator is moving at a constant speed, there is no change in potential energy. The potential energy remains constant throughout the journey.

2. Motor Efficiency: The efficiency of the elevator’s motor can affect the calculation for the work required to lift the elevator and passengers. If the motor has an efficiency of 25%, the input work required would be 4 times the output work (potential energy).

3. Potential Energy Gain Rate: To calculate the rate at which the elevator plus the person is gaining potential energy, divide the potential energy by the time it takes to ascend. For example, if it takes 30 seconds to ascend 50 meters, the rate of potential energy gain would be:

Potential Energy Gain Rate = 490,000 J / 30 s = 16,333.33 Watts (W) or 16.33 kilowatts (kW)

This means that the elevator plus the person is gaining potential energy at a rate of 16.33 kW.

Numerical Problems

1. Problem: An elevator with a mass of 840 kg (including passengers) ascends from the ground floor to the 10th floor, a height of 41 meters, in 35 seconds. Calculate the power necessary to lift the elevator.

Solution:
– Mass (m) = 840 kg
– Height (h) = 41 m
– Time (t) = 35 s

Potential energy change:
PE = mgh
PE = (840 kg) * (9.8 m/s²) * (41 m)
PE = 338,280 J

Power required:
Power = PE / t
Power = 338,280 J / 35 s
Power = 9,665 W or 9.665 kW

Therefore, the power necessary to lift the elevator is 9.665 kW.

1. Problem: A person with a mass of 86.9 kg takes an elevator from the first floor to the 15th floor in a building, where the distance between floors is 4.47 meters. Calculate the change in potential energy for the person.

Solution:
– Mass (m) = 86.9 kg
– Height (h) = 14 floors * 4.47 m/floor = 62.58 m

Potential energy change:
PE = mgh
PE = (86.9 kg) * (9.8 m/s²) * (62.58 m)
PE = 53,400 J

Therefore, the change in potential energy for the person is 53,400 Joules.

Energy Consumption and Cost Considerations

When considering the overall energy consumption and costs of an elevator system, it’s important to take into account factors such as the efficiency of the motor, the counterweight system, and the energy-saving features of the elevator.

Tools like the TK Elevator Energy Calculator can help evaluate the energy consumption and costs of an elevator, and compare it against other elevator systems. These tools consider factors such as the elevator’s power consumption, energy efficiency, and operating costs, providing a comprehensive analysis to optimize the energy performance of the elevator.

Conclusion

Calculating the potential energy change in an elevator moving up a building is a fundamental concept in physics. By understanding the formula for potential energy (PE = mgh) and applying it to the specific parameters of the elevator and its journey, you can accurately determine the change in potential energy. This knowledge is crucial for understanding the energy requirements, efficiency, and overall performance of elevator systems. The examples and numerical problems provided in this article should give you a solid foundation to tackle potential energy calculations in elevator scenarios.

References

1. Work and energy in an elevator – Physics Stack Exchange. Retrieved from https://physics.stackexchange.com/questions/725938/work-and-energy-in-an-elevator
2. Calculate the change in potential energy of a 86.9 kg man when he takes an elevator from the first floor to 15th floor, if the distance between floors is 4.47 m. Retrieved from https://homework.study.com/explanation/calculate-the-change-in-potential-energy-of-a-86-9-kg-man-when-he-takes-an-elevator-from-the-first-floor-to-15th-floor-if-the-distance-between-floors-is-4-47-m-this-building-has-a-thirteenth-floor.html
3. A Physics 11 Elevator Work/Power Problem. Retrieved from https://www.physicsforums.com/threads/a-physics-11-elevator-work-power-problem.950992/
4. Problem 63 An elevator ascends from the ground floor to the 10th floor, a height of 41 m, in 35 s. Retrieved from https://www.vaia.com/en-us/textbooks/physics/essential-university-physics-global-edition-4-edition/chapter-6/problem-63-an-elevator-ascends-from-the-ground-floor-to-the-10th-floor-a-height-of-41-m-in-35-s-if-the-mass-of-the-elevator-and-passengers-is-840-kg-what-s-the-power-necessary-to-lift-the-elevator-your-answer-is-greater-than-the-actual-power-needed-because-elevators-are-counterweighted-thus-reducing-the-work-the-motor-needs-to-do
5. Energy Calculator | TK Elevator Escalators Moving Walks. Retrieved from https://design.na.tkelevator.com/tools/energy-calculator