How to Calculate Gravitational Potential Energy in an Orbit

by

Summary

Calculating the gravitational potential energy in an orbit involves using the formula PE = -G * (m1 * m2) / r, where PE is the potential energy, G is the gravitational constant, m1 and m2 are the masses of the objects, and r is the distance between their centers. This formula can be simplified when one object is much more massive than the other, as is the case with a satellite orbiting a planet. Accurate measurements of the masses and distances are crucial, and other forces like atmospheric drag must also be considered.

Understanding the Gravitational Potential Energy Formula

how to calculate gravitational potential energy in an orbit

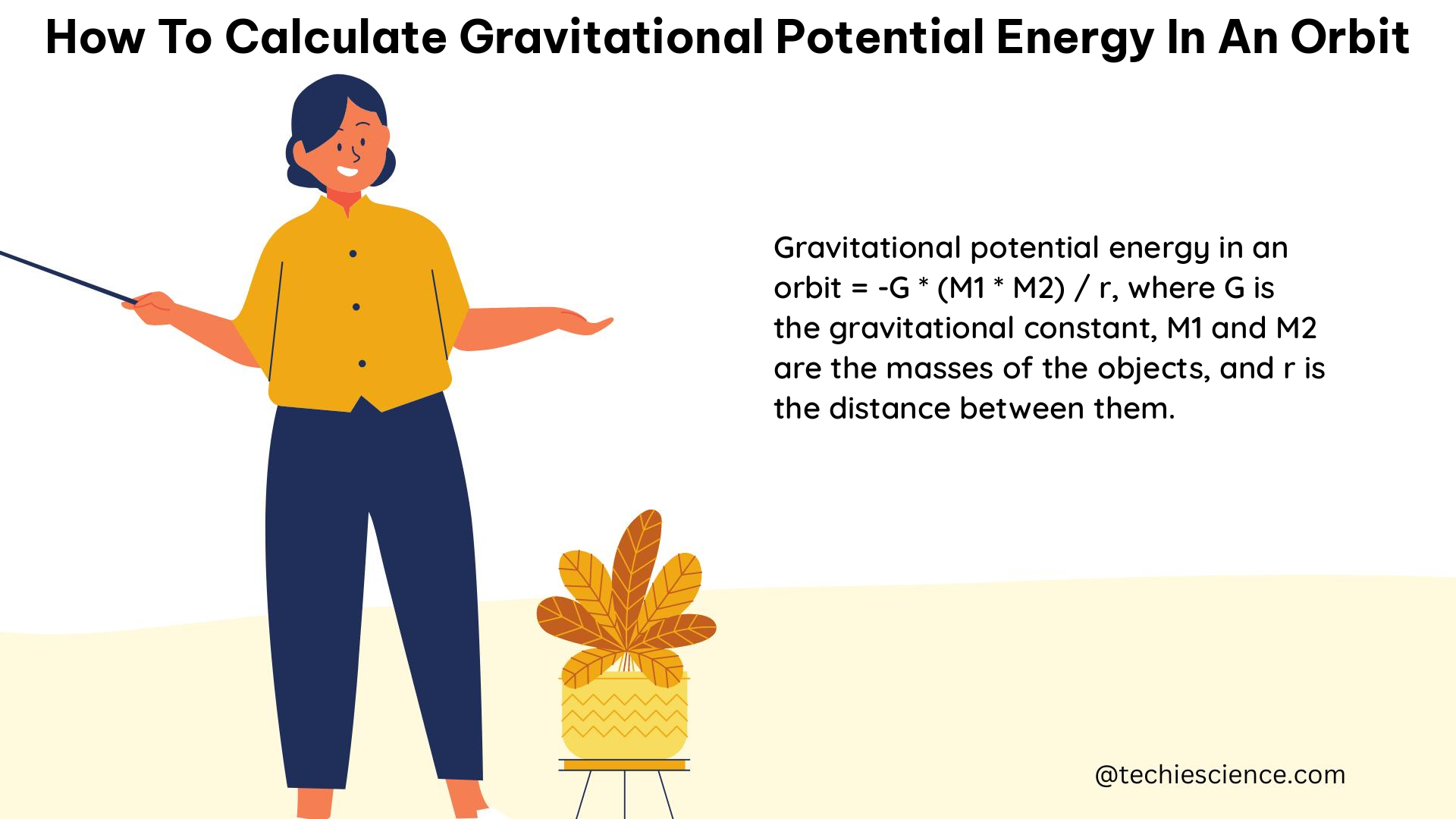
The formula for gravitational potential energy is:

PE = -G * (m1 * m2) / r

Where:
– PE is the gravitational potential energy (in Joules)
– G is the gravitational constant (6.674 × 10^-11 N⋅m^2/kg^2)
– m1 and m2 are the masses of the two objects (in kg)
– r is the distance between the centers of the two objects (in meters)

The negative sign in the formula indicates that the potential energy is lower than it would be if the objects were infinitely far apart. This is because the objects are being held together by the gravitational force.

Simplifying the Formula for Orbits

In the context of an orbit, we can simplify the formula by assuming that one of the objects is much more massive than the other. This is often the case when considering a satellite orbiting a planet, where the planet’s mass is significantly greater than the satellite’s mass.

In this scenario, we can treat the more massive object (the planet) as fixed and calculate the potential energy of the less massive object (the satellite) relative to the more massive one.

Calculating the Potential Energy of a Satellite in Orbit

Let’s consider an example of calculating the gravitational potential energy of a satellite in orbit around the Earth.

Given:
– Satellite mass (m1) = 370,000 kg
– Earth mass (m2) = 5.972 × 10^24 kg
– Satellite altitude above Earth’s surface = 350 km

First, we need to calculate the distance (r) between the satellite and the center of the Earth:

r = Earth's radius + Satellite altitude
r = 6.371 × 10^6 m + 350 × 10^3 m
r = 6.721 × 10^6 m

Now, we can plug the values into the gravitational potential energy formula:

PE = -G * (m1 * m2) / r
PE = -(6.674 × 10^-11 N⋅m^2/kg^2) * (370,000 kg * 5.972 × 10^24 kg) / (6.721 × 10^6 m)
PE = -1.02 × 10^11 J

Therefore, the gravitational potential energy of the satellite in this orbit is -1.02 × 10^11 Joules.

Importance of Accurate Measurements

Accurate measurements of the masses and distances involved are crucial for calculating the gravitational potential energy in an orbit. This can be achieved using various techniques, such as:

  1. Laser Ranging: Measuring the distance between the satellite and the Earth’s surface using laser pulses.
  2. Radio Tracking: Tracking the satellite’s position and velocity using radio signals.
  3. Satellite Laser Ranging (SLR): Measuring the distance between the satellite and ground-based laser stations.

It’s also important to consider the effects of other forces, such as atmospheric drag and solar radiation pressure, which can affect the satellite’s orbit and its potential energy.

Applications and Importance

Understanding how to calculate gravitational potential energy in an orbit is an essential part of learning about orbital mechanics and the behavior of objects in space. This knowledge is crucial in fields such as:

  1. Astrophysics: Studying the behavior of galaxies and other large-scale structures in the universe, where gravitational potential energy plays a significant role.
  2. Satellite and Spacecraft Design: Designing and optimizing the orbits of satellites and spacecraft to ensure their stability and efficiency.
  3. Space Exploration: Planning and executing missions to explore the solar system and beyond, where gravitational potential energy is a key factor.
  4. Satellite Communications: Ensuring the reliable operation of communication satellites in their orbits.

By mastering the techniques for calculating gravitational potential energy in an orbit, physics students can gain a deeper understanding of the fundamental principles of physics and their practical applications in the field of space science and engineering.

Conclusion

In summary, to calculate the gravitational potential energy in an orbit, you need to use the formula PE = -G * (m1 * m2) / r, where PE is the potential energy, G is the gravitational constant, m1 and m2 are the masses of the objects, and r is the distance between their centers. This formula can be simplified when one object is much more massive than the other, as is the case with a satellite orbiting a planet. Accurate measurements of the masses and distances are crucial, and other forces like atmospheric drag must also be considered. Understanding how to calculate gravitational potential energy in an orbit is an essential skill for physics students and professionals working in fields related to space science and engineering.

Reference:

  1. Estimate the Potential Energy of the International Space Station
  2. How to Calculate the Gravitational Potential Energy of an Object Above Earth
  3. Gravitational Potential Energy and Total Energy