How to Calculate Gravitational Energy in Satellite Orbits: A Comprehensive Guide

Calculating the gravitational energy in satellite orbits is a crucial aspect of understanding the dynamics and energy requirements of these celestial objects. This comprehensive guide will walk you through the step-by-step process of determining the gravitational potential energy and the changes in gravitational potential energy as a satellite moves between different orbits.

Understanding Gravitational Potential Energy

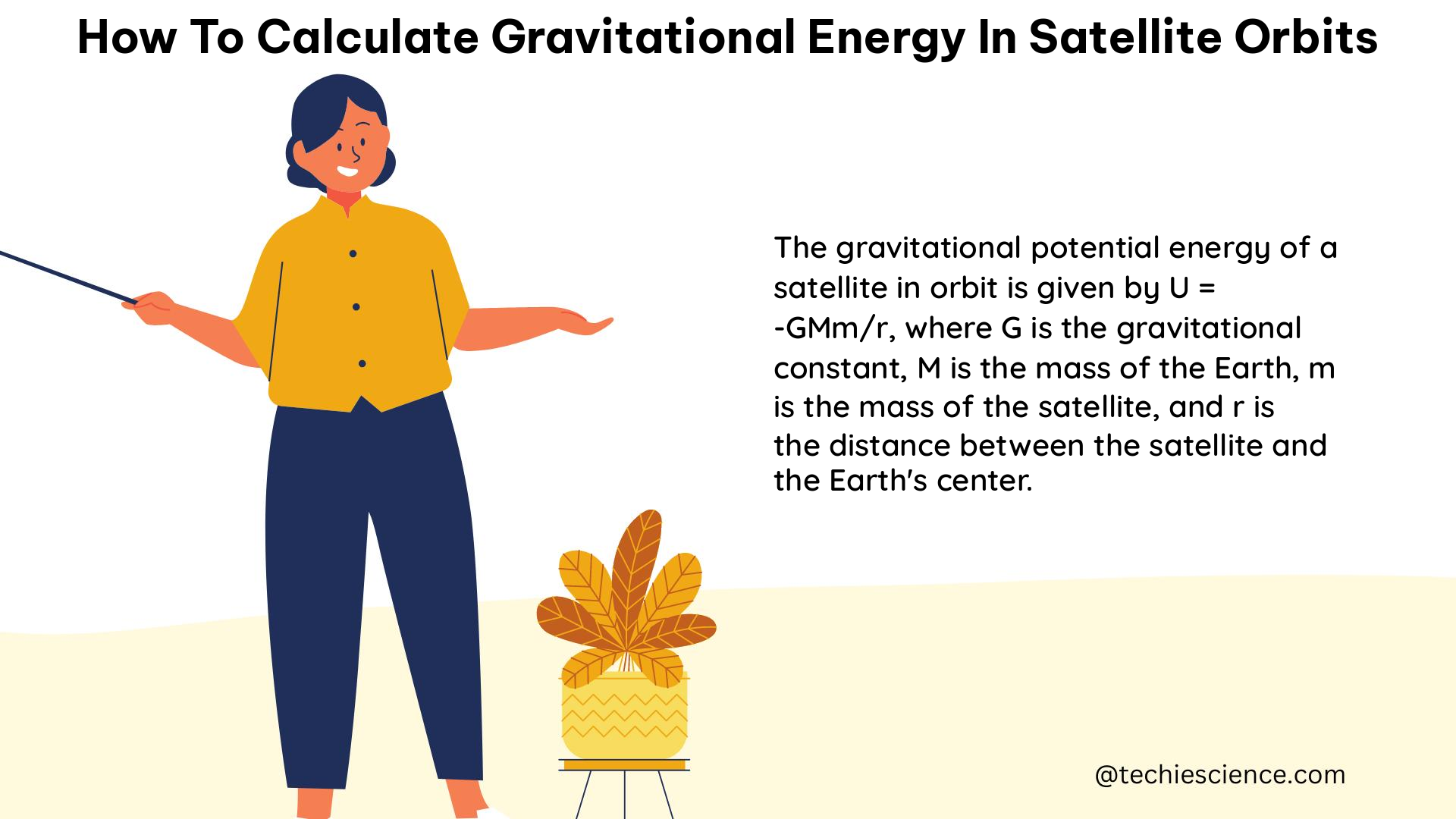
The gravitational potential energy (U) of a satellite in orbit around the Earth is given by the formula:

U = -G * (m_satellite * M_Earth) / (R_Earth + h)

Where:
– G is the gravitational constant (6.674 * 10^-11 N(m/kg)^2)
– m_satellite is the mass of the satellite
– M_Earth is the mass of the Earth (5.972 * 10^24 kg)
– R_Earth is the radius of the Earth (6.371 * 10^6 m)
– h is the height of the satellite above the Earth’s surface

The negative sign in the formula indicates that the gravitational potential energy is always negative, as it represents the energy required to move the satellite away from the Earth’s gravitational pull.

Example Calculation

Let’s consider a satellite with a mass of 1000 kg in a low Earth orbit at an altitude of 300 km. We can calculate the gravitational potential energy as follows:

U = -G * (m_satellite * M_Earth) / (R_Earth + h)
U = -(6.674 * 10^-11 N(m/kg)^2) * (1000 kg * 5.972 * 10^24 kg) / (6.371 * 10^6 m + 300 * 10^3 m)
U = -5.25 * 10^10 J

Therefore, the gravitational potential energy of the satellite in this orbit is -5.25 * 10^10 J.

Calculating Changes in Gravitational Potential Energy

As a satellite moves between different orbits, the change in gravitational potential energy (ΔU) can be calculated using the formula:

ΔU = -G * (m1 * m2) * (1/rf – 1/ri)

Where:
– ΔU is the change in gravitational potential energy
– m1 and m2 are the masses of the two objects (in this case, the satellite and the Earth)
– rf is the final distance between the two objects
– ri is the initial distance between the two objects

Example Calculation

Let’s say we want to calculate the change in gravitational potential energy as the satellite moves from a low Earth orbit at an altitude of 300 km to a higher orbit at an altitude of 10,000 km. We can calculate the initial and final distances as follows:

ri = R_Earth + h_initial = 6.371 * 10^6 m + 300 * 10^3 m = 6.671 * 10^6 m
rf = R_Earth + h_final = 6.371 * 10^6 m + 10,000 * 10^3 m = 7.371 * 10^6 m

We can then calculate the change in gravitational potential energy as follows:

ΔU = -G * (m_satellite * M_Earth) * (1/rf – 1/ri)
ΔU = -(6.674 * 10^-11 N(m/kg)^2) * (1000 kg * 5.972 * 10^24 kg) * (1/(7.371 * 10^6 m) – 1/(6.671 * 10^6 m))
ΔU = -3.25 * 10^11 J

Therefore, the change in gravitational potential energy as the satellite moves to the higher orbit is -3.25 * 10^11 J.

Factors Affecting Gravitational Potential Energy

Several factors can influence the gravitational potential energy of a satellite in orbit:

1. Satellite Mass: The mass of the satellite (m_satellite) directly affects the gravitational potential energy. Heavier satellites will have a higher gravitational potential energy.

2. Earth’s Mass: The mass of the Earth (M_Earth) is a constant in the gravitational potential energy formula. A more massive Earth will result in a higher gravitational potential energy for the satellite.

3. Orbital Altitude: The height of the satellite above the Earth’s surface (h) is a crucial factor. As the satellite’s altitude increases, the gravitational potential energy decreases.

4. Orbital Eccentricity: Satellites in elliptical orbits will have varying gravitational potential energy as they move between the apogee (farthest point from Earth) and perigee (closest point to Earth).

5. Gravitational Constant: The gravitational constant (G) is a fundamental physical constant that determines the strength of the gravitational force between two objects.

Advanced Considerations

1. Gravitational Potential Energy and Total Energy: The total energy of a satellite in orbit is the sum of its kinetic energy and gravitational potential energy. Understanding the relationship between these two forms of energy is crucial for analyzing the overall energy dynamics of a satellite.

2. Orbital Mechanics: The principles of orbital mechanics, such as Kepler’s laws of planetary motion and the conservation of angular momentum, are closely related to the calculation of gravitational potential energy and the changes in energy as a satellite moves between orbits.

3. Perturbations and Orbital Variations: In real-world scenarios, various factors, such as atmospheric drag, solar radiation pressure, and the gravitational influence of other celestial bodies, can cause perturbations and variations in a satellite’s orbit, which can affect the calculations of gravitational potential energy.

4. Numerical Simulations: Advanced computational tools and numerical simulations can be used to model the complex dynamics of satellite orbits, including the changes in gravitational potential energy, with greater accuracy and precision.

Conclusion

Calculating the gravitational energy in satellite orbits is a fundamental aspect of understanding the dynamics and energy requirements of these celestial objects. By mastering the formulas and concepts presented in this guide, you can accurately determine the gravitational potential energy of a satellite in a given orbit and the changes in energy as the satellite moves between different orbits. This knowledge is crucial for satellite design, mission planning, and the optimization of satellite operations.

References

1. The Physics Classroom – Mathematics of Satellite Motion
2. Lumen Learning – Gravitational Potential Energy and Total Energy
3. Study.com – How to Calculate the Gravitational Potential Energy of an Object Above Earth
4. Science Ready – Gravitational Potential Energy and Energy Changes in Space