Calculating the Energy Needed to Heat: A Comprehensive Guide

Summary

Calculating the energy required to heat a substance is a fundamental concept in physics and thermodynamics. This comprehensive guide will walk you through the step-by-step process of using the formula Q = mcΔT to determine the heat energy needed, where Q is the heat energy, m is the mass of the substance, c is the specific heat capacity, and ΔT is the change in temperature. We’ll also explore the importance of specific heat capacity, its variation across different substances, and how calorimetry can be used to measure the change in enthalpy during chemical reactions.

Understanding the Q = mcΔT Formula

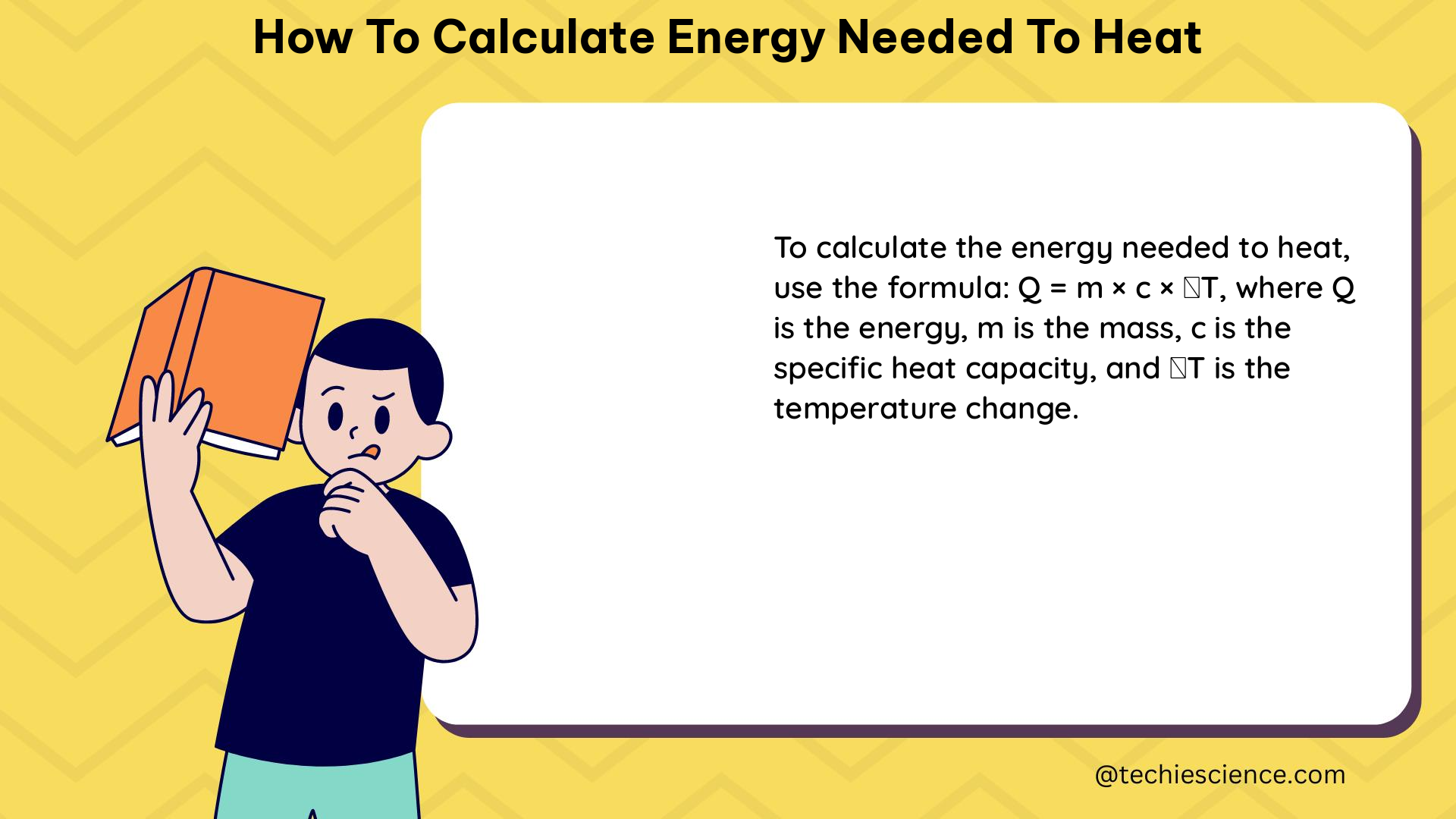
The formula Q = mcΔT is the cornerstone of calculating the energy needed to heat a substance. Let’s break down each component:

1. Q (Heat Energy): This represents the amount of heat energy required to raise the temperature of the substance. The unit for Q is Joules (J).

2. m (Mass): This is the mass of the substance being heated, typically measured in kilograms (kg).

3. c (Specific Heat Capacity): The specific heat capacity is the amount of heat energy required to raise the temperature of one unit of mass of a substance by one degree Celsius. The unit for c is Joules per kilogram-degree Celsius (J/kg°C).

4. ΔT (Change in Temperature): This is the difference between the final and initial temperatures of the substance, measured in degrees Celsius (°C).

To use this formula, you’ll need to know the mass of the substance, its specific heat capacity, and the desired change in temperature. Plugging these values into the equation will give you the amount of heat energy required to achieve the desired temperature change.

Calculating Specific Heat Capacity

The specific heat capacity (c) is a crucial parameter in the Q = mcΔT formula. The specific heat capacity can vary significantly depending on the substance. Here’s a table of the specific heat capacities for various substances:

Substance	Symbol (state)	Specific Heat (J/g°C)
Helium	He(g)	5.193
Water	H2O(l)	4.184
Ethanol	C2H6O(l)	2.376
Ice	H2O(s)	2.093 (at -10°C)
Water vapor	H2O(g)	1.864
Nitrogen	N2(g)	1.040
Air	–	1.007
Oxygen	O2(g)	0.918
Aluminum	Al(s)	0.897

It’s important to note that the specific heat capacity can also vary with temperature, pressure, and other factors. For accurate calculations, it’s best to use the specific heat capacity value that corresponds to the conditions of your system.

Example Calculation: Heating Water

Let’s consider an example of calculating the energy needed to heat 2 kg of water from 20°C to 80°C.

Given:
– Mass (m) = 2 kg
– Initial temperature (T1) = 20°C
– Final temperature (T2) = 80°C
– Specific heat capacity of water (c) = 4.184 J/g°C (or 4180 J/kg°C)

Step 1: Calculate the change in temperature (ΔT).
ΔT = T2 – T1 = 80°C – 20°C = 60°C

Step 2: Plug the values into the Q = mcΔT formula.
Q = m × c × ΔT
Q = 2 kg × 4180 J/kg°C × 60°C
Q = 500,400 J

Therefore, the energy needed to heat 2 kg of water from 20°C to 80°C is 500,400 J.

Calorimetry and Enthalpy Changes

In addition to calculating the energy needed to heat a substance, the Q = mcΔT formula can also be used to determine the change in enthalpy (ΔH) during a chemical reaction. This is done through the process of calorimetry, which involves measuring the heat flow and temperature changes in a system.

For example, if a chemical reaction releases 100 kJ of heat and the temperature of the calorimeter increases by 5°C, we can calculate the heat capacity of the calorimeter using the formula:

C = Q/ΔT

Where:
– C is the heat capacity of the calorimeter (in J/°C)
– Q is the heat energy released by the reaction (in J)
– ΔT is the change in temperature of the calorimeter (in °C)

Plugging in the values, we get:
C = 100 kJ / 5°C
C = 20 kJ/°C

This means the heat capacity of the calorimeter is 20 kJ/°C.

By measuring the temperature change in a calorimeter during a chemical reaction, you can use the Q = mcΔT formula to determine the change in enthalpy (ΔH) for the reaction. This is a powerful tool for understanding the energy changes that occur in chemical processes.

Advanced Considerations

When dealing with more complex systems or situations, there are a few additional factors to consider:

1. Phase Changes: When a substance undergoes a phase change (e.g., from liquid to gas), the specific heat capacity can change significantly. In these cases, you may need to use the latent heat of vaporization or fusion instead of the specific heat capacity.

2. Pressure and Volume Changes: If the system experiences changes in pressure or volume, you may need to account for work done by or on the system, which would affect the overall energy balance.

3. Non-Uniform Heating: If the substance is not heated uniformly, you may need to consider the spatial distribution of temperature and heat flow within the system.

4. Thermal Losses: In real-world scenarios, there may be thermal losses to the environment, which would need to be accounted for in the energy calculations.

5. Transient Effects: When dealing with time-dependent heating or cooling processes, you may need to consider the transient behavior of the system, which can involve differential equations and more advanced mathematical techniques.

These advanced considerations are beyond the scope of this introductory guide, but they highlight the complexity and nuance involved in accurately calculating the energy needed to heat a substance in more complex real-world situations.

Conclusion

Calculating the energy needed to heat a substance is a fundamental skill in physics and thermodynamics. By understanding the Q = mcΔT formula and the importance of specific heat capacity, you can determine the amount of heat energy required to raise the temperature of a substance by a desired amount. Additionally, the principles of calorimetry can be used to measure the change in enthalpy during chemical reactions.

Remember, this guide provides a solid foundation, but as you encounter more complex systems or situations, you may need to consider additional factors and apply more advanced techniques to ensure accurate energy calculations.

References

1. Energy & Heat – Foundations of Physics – BC Open Textbooks
2. Chemistry LibreTexts
3. AP Chem Study Guide 2024
4. The Physics Classroom