Calculating the energy stored in a solenoid is a crucial step in understanding the behavior and performance of electromagnetic devices. This comprehensive guide will walk you through the key formulas, principles, and examples to help you accurately determine the energy stored in a solenoid.
Understanding Solenoid Energy
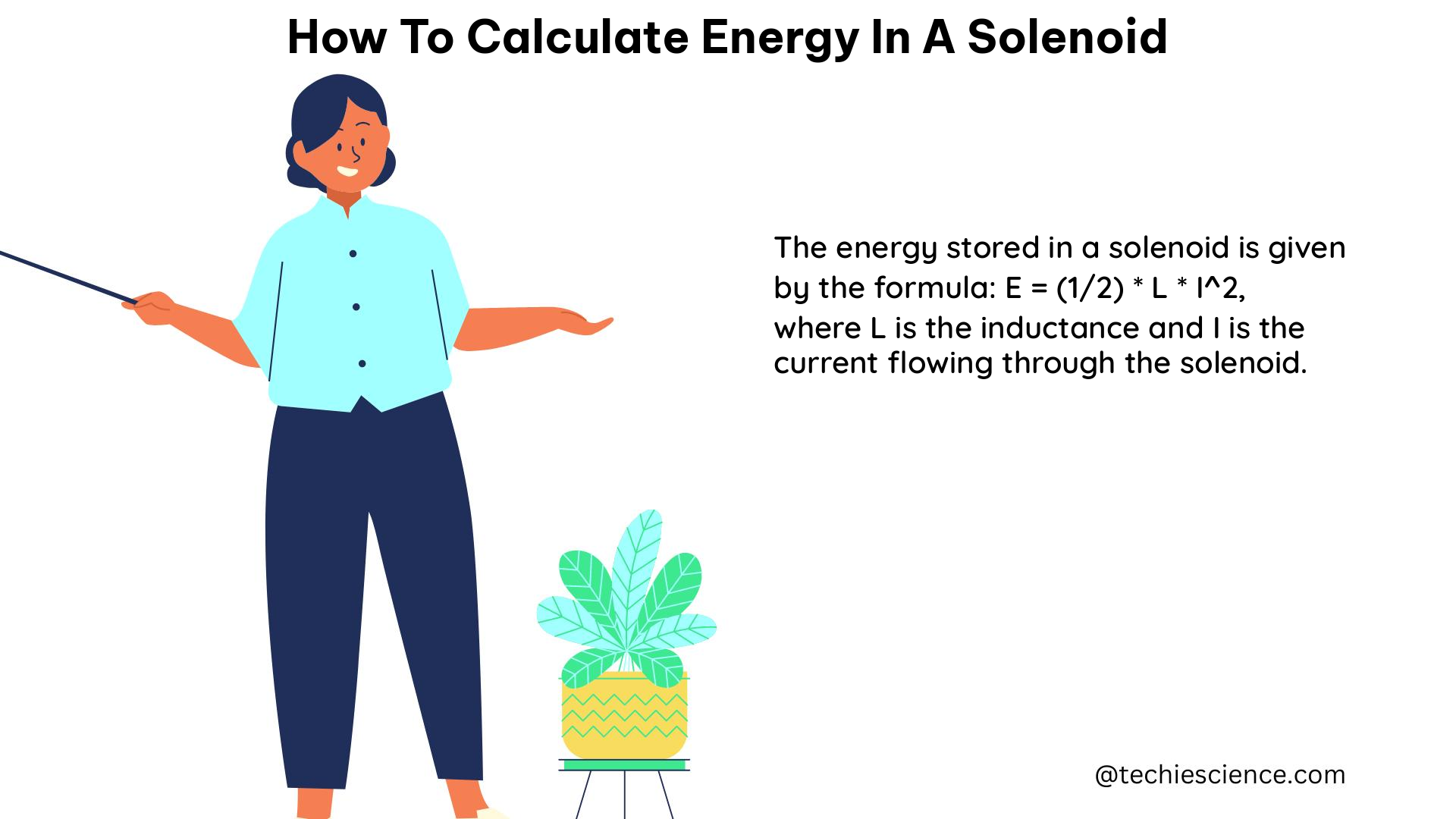
A solenoid is an electromagnetic coil that generates a magnetic field when an electric current flows through it. The energy stored in a solenoid is directly related to the magnetic field it produces and the current flowing through the coil. This energy can be calculated using the following formula:
E = 1/2 * L * I^2
Where:
– E is the energy stored in the solenoid (in Joules)
– L is the self-inductance of the solenoid (in Henries)
– I is the steady-state current flowing through the solenoid (in Amperes)
To calculate the energy stored in a solenoid, you need to determine the self-inductance (L) and the steady-state current (I) flowing through the coil.
Calculating Solenoid Self-Inductance
The self-inductance of a solenoid is a measure of the magnetic flux produced by the coil per unit of current flowing through it. It can be calculated using the following formula:
L = μ₀ * N^2 * A / L
Where:
– L is the self-inductance of the solenoid (in Henries)
– μ₀ is the permeability of free space (4π × 10^-7 T·m/A)
– N is the number of turns in the solenoid
– A is the cross-sectional area of the solenoid (in square meters)
– L is the length of the solenoid (in meters)
To use this formula, you’ll need to know the number of turns in the solenoid, the cross-sectional area, and the length of the solenoid.
Example Calculation
Let’s say you have a solenoid with the following characteristics:
– Number of turns (N) = 500
– Cross-sectional area (A) = 0.01 m^2
– Length (L) = 0.5 m
Using the formula above, we can calculate the self-inductance of the solenoid:
L = μ₀ * N^2 * A / L
L = (4π × 10^-7 T·m/A) * (500)^2 * (0.01 m^2) / (0.5 m)
L = 0.0628 H
So, the self-inductance of the solenoid is 0.0628 H.
Calculating Magnetic Flux
The magnetic flux (Φ) produced by the solenoid is another important factor in determining the energy stored. The magnetic flux can be calculated using the following formula:
Φ = B * A
Where:
– Φ is the magnetic flux (in Webers)
– B is the magnetic field strength (in Teslas)
– A is the cross-sectional area of the solenoid (in square meters)
To find the magnetic field strength (B), you can use the following formula:
B = μ₀ * N * I / L
Where:
– B is the magnetic field strength (in Teslas)
– μ₀ is the permeability of free space (4π × 10^-7 T·m/A)
– N is the number of turns in the solenoid
– I is the steady-state current flowing through the solenoid (in Amperes)
– L is the length of the solenoid (in meters)
Example Calculation
Let’s continue with the previous example, where we have a solenoid with:
– Number of turns (N) = 500
– Cross-sectional area (A) = 0.01 m^2
– Length (L) = 0.5 m
– Steady-state current (I) = 5 A
First, we can calculate the magnetic field strength (B):
B = μ₀ * N * I / L
B = (4π × 10^-7 T·m/A) * 500 * 5 A / 0.5 m
B = 0.628 T
Now, we can calculate the magnetic flux (Φ):
Φ = B * A
Φ = 0.628 T * 0.01 m^2
Φ = 0.00628 Wb
Calculating Energy Stored in the Solenoid
With the self-inductance (L) and the steady-state current (I) known, we can now calculate the energy stored in the solenoid using the formula:
E = 1/2 * L * I^2
Continuing the example:
– Self-inductance (L) = 0.0628 H
– Steady-state current (I) = 5 A
E = 1/2 * L * I^2
E = 1/2 * 0.0628 H * (5 A)^2
E = 3.9 J
Therefore, the energy stored in the solenoid is 3.9 Joules.
Additional Considerations
- The energy stored in a solenoid is directly proportional to the square of the current flowing through it. Doubling the current will result in four times the energy stored.
- The energy stored is also directly proportional to the self-inductance of the solenoid. Increasing the number of turns, the cross-sectional area, or decreasing the length of the solenoid will increase the self-inductance and, consequently, the energy stored.
- In practice, the energy stored in a solenoid may be affected by factors such as the material of the core, the presence of magnetic shielding, and the operating temperature, which can influence the permeability and resistance of the coil.
Conclusion
Calculating the energy stored in a solenoid is a crucial step in understanding the behavior and performance of electromagnetic devices. By using the formulas and principles outlined in this guide, you can accurately determine the energy stored in a solenoid based on its physical characteristics and the steady-state current flowing through it. This knowledge can be applied in various fields, such as electrical engineering, physics, and the design of electromagnetic systems.
References:
- Physics 47 Inductance (9 of 20) Energy Stored in a Solenoid
- Design and Application of Near Infrared LED and Solenoid Magnetic Field
- Energy in a Magnetic Field – Physics – StudySmarter
- An air-filled toroidal solenoid has a mean radius of 15.0 cm
- How Can I Calculate the Energy Requirement for a Lateral Solenoid?

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.