How to Calculate Binding Energy: A Comprehensive Guide

The binding energy of a nucleus is a crucial concept in nuclear physics, as it represents the energy required to separate a nucleus into its individual nucleons (protons and neutrons). Understanding how to calculate binding energy is essential for studying the stability and properties of atomic nuclei. In this comprehensive guide, we will delve into the step-by-step process of calculating binding energy, providing you with the necessary tools and knowledge to become proficient in this area.

Determining the Mass Defect

The first step in calculating the binding energy of a nucleus is to determine the mass defect. The mass defect is the difference between the mass of the nucleus and the sum of the masses of its constituent nucleons. This can be expressed mathematically as:

Mass Defect = (Sum of Masses of Constituent Nucleons) – (Mass of Nucleus)

To calculate the mass defect, we need to know the masses of the protons and neutrons that make up the nucleus. The mass of a proton is 1.00728 atomic mass units (amu), and the mass of a neutron is 1.00867 amu.

Let’s consider an example of calculating the mass defect for the copper-63 (^63Cu) nucleus. Copper-63 has 29 protons and 34 neutrons, so the combined mass of the nucleons is:

29 protons × 1.00728 amu/proton + 34 neutrons × 1.00867 amu/neutron = 63.541578 amu

The mass of the copper-63 nucleus is 62.929600 amu. Therefore, the mass defect is:

Mass Defect = 63.541578 amu – 62.929600 amu = 0.611978 amu

Converting Mass Defect to Binding Energy

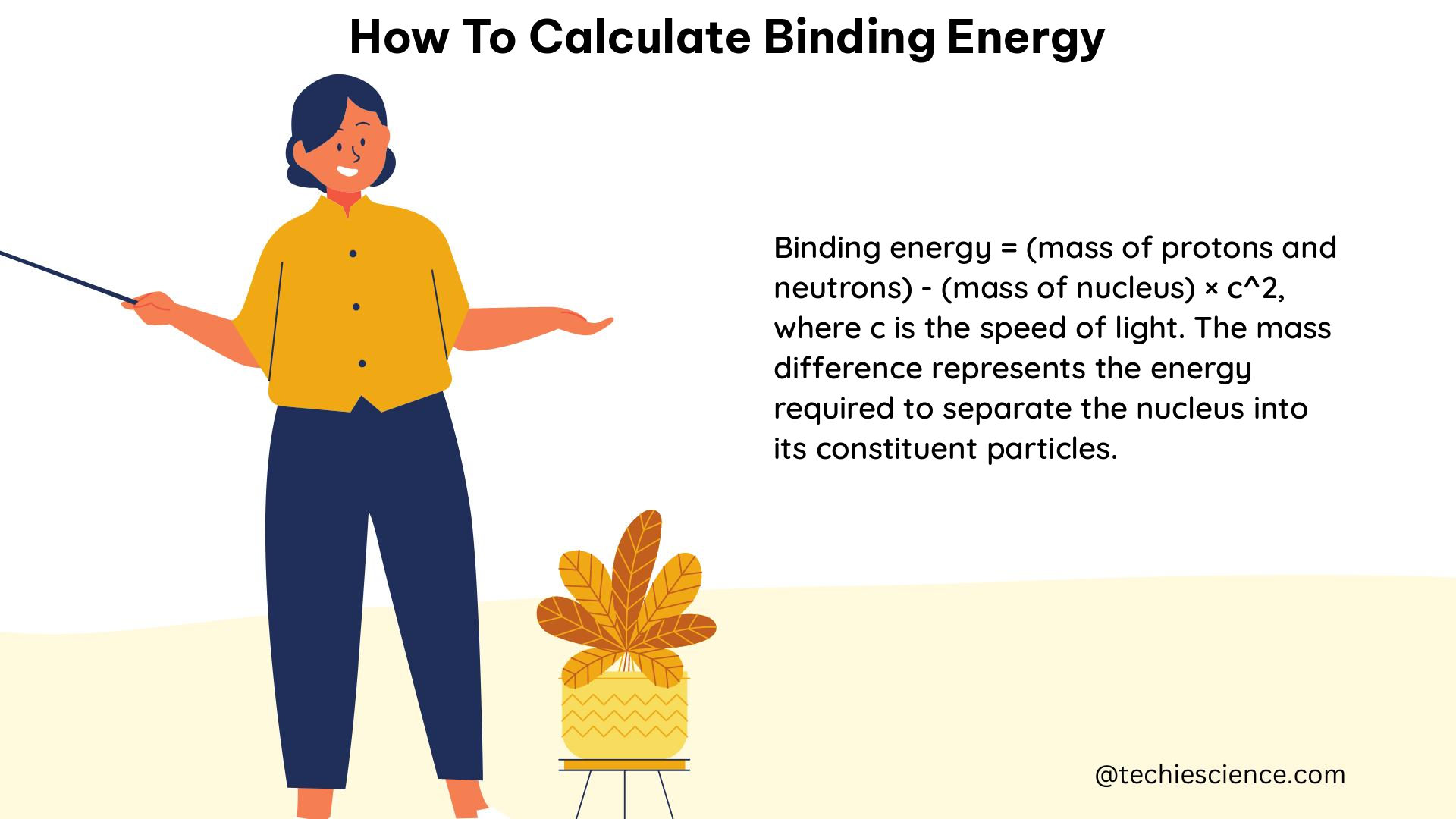
Once we have the mass defect, we can convert it into the binding energy of the nucleus using the famous Einstein equation, E = mc^2, where:

• E is the binding energy (in energy units)
• m is the mass defect (in mass units)
• c is the speed of light in a vacuum, approximately 3.00 × 10^8 m/s

Applying this equation to the copper-63 example, we get:

Binding Energy = 0.611978 amu × (931.5 MeV/c^2) × (c^2)
Binding Energy = 567.98 MeV

Calculating Binding Energy per Nucleon

The binding energy per nucleon is a useful quantity that allows us to compare the stability of different nuclei. It is calculated by dividing the total binding energy of the nucleus by the number of nucleons (protons and neutrons) in the nucleus. For the copper-63 example, the binding energy per nucleon is:

Binding Energy per Nucleon = 567.98 MeV / 63 = 9.016 MeV/nucleon

Graphing Binding Energy per Nucleon

A graph of binding energy per nucleon versus mass number (A) can be used to assess the relative stability of a nucleus. This graph typically shows a characteristic “binding energy curve” that reaches a maximum value for the iron-56 (^56Fe) nucleus, which has a mass number of 56 and 26 protons and 30 neutrons. This means that iron-56 is the most stable nucleus in terms of binding energy per nucleon.

The binding energy curve can be divided into two regions:

1. Light Nuclei: For nuclei with low mass numbers (A < 56), the binding energy per nucleon increases as the mass number increases. This is because the strong nuclear force becomes more effective as the number of nucleons increases, leading to greater stability.

2. Heavy Nuclei: For nuclei with high mass numbers (A > 56), the binding energy per nucleon decreases as the mass number increases. This is due to the increasing repulsive forces between the protons, which become more dominant as the nucleus grows larger.

The peak of the binding energy curve at iron-56 represents the most stable configuration, as it has the highest binding energy per nucleon. This information is crucial for understanding nuclear stability, fission, and fusion processes.

Numerical Examples

To further solidify your understanding, let’s work through a few numerical examples:

1. Calculating the Binding Energy of Helium-4 (^4He)
2. Helium-4 has 2 protons and 2 neutrons.
3. The combined mass of the nucleons is: 2 × 1.00728 amu + 2 × 1.00867 amu = 4.032 amu.
4. The mass of the helium-4 nucleus is 4.002602 amu.
5. The mass defect is: 4.032 amu – 4.002602 amu = 0.029398 amu.
6. The binding energy is: 0.029398 amu × (931.5 MeV/c^2) × (c^2) = 27.31 MeV.

7. The binding energy per nucleon is: 27.31 MeV / 4 = 6.828 MeV/nucleon.

8. Calculating the Binding Energy of Uranium-235 (^235U)

9. Uranium-235 has 92 protons and 143 neutrons.
10. The combined mass of the nucleons is: 92 × 1.00728 amu + 143 × 1.00867 amu = 235.043646 amu.
11. The mass of the uranium-235 nucleus is 235.043923 amu.
12. The mass defect is: 235.043646 amu – 235.043923 amu = -0.000277 amu.
13. The binding energy is: -0.000277 amu × (931.5 MeV/c^2) × (c^2) = -0.258 MeV.
14. The binding energy per nucleon is: -0.258 MeV / 235 = -0.0011 MeV/nucleon.

These examples demonstrate the step-by-step process of calculating the binding energy and binding energy per nucleon for different nuclei, highlighting the importance of considering the mass defect and using the appropriate formulas.

Conclusion

In this comprehensive guide, we have explored the fundamental concepts and step-by-step process of calculating the binding energy of a nucleus. By understanding the mass defect, converting it to binding energy, and analyzing the binding energy per nucleon, you now have the necessary tools to become proficient in this crucial aspect of nuclear physics. Remember to practice with various examples and continue exploring the fascinating world of nuclear binding energy.

Reference:

1. Binding Energy and the Mass Defect
2. Nuclear Binding Energy
3. How to Calculate Nuclear Binding Energy