How to Calculate Acceleration Using Force and Mass: A Comprehensive Guide

Calculating acceleration using force and mass is a fundamental concept in classical mechanics, governed by Newton’s second law of motion. This comprehensive guide will walk you through the step-by-step process, provide theoretical explanations, and offer practical examples to help you master this essential skill.

Understanding the Relationship Between Force, Mass, and Acceleration

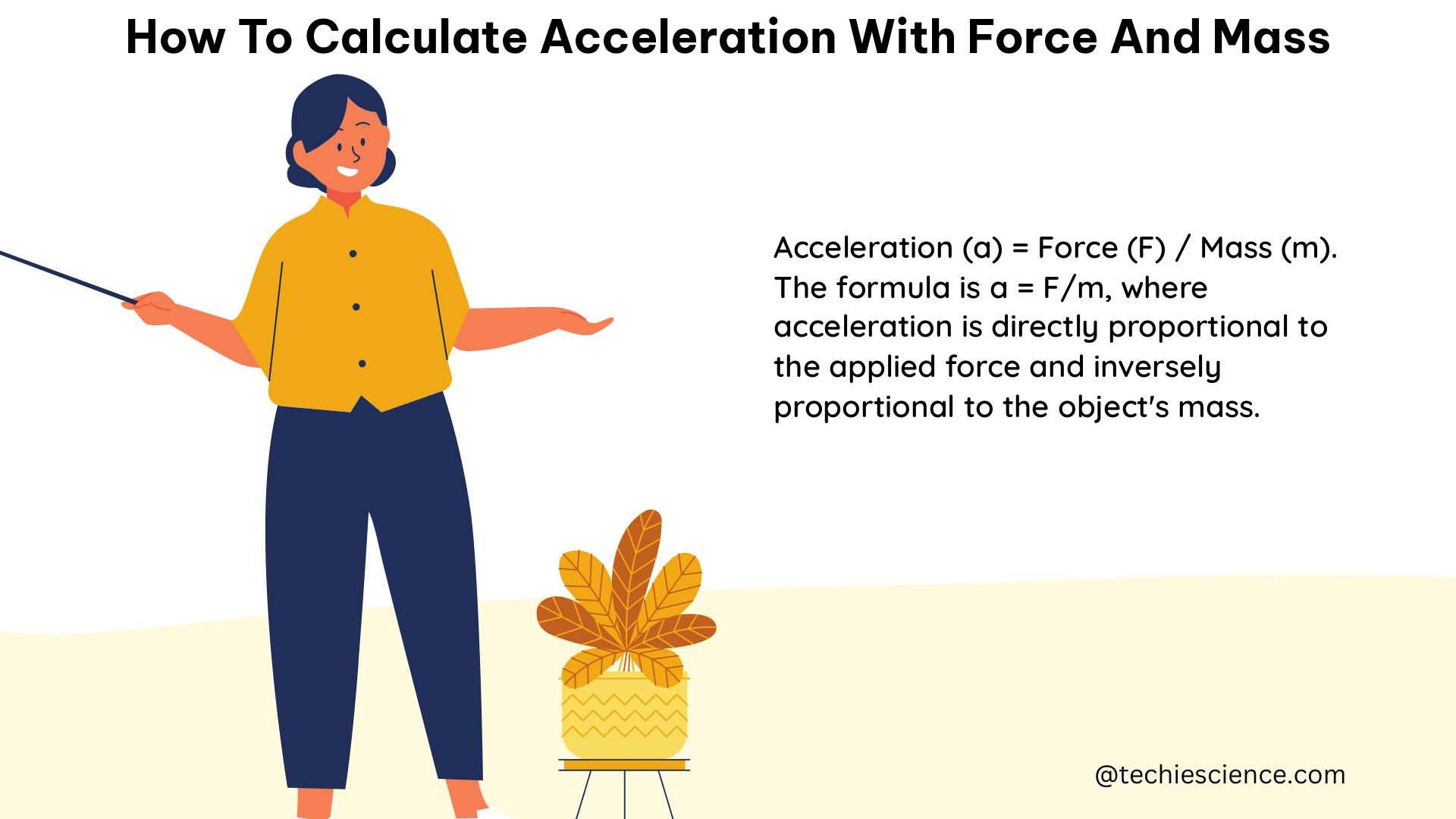
According to Newton’s second law of motion, the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. This relationship can be expressed mathematically as:

F = m × a

Where:
– F is the net force acting on the object, measured in Newtons (N)
– m is the mass of the object, measured in kilograms (kg)
– a is the acceleration of the object, measured in meters per second squared (m/s²)

Rearranging this equation, we can solve for the acceleration:

a = F / m

This formula is the key to calculating acceleration using force and mass.

Step-by-Step Guide to Calculating Acceleration

To calculate the acceleration of an object using force and mass, follow these steps:

1. Measure the Mass:

2. Determine the mass of the object in kilograms (kg). This can be done using a scale or a mass measurement device.

3. Measure the Force:

4. Measure the net force acting on the object in Newtons (N). This can be done using a force sensor or by applying a known force and measuring its magnitude.

5. Calculate Acceleration:

6. Plug the values of force (F) and mass (m) into the formula:
   a = F / m
7. Perform the calculation to find the acceleration (a) in meters per second squared (m/s²).

Example Calculations

Let’s go through some examples to illustrate the process:

1. Example 1:
2. Mass (m) = 10 kg
3. Force (F) = 50 N

4. Acceleration (a) = F / m = 50 N / 10 kg = 5 m/s²

5. Example 2:

6. Mass (m) = 3 kg
7. Force (F) = 12 N

8. Acceleration (a) = F / m = 12 N / 3 kg = 4 m/s²

9. Example 3:

10. Mass (m) = 16,000 kg
11. Force (F) = 200,000 N
12. Acceleration (a) = F / m = 200,000 N / 16,000 kg = 12.5 m/s²

Theoretical Explanation: Newton’s Second Law of Motion

The relationship between force, mass, and acceleration is governed by Newton’s second law of motion, which states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass.

Mathematically, this can be expressed as:

F = m × a

Where:
– F is the net force acting on the object, measured in Newtons (N)
– m is the mass of the object, measured in kilograms (kg)
– a is the acceleration of the object, measured in meters per second squared (m/s²)

This formula is the foundation for calculating acceleration using force and mass. By rearranging the equation, we can solve for the acceleration:

a = F / m

Key Concepts and Definitions

1. Mass: The total amount of matter in an object, measured in kilograms (kg).
2. Force: The push or pull acting on an object, measured in Newtons (N).
3. Acceleration: The rate of change in velocity, measured in meters per second squared (m/s²).

Figures and Data Points

To further illustrate the concept, let’s consider the following data points:

• Mass: 10 kg, 3 kg, 16,000 kg
• Force: 50 N, 12 N, 200,000 N
• Acceleration: 5 m/s², 4 m/s², 12.5 m/s²

These data points can be used to calculate the acceleration using the formula a = F / m.

Reference Links

1. Acceleration Calculator Using Force and Mass
2. Calculating Acceleration with Force and Mass
3. Force, Mass, and Acceleration: Newton’s Second Law

By following the step-by-step guide, understanding the theoretical foundations, and applying the provided examples and data points, you should now have a comprehensive understanding of how to calculate acceleration using force and mass. Remember to practice with various scenarios to solidify your knowledge and become proficient in this essential physics skill.