Acceleration is a fundamental concept in physics that describes the rate of change in an object’s velocity over time. To calculate acceleration using speed and time, you can employ a simple formula that provides a direct and accurate way to determine this important kinematic quantity. This comprehensive guide will delve into the details of this formula, providing a thorough understanding of the underlying principles, practical applications, and advanced techniques for finding acceleration.
Understanding the Acceleration Formula
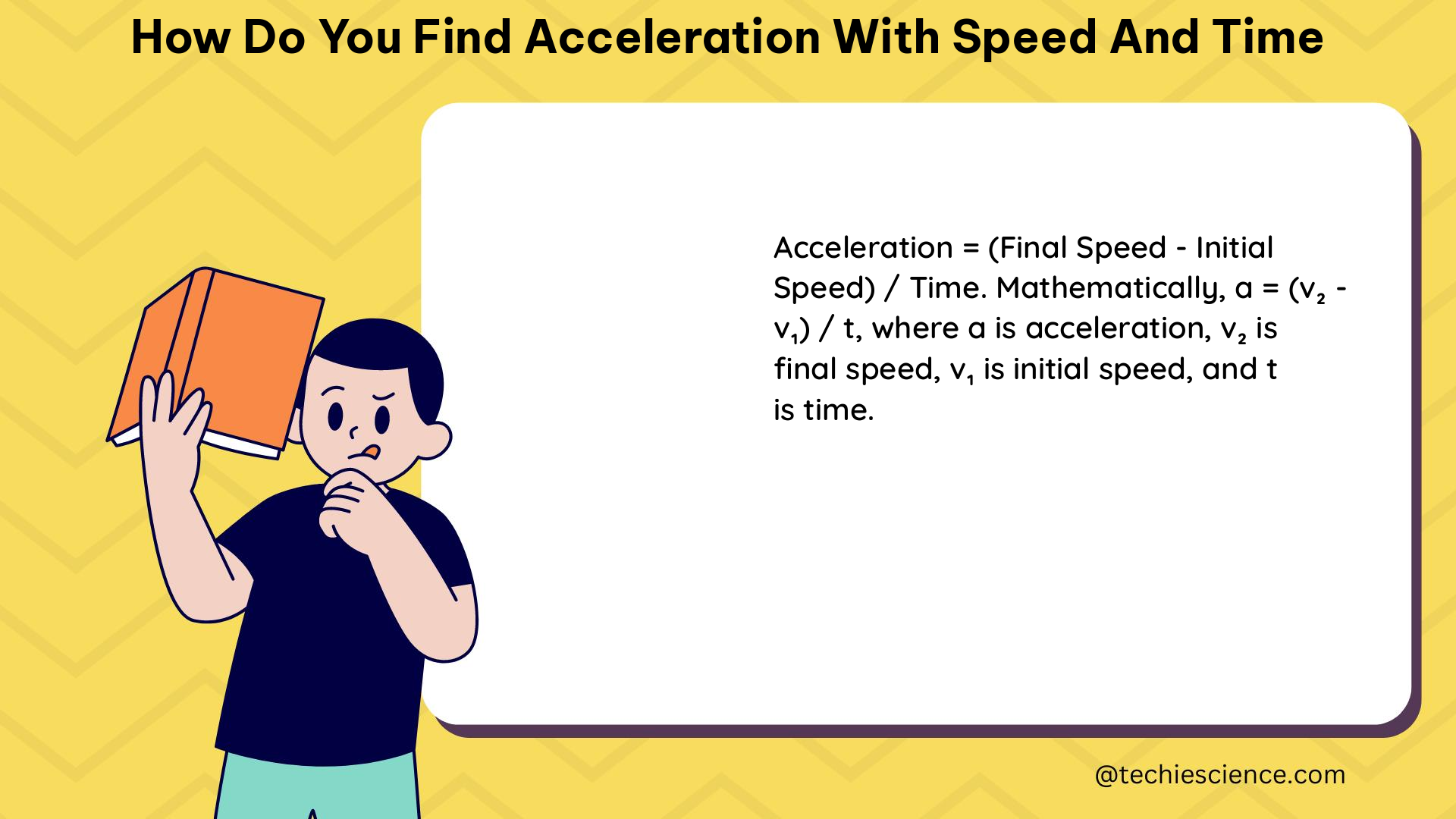
The formula for calculating acceleration using speed and time is:
a = Δv / Δt
Where:
– a is the acceleration (in units of m/s² or ft/s²)
– Δv is the change in velocity (in units of m/s or ft/s)
– Δt is the change in time (in units of seconds)
This formula is derived directly from the definition of acceleration, which is the rate of change in velocity over time. By rearranging the terms, you can also solve for the change in velocity or the change in time, if the other two variables are known.
Calculating the Change in Velocity (Δv)
The change in velocity, Δv, is the difference between the final velocity (v₁) and the initial velocity (v₀):
Δv = v₁ - v₀
For example, if an object starts with an initial velocity of 10 m/s and accelerates to a final velocity of 30 m/s, the change in velocity would be:
Δv = 30 m/s - 10 m/s = 20 m/s
Calculating the Change in Time (Δt)
The change in time, Δt, is the difference between the final time (t₁) and the initial time (t₀):
Δt = t₁ - t₀
For instance, if an object accelerates from 10 m/s to 30 m/s over a period of 5 seconds, the change in time would be:
Δt = 5 s - 0 s = 5 s
Applying the Acceleration Formula
Once you have the values for the change in velocity and the change in time, you can plug them into the acceleration formula to find the acceleration:
a = Δv / Δt
Let’s consider an example:
Suppose a car is traveling at an initial velocity of 20 m/s and then accelerates to a final velocity of 40 m/s over a period of 5 seconds. To find the acceleration, we would calculate:
Δv = 40 m/s - 20 m/s = 20 m/s
Δt = 5 s - 0 s = 5 s
a = Δv / Δt = 20 m/s / 5 s = 4 m/s²
Therefore, the acceleration of the car is 4 m/s².
Acceleration Units
Acceleration is typically measured in units of meters per second squared (m/s²) or feet per second squared (ft/s²). However, it can also be expressed in other units, such as miles per hour per second (mph/s), depending on the context and the units used for velocity and time.
It’s important to ensure that the units for velocity and time are consistent when using the acceleration formula. For example, if the velocity is in meters per second and the time is in seconds, the resulting acceleration will be in meters per second squared.
Theoretical Explanation of Acceleration
Acceleration is a vector quantity, meaning it has both magnitude (the numerical value) and direction. It describes the rate of change in an object’s velocity over time, which can be in the form of a change in speed, a change in direction, or a combination of both.
Acceleration is a fundamental concept in physics and is closely related to the study of motion, forces, and energy. It is a crucial factor in understanding and analyzing the behavior of objects, from everyday situations to complex physical systems.
The acceleration formula, a = Δv / Δt, is a direct application of the definition of acceleration. It provides a straightforward way to calculate the acceleration of an object given the change in its velocity and the time over which that change occurred.
Advanced Techniques and Applications
While the basic acceleration formula is widely used, there are more advanced techniques and applications that can be explored:
Acceleration Graphs
Plotting acceleration as a function of time can provide valuable insights into the motion of an object. These graphs can be used to analyze patterns, identify changes in acceleration, and even determine the forces acting on the object.
Acceleration in Two or Three Dimensions
The acceleration formula can be extended to handle motion in two or three dimensions, where the velocity and acceleration vectors have both magnitude and direction. This is particularly important in the study of projectile motion, circular motion, and other complex physical systems.
Numerical Problems and Simulations
Solving numerical problems and running simulations can help reinforce the understanding of acceleration and its applications. These exercises can involve calculating acceleration, predicting motion, and analyzing the effects of various factors on the acceleration of an object.
Acceleration in Rotational Motion
The concept of acceleration can also be applied to rotational motion, where the object is undergoing angular acceleration. In this case, the formula for angular acceleration is similar to the linear acceleration formula, but it involves angular quantities instead of linear ones.
Acceleration and Newton’s Laws of Motion
Acceleration is closely linked to Newton’s laws of motion, which describe the relationship between an object’s motion and the forces acting upon it. Understanding the connection between acceleration and these fundamental laws can provide a deeper insight into the underlying principles of physics.
Conclusion
Mastering the ability to find acceleration using speed and time is a crucial skill for any physics student or enthusiast. By understanding the acceleration formula, its derivation, and the various techniques and applications, you can develop a comprehensive understanding of this important kinematic concept.
Remember, the key to success in this area is to practice solving a wide range of problems, from simple to complex, and to continuously expand your knowledge by exploring the theoretical foundations and advanced topics related to acceleration.
References
- College Transitions: How to Calculate Acceleration – 3 Formulas You Must Know (https://www.collegetransitions.com/blog/how-to-calculate-acceleration-formula/)
- Dummies.com: How to Calculate Acceleration (https://www.dummies.com/article/academics-the-arts/science/physics/how-to-calculate-acceleration-174302/)
- Khan Academy: Acceleration (https://www.khanacademy.org/science/physics/one-dimensional-motion/acceleration-tutorial/v/acceleration)

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.