The Definitive Guide to Understanding Mercury Density (Hg Density)

Mercury, also known by its chemical symbol Hg, is a unique and fascinating element with a wide range of applications in various industries. One of the most fundamental properties of mercury is its density, which is a crucial factor in understanding its behavior and characteristics. In this comprehensive guide, we will delve into the intricacies of mercury density, exploring its measurement, calculation, and practical implications.

Understanding the Absolute Density of Mercury

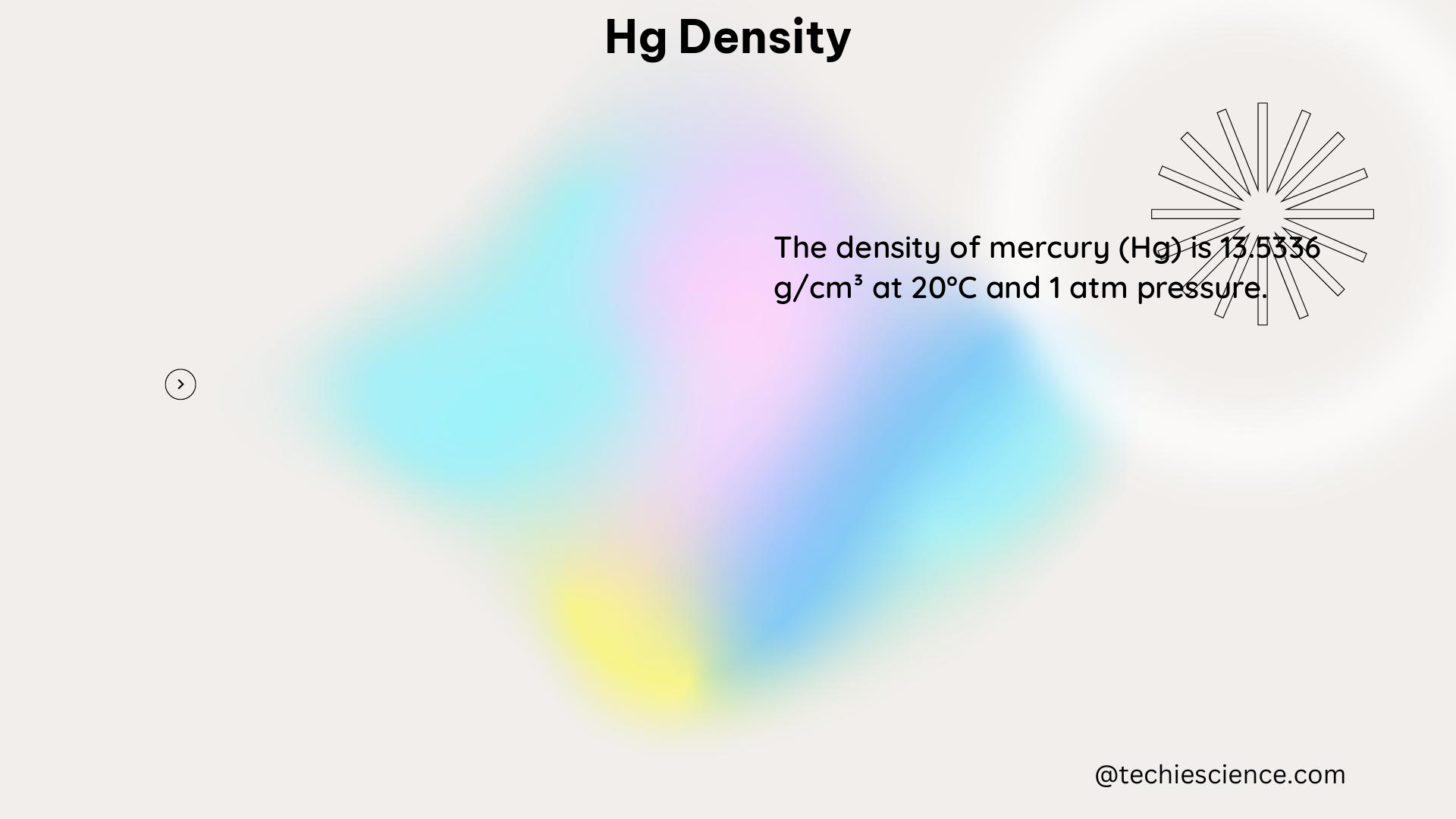
The absolute density of mercury is a fixed value that is approximately 13.534 g/cm^3 at 20°C and standard atmospheric pressure. This value is a physical property of mercury and remains constant under normal conditions. However, it is important to note that the density of a substance can change with variations in temperature and pressure.

The formula to calculate the density of a substance is:

Density = Mass / Volume

For mercury, the density can be expressed as:

Density of Mercury = 13.534 g/cm^3 at 20°C

This value is a crucial parameter in many applications, as it affects the behavior and handling of mercury in various contexts.

Factors Affecting Mercury Density

While the absolute density of mercury is a fixed value, there are several factors that can influence the density of mercury under different conditions:

1. Temperature: The density of mercury is known to decrease as the temperature increases. This is due to the thermal expansion of the material, which causes the volume to expand, resulting in a lower density. The relationship between temperature and density can be expressed using the following formula:

Density of Mercury = 13.534 g/cm^3 - (0.0182 g/cm^3 × (T - 20°C))

where T is the temperature in degrees Celsius.

1. Pressure: The density of mercury also varies with changes in pressure. As the pressure increases, the volume of the mercury decreases, leading to an increase in density. This relationship can be described by the following equation:

Density of Mercury = 13.534 g/cm^3 + (0.000156 g/cm^3 × (P - 1 atm))

where P is the pressure in atmospheres (atm).

1. Impurities: The presence of impurities in mercury can also affect its density. Trace amounts of other elements or compounds can alter the overall density of the mercury sample.

By understanding these factors, researchers and professionals can accurately predict and account for the density of mercury in various applications, ensuring optimal performance and safety.

Measuring Mercury Density

There are several methods and techniques used to measure the density of mercury, each with its own advantages and limitations. Here are some of the common approaches:

1. Direct Measurement: The most straightforward method is to directly measure the mass and volume of a mercury sample. This can be done by weighing a known volume of mercury and then calculating the density using the formula:

Density = Mass / Volume

This method is simple and accurate but requires precise measurement of the mercury sample.

1. Pycnometry: Pycnometry is a technique that uses a calibrated glass vessel, called a pycnometer, to determine the density of a liquid or solid. The pycnometer is filled with the mercury sample, and the mass of the filled pycnometer is measured. The density is then calculated based on the known volume of the pycnometer and the mass of the mercury.

2. Mercury Intrusion Porosimetry: This technique is used to measure the pore size and volume of solid materials by forcing mercury into the pores under high pressure. By measuring the amount of mercury intruded into the pores, the density of the solid material can be determined.

3. Ideal Gas Law: The density of mercury vapor can be determined using the ideal gas law, which relates the pressure, volume, and temperature of a gas to its molar mass. By measuring the density of mercury vapor at a known temperature and pressure, the molar mass of the vapor can be calculated, which is related to the density of the liquid mercury.

Each of these methods has its own advantages and limitations, and the choice of technique depends on the specific requirements of the application and the available equipment.

Applications of Mercury Density

The density of mercury is a crucial parameter in a wide range of applications, including:

1. Industrial Applications: Mercury’s high density makes it useful in various industrial applications, such as in the construction of barometers, thermometers, and other scientific instruments.

2. Dental Amalgams: Mercury is a key component in dental amalgams, which are used to fill cavities in teeth. The density of mercury plays a role in the durability and performance of these dental fillings.

3. Electrical and Electronic Devices: Mercury’s high density and electrical conductivity make it useful in the production of electrical switches, relays, and other electronic components.

4. Research and Development: The density of mercury is an important factor in various research and development activities, such as in the study of fluid dynamics, phase transitions, and material properties.

5. Environmental Monitoring: The density of mercury is a crucial parameter in environmental monitoring and remediation efforts, as it affects the transport and fate of mercury in the environment.

By understanding the intricacies of mercury density, researchers, engineers, and professionals can optimize the performance, safety, and efficiency of these applications.

Numerical Examples and Calculations

To further illustrate the practical applications of mercury density, let’s consider a few numerical examples:

1. Calculating the Mass of Mercury in a Barometer:
2. Assume a barometer has a mercury column with a height of 76 cm and a cross-sectional area of 1 cm^2.

3. Using the density of mercury at 20°C (13.534 g/cm^3), the mass of the mercury in the barometer can be calculated as:
   Mass of Mercury = Density × Volume
Mass of Mercury = 13.534 g/cm^3 × (76 cm × 1 cm^2)
Mass of Mercury = 1,028.58 g

4. Determining the Density of Mercury at Different Temperatures:

5. Suppose we want to calculate the density of mercury at 30°C.

6. Using the formula for the relationship between temperature and density:
   Density of Mercury = 13.534 g/cm^3 - (0.0182 g/cm^3 × (30°C - 20°C))
Density of Mercury = 13.534 g/cm^3 - (0.0182 g/cm^3 × 10°C)
Density of Mercury = 13.534 g/cm^3 - 0.182 g/cm^3
Density of Mercury = 13.352 g/cm^3

7. Calculating the Density of Mercury Vapor using the Ideal Gas Law:

8. Assume the temperature of the mercury vapor is 100°C and the pressure is 1 atm.
9. Using the ideal gas law:
   PV = nRT
Density = m/V = (n × M) / V
10. Where:
    • P = Pressure (1 atm)
    • V = Volume (1 L)
    • n = Moles of mercury vapor
    • R = Ideal gas constant (0.082057 L·atm/mol·K)
    • T = Temperature (373.15 K)
    • M = Molar mass of mercury (200.59 g/mol)
11. Solving for the density:
    Density = (n × 200.59 g/mol) / 1 L
Density = (1 atm × 1 L) / (0.082057 L·atm/mol·K × 373.15 K) × 200.59 g/mol
Density = 15.34 g/L

These examples demonstrate how the density of mercury can be calculated and applied in various scenarios, highlighting the importance of understanding this fundamental property.

Conclusion

In this comprehensive guide, we have explored the intricacies of mercury density, covering its absolute value, the factors that influence it, and the various methods used to measure it. We have also discussed the practical applications of mercury density in a wide range of industries and provided numerical examples to illustrate the calculations involved.

By understanding the density of mercury, researchers, engineers, and professionals can optimize the performance, safety, and efficiency of their applications, whether it’s in the construction of scientific instruments, the development of dental amalgams, or the monitoring of environmental mercury levels.

As you continue your exploration of mercury and its properties, remember to always prioritize safety and follow all relevant regulations and guidelines when working with this unique and fascinating element.

References

1. Quizlet. (n.d.). ISSA Final Exam. Retrieved from https://quizlet.com/727616773/issa-final-exam-flash-cards/
2. GEW UV. (n.d.). Understanding and Quantifying Energy Emitted from UV Curing Sources. Retrieved from https://www.gewuv.com/understanding-and-quantifying-energy-emitted-from-uv-curing-sources/
3. Anton Paar. (n.d.). Mercury Intrusion Porosimetry: Basics – Measuring Pores in Solids. Retrieved from https://wiki.anton-paar.com/us-en/mercury-intrusion-porosimetry-basics-measuring-pores-in-solids/
4. LibreTexts. (n.d.). Combining the Gas Laws: The Ideal Gas Equation and the General Gas Equation. Retrieved from https://chem.libretexts.org/Bookshelves/General_Chemistry/Map:General_Chemistry(Petrucci_et_al.)/06:_Gases/6.3:_Combining_the_Gas_Laws:_The_Ideal_Gas_Equation_and_the_General_Gas_Equation