Gravitational Force Formula 2: A Comprehensive Guide

The gravitational force formula 2, also known as Newton’s Law of Universal Gravitation, is a fundamental equation in physics that allows us to calculate the gravitational force between any two objects, given their masses and the distance between them. This formula is a crucial tool for understanding the behavior of celestial bodies, designing spacecraft trajectories, and analyzing the dynamics of various physical systems.

Understanding the Gravitational Force Formula 2

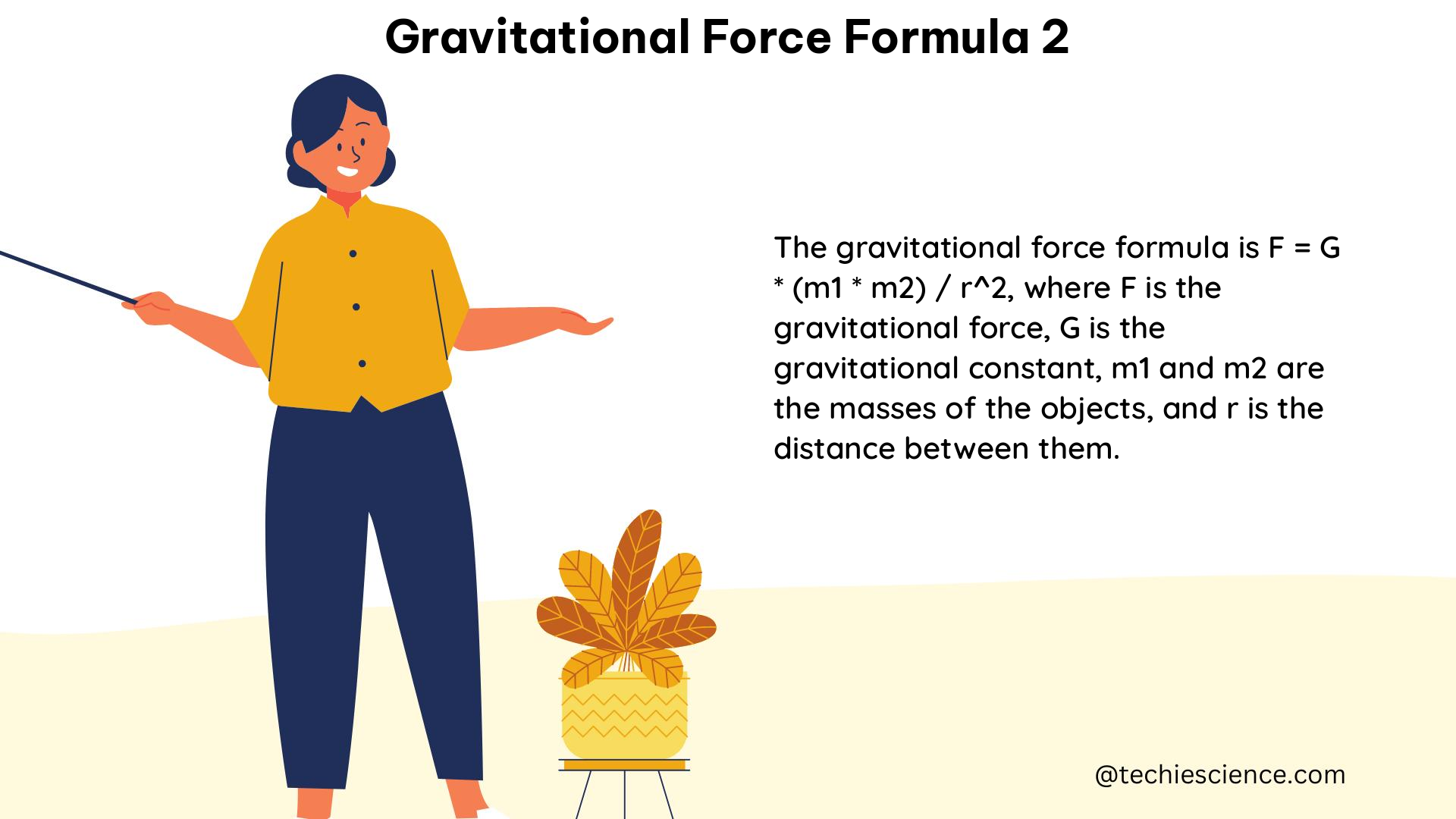
The gravitational force formula 2 is expressed as:

F = G * (m1 * m2) / r^2

Where:
– F is the gravitational force between the two objects, measured in Newtons (N)
– G is the gravitational constant, approximately equal to 6.674 × 10^-11 N⋅m^2/kg^2
– m1 and m2 are the masses of the two objects, measured in kilograms (kg)
– r is the distance between the centers of the two objects, measured in meters (m)

This formula is based on the principle of proportionality, where the gravitational force is directly proportional to the product of the masses of the two objects and inversely proportional to the square of the distance between them.

Gravitational Constant (G)

The gravitational constant, G, is a fundamental physical constant that relates the strength of the gravitational force between two objects to their masses and the distance between them. The value of G has been measured experimentally and is approximately equal to 6.674 × 10^-11 N⋅m^2/kg^2.

The gravitational constant is a universal constant, meaning that it has the same value regardless of the specific objects or the location in the universe. This constant is a crucial parameter in the gravitational force formula 2 and is used in various applications, such as calculating the gravitational force between celestial bodies, designing spacecraft trajectories, and analyzing the dynamics of physical systems.

Gravitational Force Calculation

To calculate the gravitational force between two objects using the gravitational force formula 2, you need to know the masses of the objects and the distance between them. Once you have these values, you can plug them into the formula and solve for the gravitational force.

For example, let’s calculate the gravitational force between the Earth and the Moon:

• Mass of the Earth (m1) = 5.972 × 10^24 kg
• Mass of the Moon (m2) = 7.342 × 10^22 kg
• Distance between the Earth and the Moon (r) = 384,400,000 m

Plugging these values into the formula:

F = G * (m1 * m2) / r^2
F = (6.674 × 10^-11 N⋅m^2/kg^2) * ((5.972 × 10^24 kg) * (7.342 × 10^22 kg)) / (384,400,000 m)^2
F = 1.98 × 10^20 N

Therefore, the gravitational force between the Earth and the Moon is approximately 1.98 × 10^20 Newtons.

Applications of the Gravitational Force Formula 2

The gravitational force formula 2 has a wide range of applications in various fields of physics and astronomy. Here are some of the key applications:

Celestial Mechanics

The gravitational force formula 2 is fundamental to the study of celestial mechanics, which is the branch of physics that deals with the motion of celestial bodies, such as planets, moons, and stars. This formula is used to calculate the gravitational forces between these bodies and to predict their orbits and trajectories.

For example, the gravitational force formula 2 is used to calculate the orbits of planets around the Sun, the motion of moons around planets, and the trajectories of comets and asteroids.

Spacecraft Dynamics

The gravitational force formula 2 is also crucial in the design and analysis of spacecraft trajectories. When a spacecraft is launched into space, it is subject to the gravitational forces of the Earth, the Moon, and other celestial bodies. By using the gravitational force formula 2, engineers can accurately predict the spacecraft’s trajectory and make adjustments to ensure a successful mission.

Gravitational Lensing

Gravitational lensing is a phenomenon where the gravitational field of a massive object, such as a galaxy or a cluster of galaxies, bends the path of light from a distant object. The gravitational force formula 2 is used to calculate the strength of the gravitational field and the degree of bending of the light, which can be used to study the properties of the lensing object and the distant object.

Gravitational Waves

The gravitational force formula 2 is also relevant in the study of gravitational waves, which are ripples in the fabric of spacetime caused by the acceleration of massive objects. The formula is used to calculate the strength of the gravitational waves and to predict their effects on the motion of objects in the universe.

Geophysics and Geology

The gravitational force formula 2 is also used in geophysics and geology to study the Earth’s internal structure and composition. By measuring the gravitational field at different locations on the Earth’s surface, scientists can infer the distribution of mass within the Earth and use this information to study the Earth’s interior.

Limitations and Assumptions of the Gravitational Force Formula 2

While the gravitational force formula 2 is a powerful tool for understanding and predicting the behavior of physical systems, it is important to be aware of its limitations and the assumptions underlying its use.

1. Point Masses: The formula assumes that the objects involved are point masses, meaning that their mass is concentrated at a single point. This assumption may not hold true for large or irregularly shaped objects, where the distribution of mass within the object can affect the gravitational force.

2. Uniform Gravitational Field: The formula assumes that the gravitational field is uniform, meaning that the gravitational acceleration is constant throughout the region of interest. This assumption may not hold true in situations where the gravitational field is non-uniform, such as near the surface of a planet or in the presence of strong gravitational gradients.

3. Weak Gravitational Fields: The formula is valid for weak gravitational fields, where the effects of general relativity can be neglected. In situations with strong gravitational fields, such as near black holes or in the early universe, the effects of general relativity must be taken into account.

4. Constant Gravitational Constant: The formula assumes that the gravitational constant, G, is a constant and does not vary with time or location. However, some theories of gravity, such as modified gravity theories, suggest that the gravitational constant may not be truly constant.

Despite these limitations, the gravitational force formula 2 remains a fundamental and widely used tool in physics and astronomy, providing a reliable and accurate description of the gravitational force between objects in many practical situations.

Conclusion

The gravitational force formula 2, also known as Newton’s Law of Universal Gravitation, is a powerful tool for understanding and predicting the behavior of physical systems in a wide range of applications, from celestial mechanics to spacecraft dynamics and geophysics. By understanding the formula, its underlying assumptions, and its limitations, physicists and engineers can use it to gain valuable insights into the nature of the universe and to design and analyze complex systems with greater accuracy and precision.

References:

1. Newton’s Law of Universal Gravitation
2. Gravitational Constant
3. Gravitational Lensing
4. Gravitational Waves
5. Geophysics and Gravity