The gravitational acceleration formula, also known as the formula for calculating the acceleration due to gravity, is a fundamental equation in physics that describes the relationship between the mass of an object, its radius, and the acceleration experienced by objects near its surface. This formula, derived from Newton’s laws of motion and universal gravitation, is a powerful tool for understanding the behavior of celestial bodies, designing spacecraft, and analyzing the motion of objects in various gravitational fields.
Understanding the Gravitational Acceleration Formula
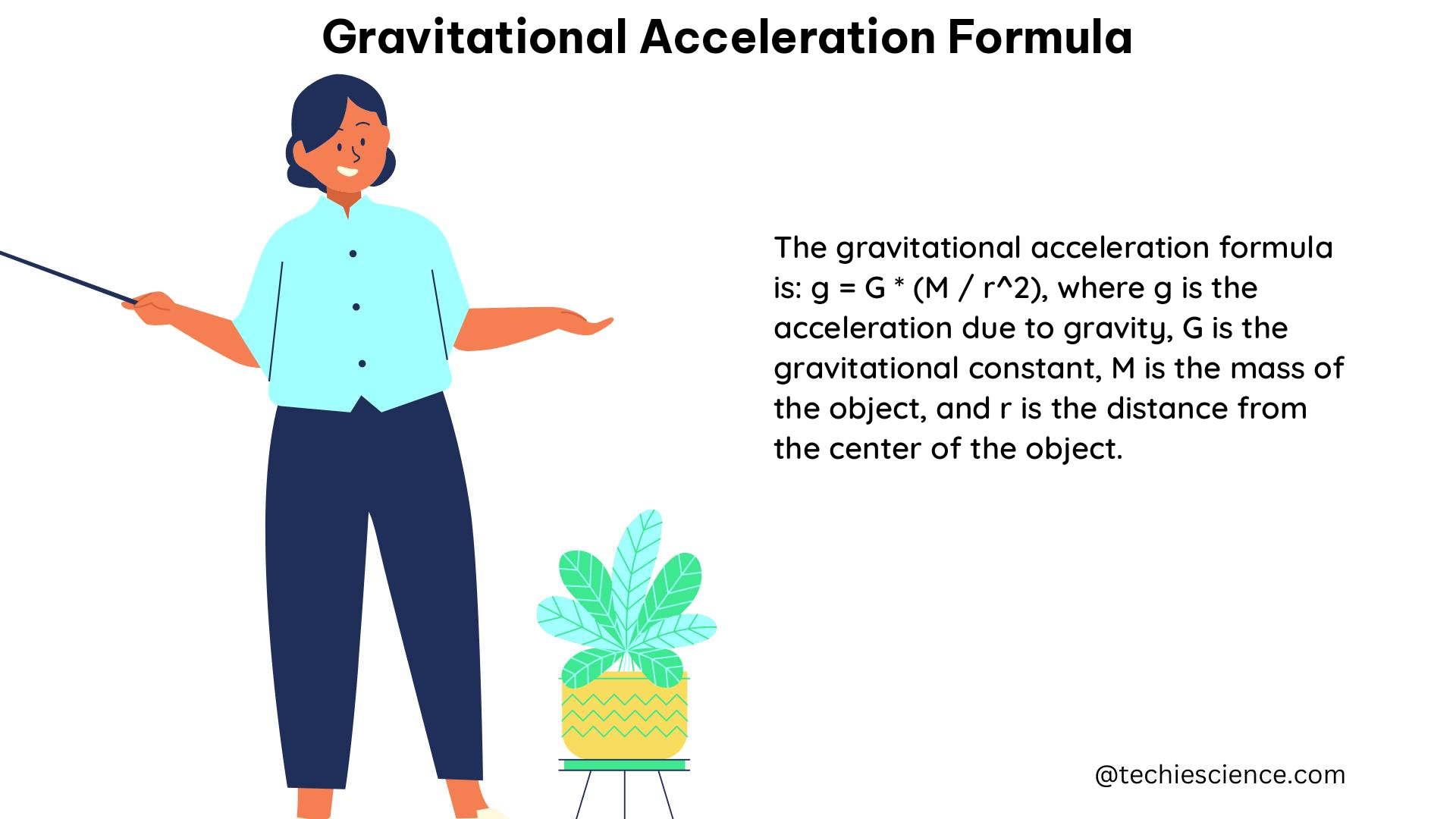
The formula for calculating the acceleration due to gravity is:
g = G * M / R^2
where:
gis the acceleration due to gravity, measured in meters per second squared (m/s²)Gis the gravitational constant, which has a value of approximately 6.67 × 10^-11 N⋅m²/kg²Mis the mass of the object, measured in kilograms (kg)Ris the radius of the object, measured in meters (m)
This formula is based on Newton’s Second Law of Motion and Newton’s Law of Universal Gravitation. It is used to calculate the acceleration due to gravity on the surface of a planet, moon, or other celestial body, based on its mass and radius.
Calculating Gravitational Acceleration on Earth
On the surface of the Earth, the acceleration due to gravity is approximately 9.8 m/s². This value can be calculated using the formula and the known values for the mass and radius of the Earth:
g = (6.67 × 10^-11 N⋅m²/kg²) * (5.979 × 10^24 kg) / (6.376 × 10^6 m)^2
g ≈ 9.8 m/s²
The mass of the Earth is approximately 5.979 × 10^24 kg, and the average radius of the Earth is approximately 6.376 × 10^6 m. Plugging these values into the formula, we can calculate the acceleration due to gravity on the surface of the Earth.
Gravitational Acceleration on Other Celestial Bodies
The gravitational acceleration formula can also be used to calculate the acceleration due to gravity on the surface of other celestial bodies, such as the Moon or Mars.
For example, on the surface of the Moon, the acceleration due to gravity is approximately 1.6 m/s². This value can be calculated using the formula and the known values for the mass and radius of the Moon:
g = (6.67 × 10^-11 N⋅m²/kg²) * (7.35 × 10^22 kg) / (1.74 × 10^6 m)^2
g ≈ 1.6 m/s²
The mass of the Moon is approximately 7.35 × 10^22 kg, and the average radius of the Moon is approximately 1.74 × 10^6 m.
Similarly, on the surface of Mars, the acceleration due to gravity is approximately 3.7 m/s². This value can be calculated using the formula and the known values for the mass and radius of Mars:
g = (6.67 × 10^-11 N⋅m²/kg²) * (6.39 × 10^23 kg) / (3.389 × 10^6 m)^2
g ≈ 3.7 m/s²
The mass of Mars is approximately 6.39 × 10^23 kg, and the average radius of Mars is approximately 3.389 × 10^6 m.
Gravitational Acceleration at Different Altitudes
The gravitational acceleration formula can also be used to calculate the acceleration due to gravity at different altitudes above the surface of a planet or celestial body. As the distance from the center of the object increases, the acceleration due to gravity decreases.
For example, on Mars, the acceleration due to gravity at an altitude of 160,000 meters above the surface is approximately 3.4 m/s². This value can be calculated using the formula and the known values for the mass of Mars and the distance from the center of the planet:
g = (6.67 × 10^-11 N⋅m²/kg²) * (6.39 × 10^23 kg) / (3.389 × 10^6 m + 160,000 m)^2
g ≈ 3.4 m/s²
In this case, the radius of Mars has been increased by 160,000 meters to account for the altitude above the surface.
Factors Affecting Gravitational Acceleration
The value of the acceleration due to gravity, g, is influenced by several factors:
- Mass of the Object: The greater the mass of the object, the stronger the gravitational pull and the higher the acceleration due to gravity.
- Radius of the Object: The acceleration due to gravity is inversely proportional to the square of the radius of the object. As the radius increases, the acceleration due to gravity decreases.
- Altitude: As the distance from the center of the object increases, the acceleration due to gravity decreases, as shown in the example for Mars.
- Latitude: The acceleration due to gravity can also vary slightly due to the Earth’s rotation and the resulting centrifugal force, which is greater at the equator than at the poles.
- Elevation: The acceleration due to gravity decreases slightly as the elevation above sea level increases, due to the decrease in the Earth’s mass below the measurement point.
Practical Applications of the Gravitational Acceleration Formula
The gravitational acceleration formula has numerous practical applications in various fields, including:
- Spacecraft Design: The formula is used to calculate the gravitational forces acting on spacecraft during launch, orbit, and landing, which is crucial for ensuring the safety and stability of the spacecraft.
- Satellite Orbits: The formula is used to determine the appropriate orbits for satellites around planets and other celestial bodies, ensuring they remain in stable and predictable paths.
- Geophysics: The formula is used to study the Earth’s internal structure and composition by measuring variations in the acceleration due to gravity at different locations on the planet.
- Pendulum Clocks: The formula is used to calculate the period of a pendulum clock, which is dependent on the acceleration due to gravity at the location of the clock.
- Ballistics: The formula is used to calculate the trajectory of projectiles, such as artillery shells or ballistic missiles, which are affected by the acceleration due to gravity.
Conclusion
The gravitational acceleration formula is a fundamental equation in physics that describes the relationship between the mass of an object, its radius, and the acceleration experienced by objects near its surface. This formula has numerous practical applications in various fields, from spacecraft design to geophysics, and is an essential tool for understanding the behavior of celestial bodies and the motion of objects in various gravitational fields.
References
- Acceleration Due to Gravity | Definition, Formula & Examples – Lesson, https://study.com/academy/lesson/calculating-acceleration-due-to-gravity-formula-lesson-quiz.html
- Gravity Lab – Physics, https://physics.weber.edu/palen/clearinghouse/homeworks/Gravity_Lab.html
- Gravity Equation | Formula, Calculation & Example – Lesson, https://study.com/academy/lesson/isaac-newtons-formula-for-the-force-of-gravity-definition-example.html
- The Value of g – The Physics Classroom, https://www.physicsclassroom.com/class/circles/Lesson-3/The-Value-of-g
- Calculating Acceleration Due To Gravity on a Plane, https://www.intmath.com/blog/mathematics/calculating-acceleration-due-to-gravity-on-a-plane-12517

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.