Finding Acceleration from Friction: A Comprehensive Guide

Summary

Determining the acceleration of an object in the presence of friction involves understanding the net force acting on the object, the frictional force, and the application of Newton’s second law of motion. This comprehensive guide will provide you with the necessary theoretical knowledge, formulas, examples, and problem-solving techniques to master the concept of finding acceleration from friction.

Understanding Net Force and Frictional Force

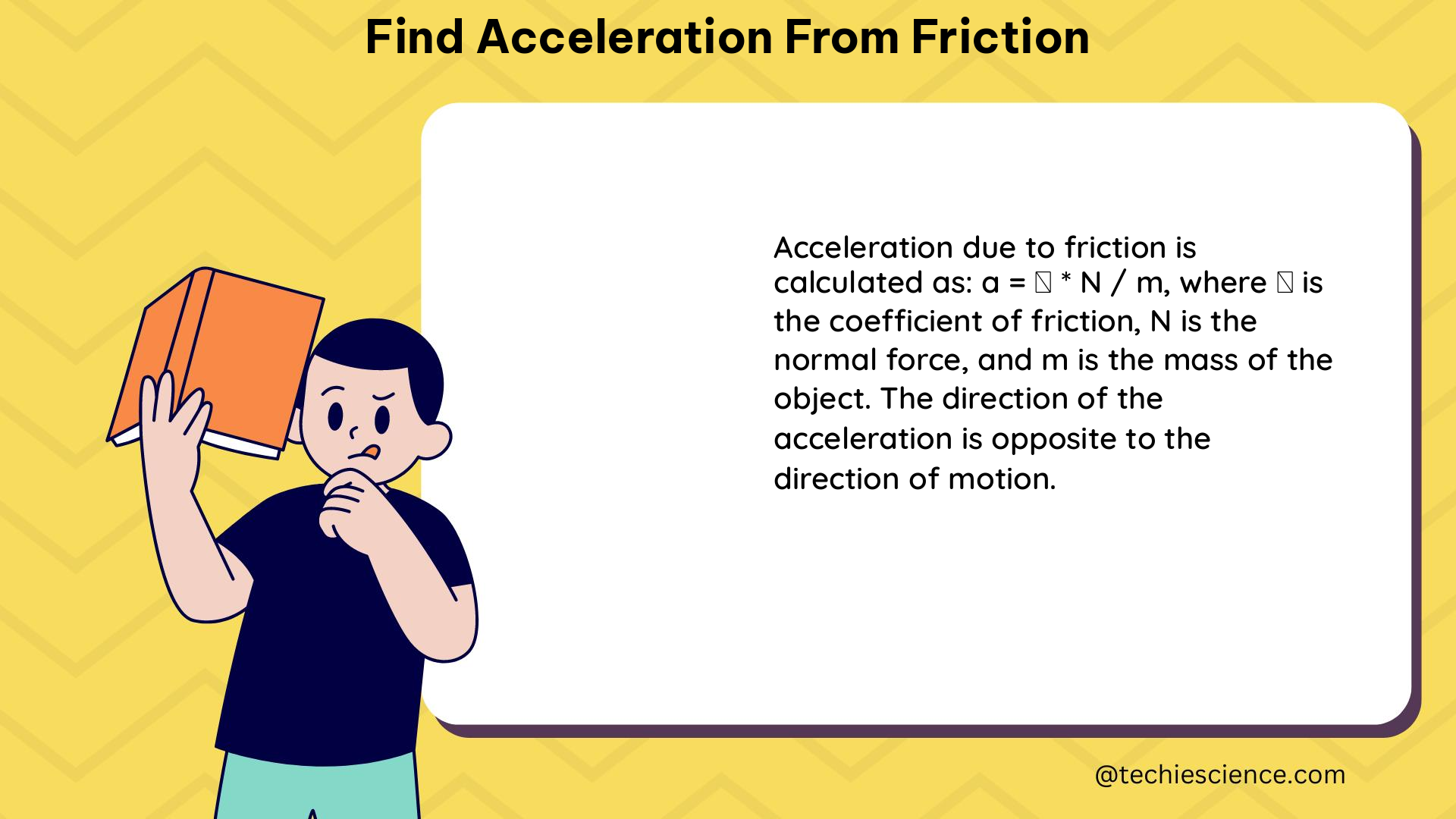
The net force acting on an object is the vector sum of all the forces acting on it. In the presence of friction, the net force is given by the equation:

Fnet = Fapp - Ffrict

where Fapp is the applied force, and Ffrict is the frictional force. The frictional force is given by the equation:

Ffrict = μN

where μ is the coefficient of friction, and N is the normal force. The normal force is equal to the weight of the object, which is given by the equation:

w = mg

where m is the mass of the object, and g is the acceleration due to gravity.

Applying Newton’s Second Law

Once we have the net force, we can find the acceleration using Newton’s second law, which states that the acceleration of an object is proportional to the net force acting on it, and inversely proportional to its mass. Mathematically, this is expressed as:

a = Fnet/m

where a is the acceleration, Fnet is the net force, and m is the mass of the object.

Example Calculation

Let’s consider an example to illustrate the process of finding acceleration from friction.

Suppose we have a box of mass 10 kg on a horizontal surface, and we apply a force of 20 N to the box. The coefficient of friction between the box and the surface is 0.2. We want to find the acceleration of the box.

1. Calculate the frictional force:
   Ffrict = μN
Ffrict = 0.2 x 10 x 9.8
Ffrict = 19.6 N

2. Calculate the net force:
   Fnet = Fapp - Ffrict
Fnet = 20 - 19.6
Fnet = 0.4 N

3. Calculate the acceleration using Newton’s second law:
   a = Fnet/m
a = 0.4/10
a = 0.04 m/s^2

Therefore, the acceleration of the box is 0.04 m/s^2.

Theorem and Formulas

1. Net Force Equation:
   Fnet = Fapp - Ffrict

2. Frictional Force Equation:
   Ffrict = μN

3. Normal Force Equation:
   w = mg

4. Newton’s Second Law:
   a = Fnet/m

Physics Examples

1. Example 1: A 5 kg block is pushed across a horizontal surface with a force of 10 N. The coefficient of friction between the block and the surface is 0.3. Find the acceleration of the block.

2. Example 2: A 2 kg object is sliding on a rough surface with a constant velocity of 3 m/s. The coefficient of friction between the object and the surface is 0.2. Find the force required to maintain the constant velocity.

3. Example 3: A 50 kg crate is being pulled across a floor with a force of 100 N. The coefficient of friction between the crate and the floor is 0.4. Find the acceleration of the crate.

Physics Numerical Problems

1. Problem 1: A 10 kg box is pushed across a horizontal surface with a force of 20 N. The coefficient of friction between the box and the surface is 0.3. Find the acceleration of the box.

2. Problem 2: A 15 kg object is sliding on a surface with a constant velocity of 2 m/s. The coefficient of friction between the object and the surface is 0.1. Find the force required to maintain the constant velocity.

3. Problem 3: A 25 kg crate is being pulled across a floor with a force of 80 N. The coefficient of friction between the crate and the floor is 0.2. Find the acceleration of the crate.

Figures and Data Points

Table: Coefficient of Friction Values for Common Materials

Material Combination	Coefficient of Friction (μ)
Steel on Steel	0.78
Aluminum on Steel	0.61
Copper on Steel	0.53
Rubber on Concrete	0.68
Wood on Wood	0.25 – 0.50
Ice on Ice	0.10

Conclusion

In this comprehensive guide, we have explored the concept of finding acceleration from friction. By understanding the net force, frictional force, and the application of Newton’s second law, you now have the necessary tools to solve a wide range of problems involving the acceleration of objects in the presence of friction. Remember to practice the examples and numerical problems provided to solidify your understanding and develop your problem-solving skills.

References

1. Foundations of Physics, Chapter 13: Friction and Accelerated Motion
   https://kpu.pressbooks.pub/foundationsofphysics/chapter/chapter-13-friction-and-accelerated-motion/
2. Calculate Acceleration with Friction – Sciencing
   https://sciencing.com/calculate-acceleration-friction-6245754.html
3. Finding Acceleration – The Physics Classroom
   https://www.physicsclassroom.com/class/newtlaws/Lesson-3/Finding-Acceleration