The Final Velocity Formula: A Comprehensive Guide for Physics Students

The final velocity formula, v = u + at, is a fundamental concept in the field of kinematics, which deals with the motion of objects. This formula allows us to calculate the final velocity of an object, given its initial velocity, acceleration, and the time taken. In this comprehensive guide, we will delve into the intricacies of the final velocity formula, providing a wealth of technical details, physics formulas, examples, and numerical problems to help you master this essential concept.

Understanding the Final Velocity Formula

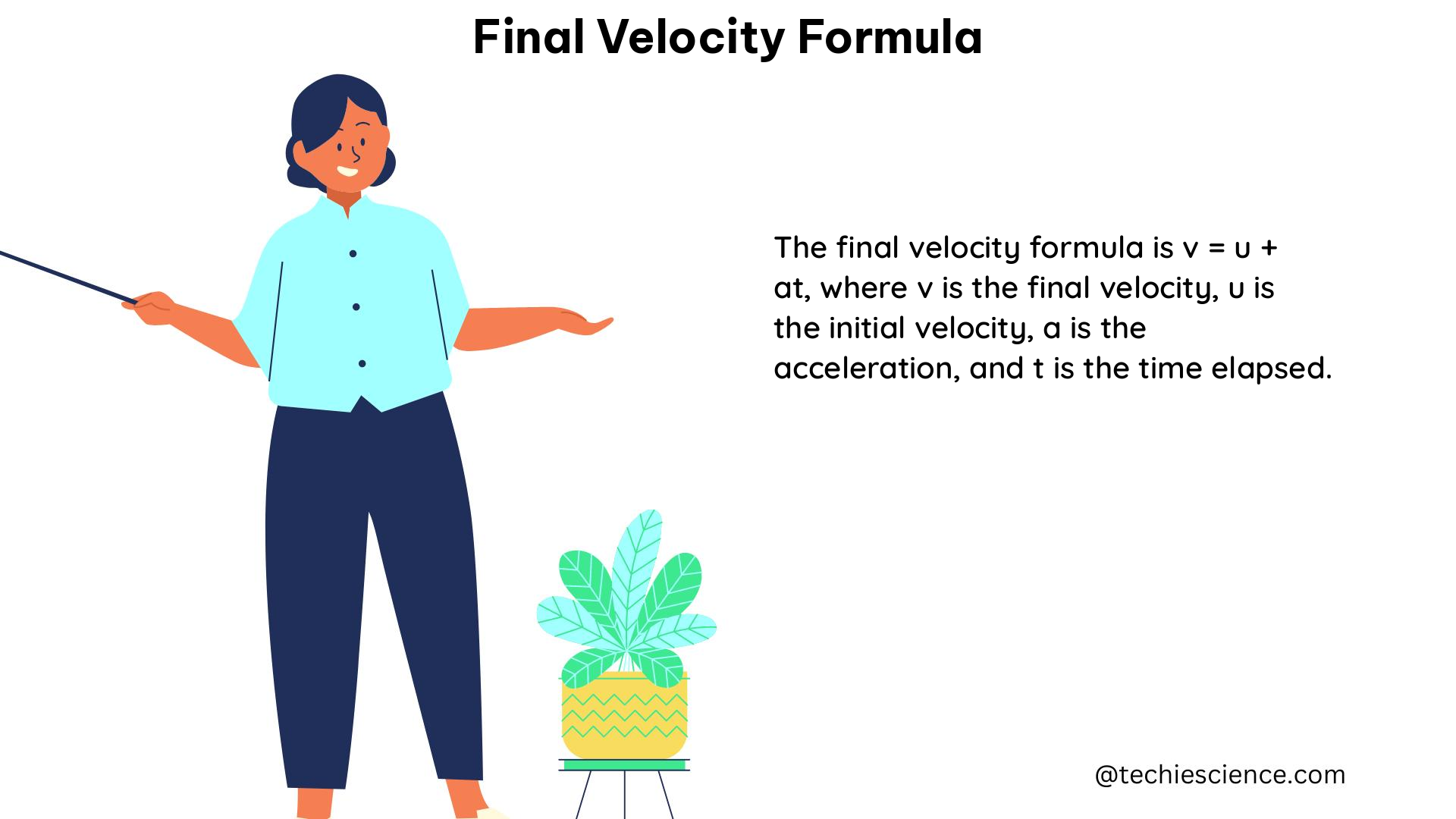
The final velocity formula is expressed as:

v = u + at

Where:
– v is the final velocity (in meters per second, m/s)
– u is the initial velocity (in meters per second, m/s)
– a is the acceleration (in meters per second squared, m/s²)
– t is the time taken (in seconds, s)

This formula is derived from the average velocity formula, which is given by:

vavg = (u + v) / 2

By rearranging this formula, we can obtain the final velocity formula:

v = 2 * vavg – u

Additionally, the final velocity formula can also be expressed in terms of displacement and time:

v = u + (Δs / t)

Where Δs is the displacement (in meters, m).

Theorem and Derivation

The final velocity formula is a direct consequence of the fundamental principles of kinematics. It can be derived using the following theorem:

Theorem: For an object moving with constant acceleration, the final velocity (v) is equal to the sum of the initial velocity (u) and the product of the acceleration (a) and the time taken (t).

Proof:
1. Let the initial position of the object be x₀ and the final position be x.
2. The displacement of the object is given by Δx = x – x₀.
3. The average velocity of the object is given by vavg = Δx / Δt.
4. Substituting the expression for displacement, we get:
vavg = (x – x₀) / Δt
5. Rearranging the terms, we get:
x – x₀ = vavg * Δt
6. Substituting the expression for average velocity, we get:
x – x₀ = (u + v) / 2 * Δt
7. Simplifying the equation, we get:
v = 2 * (x – x₀) / Δt – u
8. Recognizing that acceleration is defined as a = (v – u) / Δt, we can substitute this expression into the previous equation to obtain the final velocity formula:
v = u + a * Δt

Therefore, the final velocity formula, v = u + at, is a direct consequence of the fundamental principles of kinematics.

Physics Formulas and Relationships

The final velocity formula is closely related to other important physics formulas and concepts in kinematics. Here are some of the key relationships:

1. Displacement-Time Relationship:
   Δs = ut + 1/2 at²

2. Velocity-Time Relationship:
   v = u + at

3. Displacement-Velocity Relationship:
   Δs = (u + v) / 2 * t

4. Acceleration-Velocity Relationship:
   a = (v – u) / t

These formulas and relationships are essential for understanding and applying the final velocity formula in various physics problems.

Physics Examples

Let’s consider some examples to illustrate the application of the final velocity formula:

1. Example 1: A car is traveling at an initial velocity of 20 m/s. If the car accelerates at a rate of 3 m/s² for 5 seconds, what is the final velocity of the car?

Using the final velocity formula:
v = u + at
v = 20 m/s + (3 m/s² * 5 s)
v = 20 m/s + 15 m/s
v = 35 m/s

1. Example 2: A ball is dropped from a height of 80 meters. Assuming the acceleration due to gravity is 9.8 m/s², what is the final velocity of the ball just before it hits the ground?

Given:
– Initial velocity (u) = 0 m/s (the ball is dropped from rest)
– Acceleration (a) = -9.8 m/s² (acceleration due to gravity)
– Displacement (Δs) = -80 m (the ball is dropped from a height of 80 meters)

Using the final velocity formula:
v² = u² + 2aΔs
v² = 0 + 2 * (-9.8 m/s²) * (-80 m)
v² = 1568 m²/s²
v = √1568 m/s
v = 39.6 m/s

Physics Numerical Problems

1. Problem 1: A ball is thrown upward with an initial velocity of 20 m/s. Assuming the acceleration due to gravity is 9.8 m/s², find the maximum height reached by the ball and its final velocity when it reaches the maximum height.

Given:
– Initial velocity (u) = 20 m/s
– Acceleration (a) = -9.8 m/s² (acceleration due to gravity)

To find the maximum height:
v² = u² + 2aΔs
0 = (20 m/s)² + 2 * (-9.8 m/s²) * Δs
Δs = 20.4 m

To find the final velocity at the maximum height:
v = u + at
v = 20 m/s + (-9.8 m/s²) * (20.4 m / 20 m/s)
v = 0 m/s

1. Problem 2: A car is traveling at an initial velocity of 15 m/s. If the car accelerates at a rate of 2 m/s² for 10 seconds, find the final velocity of the car and the distance traveled during this time.

Given:
– Initial velocity (u) = 15 m/s
– Acceleration (a) = 2 m/s²
– Time (t) = 10 s

To find the final velocity:
v = u + at
v = 15 m/s + (2 m/s² * 10 s)
v = 15 m/s + 20 m/s
v = 35 m/s

To find the distance traveled:
Δs = ut + 1/2 at²
Δs = (15 m/s * 10 s) + 1/2 * (2 m/s² * 10 s²)
Δs = 150 m + 100 m
Δs = 250 m

Figures and Data Points

To further illustrate the concepts related to the final velocity formula, let’s consider the following figure and data points:

The graph shows the relationship between the final velocity (v) and the time (t) for an object moving with constant acceleration. The key data points are:

• Initial velocity (u) = 10 m/s
• Acceleration (a) = 2 m/s²
• Time (t) = 0 s to 10 s
• Final velocity (v) at t = 10 s = 30 m/s

Using the final velocity formula, we can calculate the final velocity at any given time:

v = u + at
v = 10 m/s + (2 m/s² * t)

For example, at t = 5 s, the final velocity would be:
v = 10 m/s + (2 m/s² * 5 s)
v = 10 m/s + 10 m/s
v = 20 m/s

This graph and the corresponding data points help visualize the relationship between the final velocity, initial velocity, acceleration, and time, further reinforcing the understanding of the final velocity formula.

Conclusion

The final velocity formula, v = u + at, is a fundamental concept in the field of kinematics and is essential for understanding and analyzing the motion of objects. In this comprehensive guide, we have explored the technical details, physics formulas, examples, and numerical problems related to the final velocity formula, providing you with a deep understanding of this essential concept.

By mastering the final velocity formula and its applications, you will be well-equipped to tackle a wide range of physics problems and gain a deeper appreciation for the principles of motion. Remember to practice the concepts presented in this guide, and don’t hesitate to refer back to it whenever you need a refresher on the final velocity formula.

References:

1. OpenStax, “University Physics Volume 1”, Ch. 4.3 Projectile Motion – University Physics Volume 1 | OpenStax, 2016.
2. GeeksforGeeks, “Velocity – Definition, Units, Formula, Examples”, 2022.
3. The Physics Classroom, “Kinematic Equations”, 2023.
4. Khan Academy, “Kinematic formulas and projectile motion”, 2023.
5. HyperPhysics, “Kinematic Equations”, 2023.